Angular momentum evolution can be predicted from cosmological initial conditions
With A. Pontzen & H. V. Peiris — arXiv:2012.02201

The origin of angular momentum
- Galaxies → origin of rotational support?
- Physics → origin of vector quantity irrotational field?
- Cosmology → origin of spin alignment signal?

New-Horizon simulation — Dubois+20

Tempel+13
Focus on closed systems: Lagrangian patch
Predict one value for \( \vec{L} \)
Focus on open systems: dark matter haloes
Predict \( p(\vec{L}) \)
Tidal Torque Theory
& semi-Analytical Models
$$ \vec{L} = a^2 \dot{D} \vec{L}_0 $$
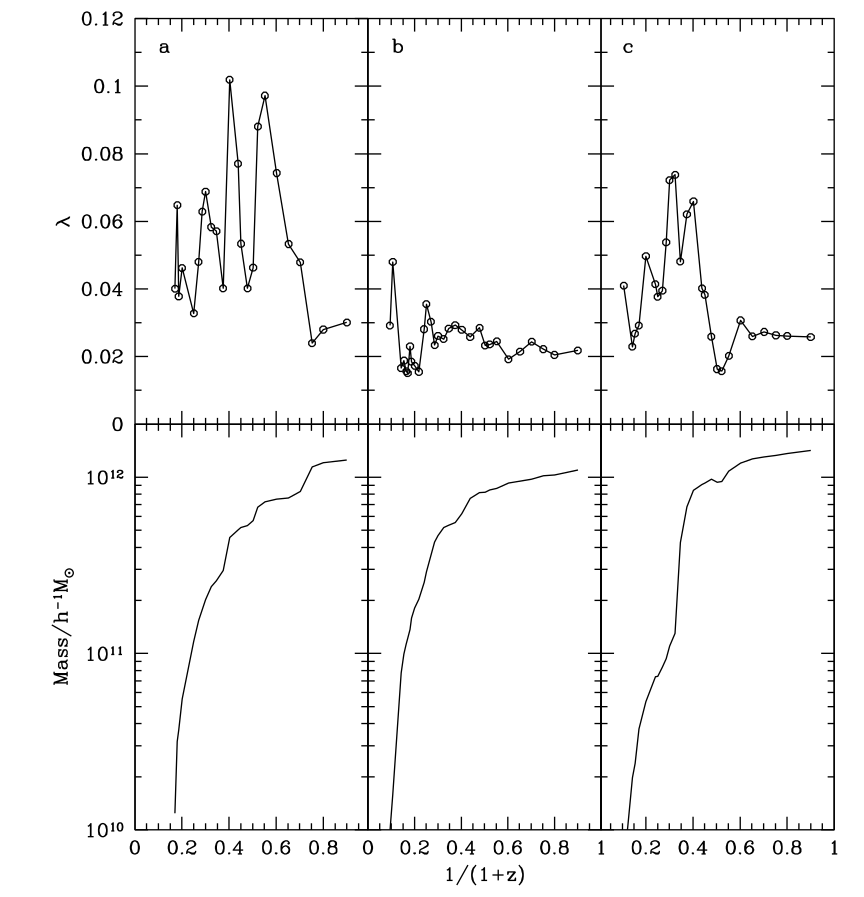
White 84, Codis+15
Vitvitska+02
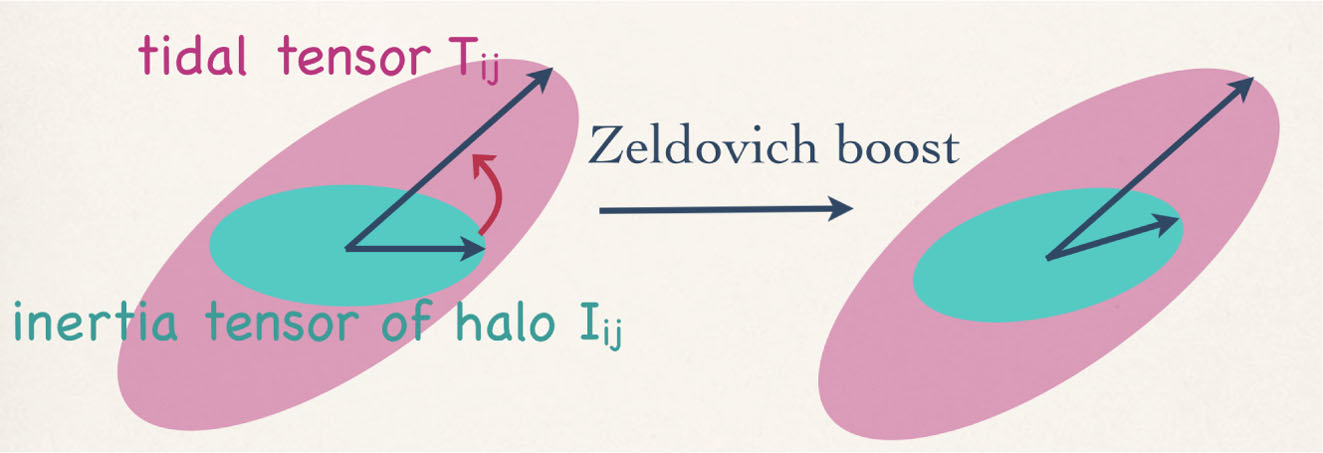
AM can be predicted
AM is stochastic/chaotic
TTT
Focus on closed systems: Lagrangian patch
Predict one value for \( \vec{L} \)
sAMs
Focus on open systems: dark matter haloes
Predict \( p(\vec{L}) \)
TTT & semi-analytical models
AM can be predicted from the initial conditions
AM is stochastic/chaotic
Is AM chaotic or not?
Is AM chaotic or not?
Not chaotic if for a given region,
$$ \vec{L}(t) = {\color{red}f(t)} \times \color{blue}\vec{L}_0,\phantom{(1+\epsilon),} $$
with \(\color{red} f\) indep. of \(\color{blue}\vec{L}_0\).
Is AM chaotic or not?
Not chaotic if for a given region,
$$ \vec{L}(t) = {\color{red}f(t)} \times \color{blue}\vec{L}_0\phantom{,}{(1+\epsilon)}, $$
with \(\color{red} f\) indep. of \(\color{blue}\vec{L}_0\).
Is AM chaotic or not?
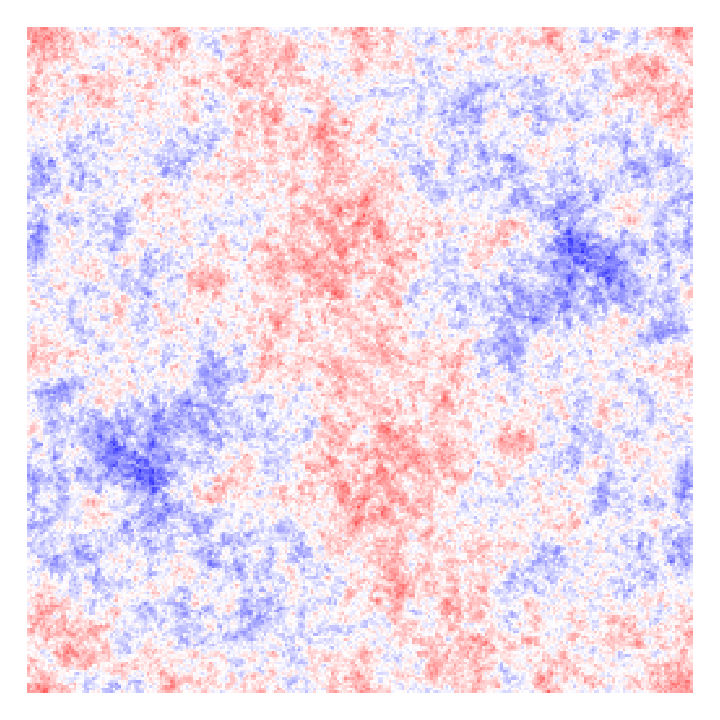
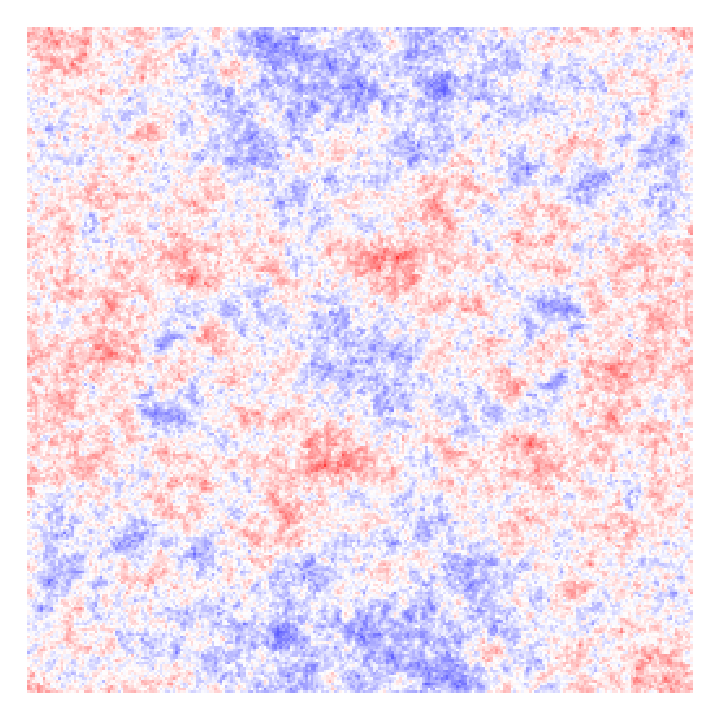
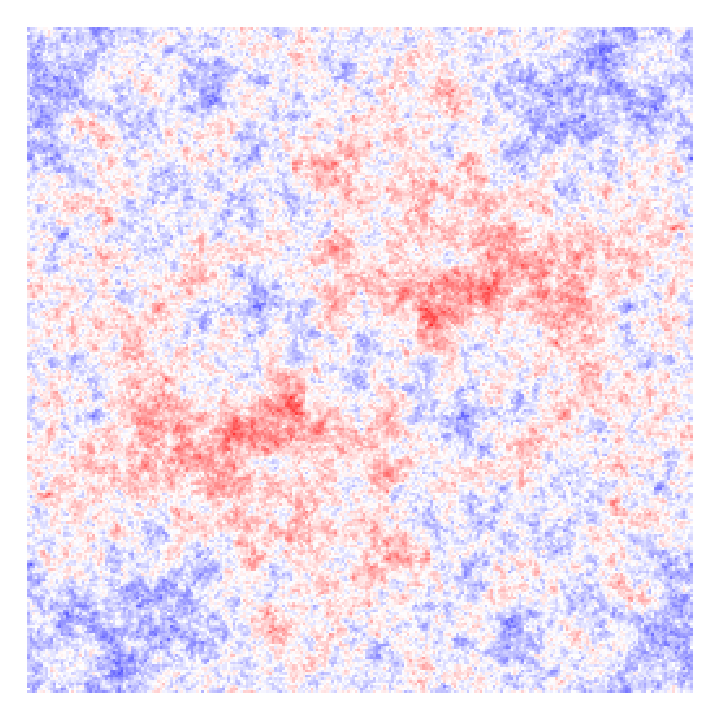
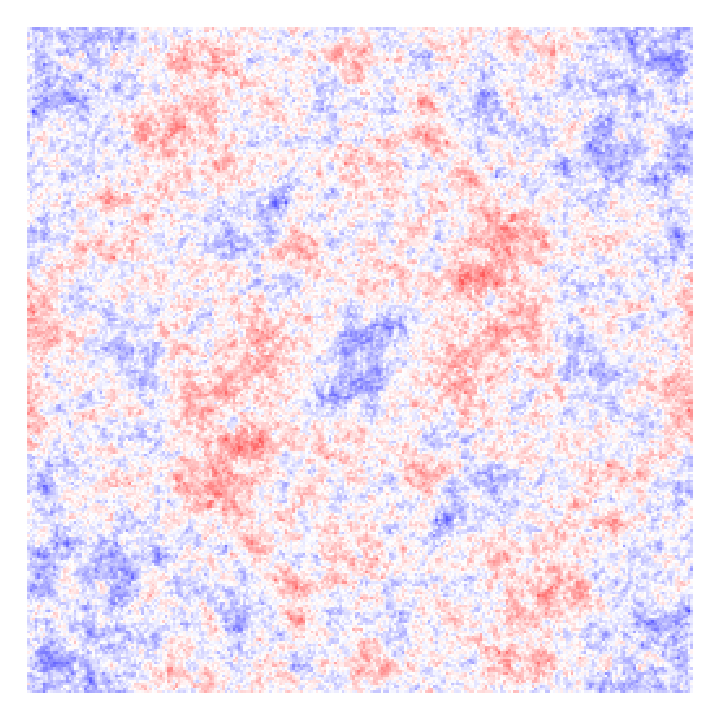

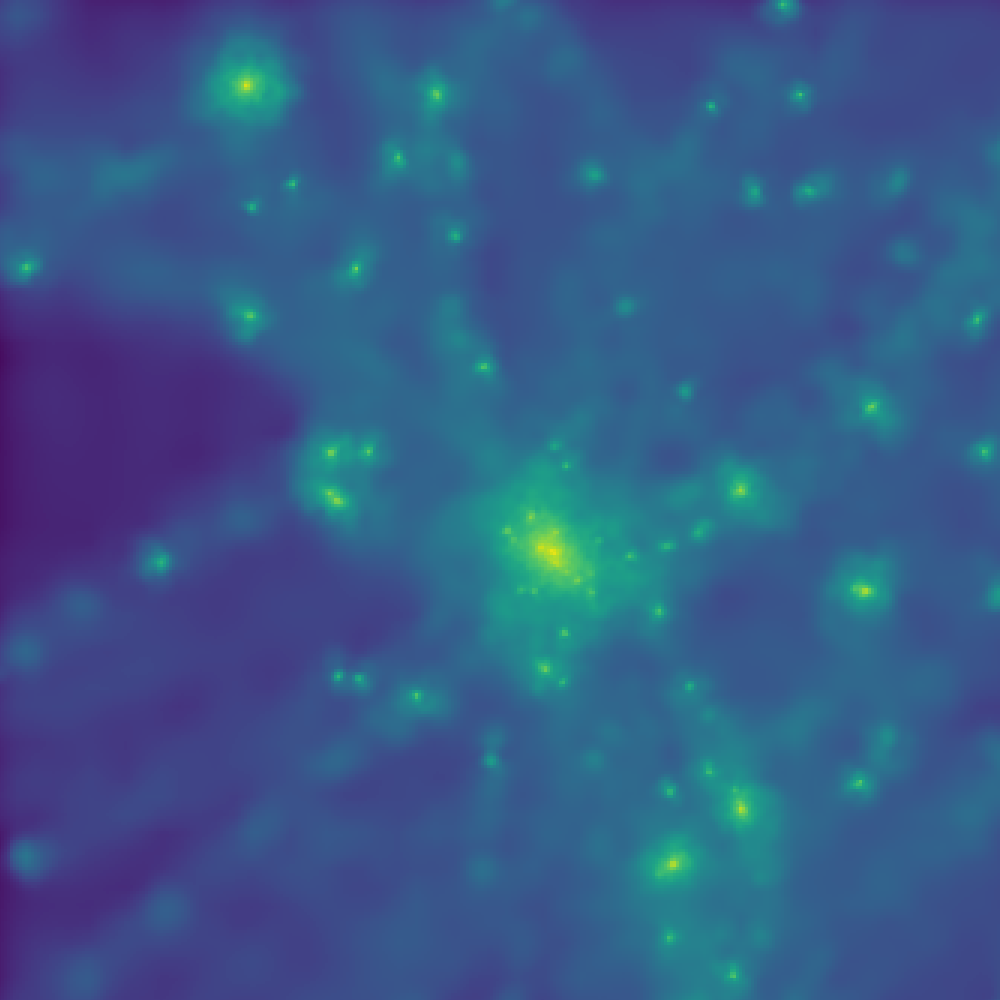
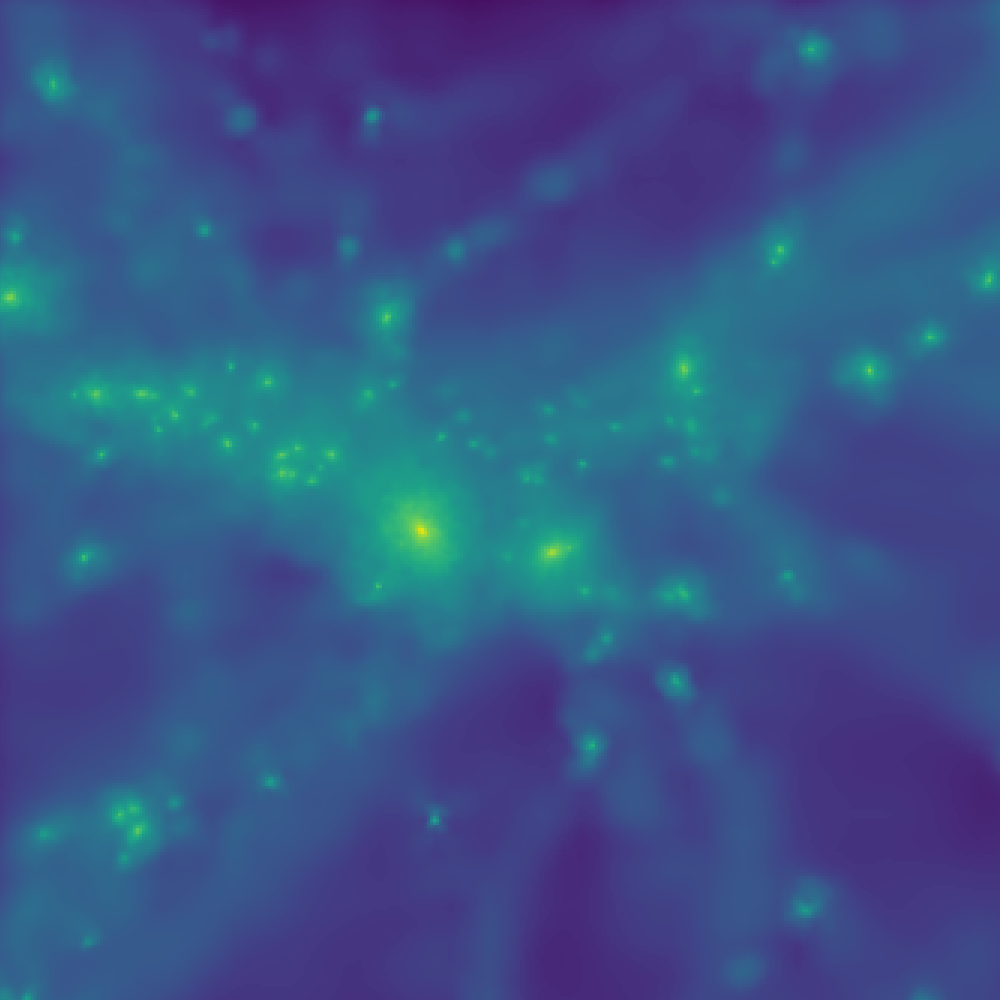
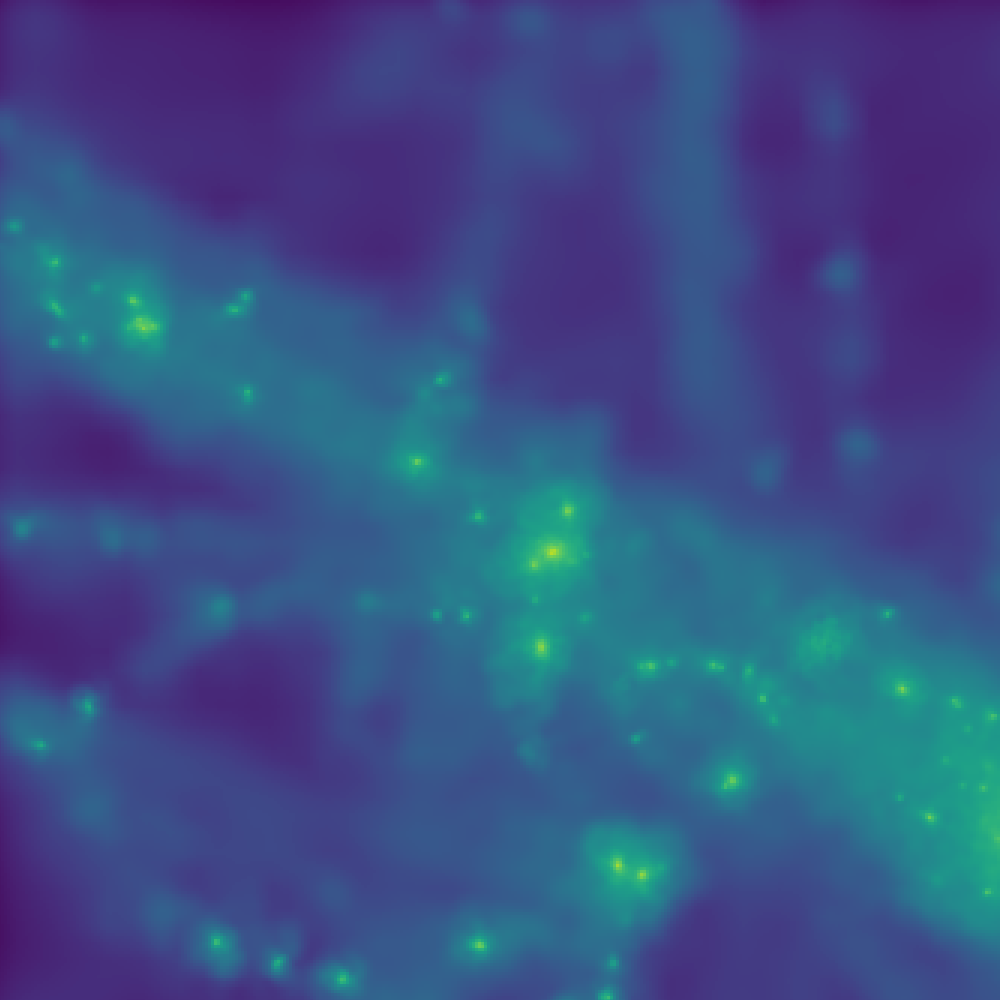
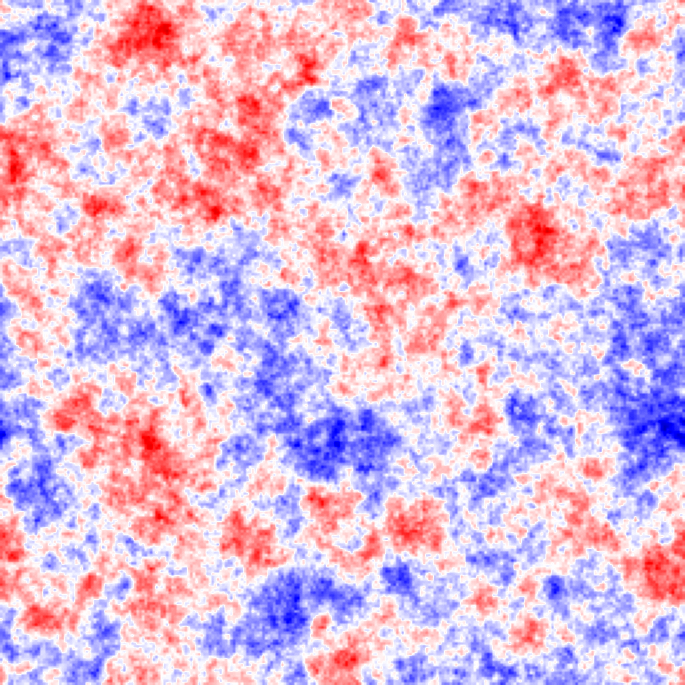
How to change \(\color{blue}\vec{L}_0\) all other things being equal?
\(\Lambda\)CDM gives you \(\neq\color{blue} \vec{L}_0\) … all other things different.
*Only an illustration, ICs do not correspond to the halos below.


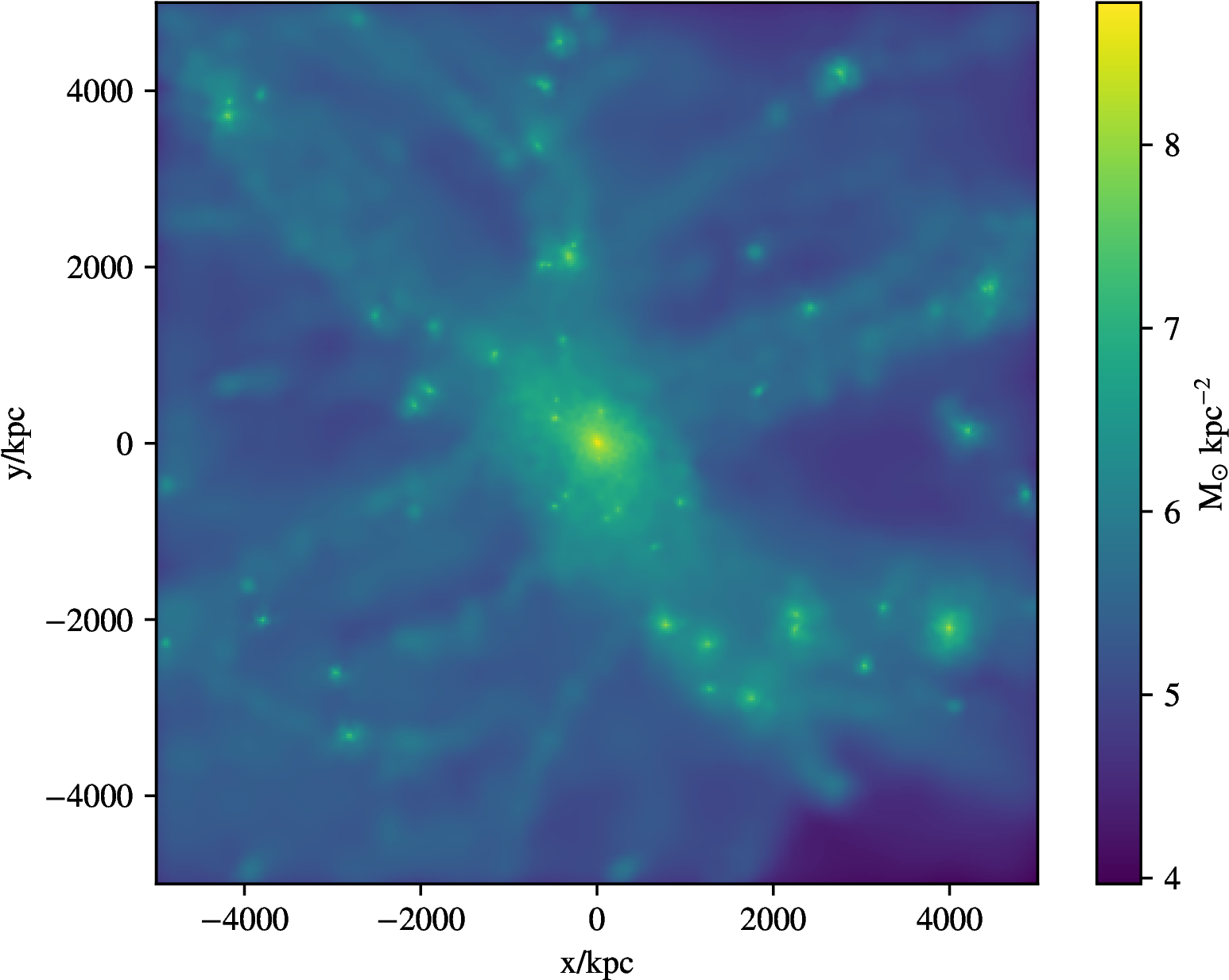
\(N\)-body numerical integration


\(N\)-body numerical integration


+
Original \(\vec{L}_0\)
Genetic modif.
\(N\)-body numerical integration
Contrast x50
Roth+15, Stopyra+20
Can compute
$$ P\big(\delta \big| \delta(x) = v\big) $$
or
$$ P\Big(\delta \Big| \sum_i \alpha_i \delta_i = v\Big) $$
or
$$ P\Big(\delta \Big| L_x = v \Big) $$
+ minimization of \(\Delta \chi^2\)
⇒ unique solution
= "genetically modified density field"




+
=
Original \(\vec{L}_0\)
Genetic modif.
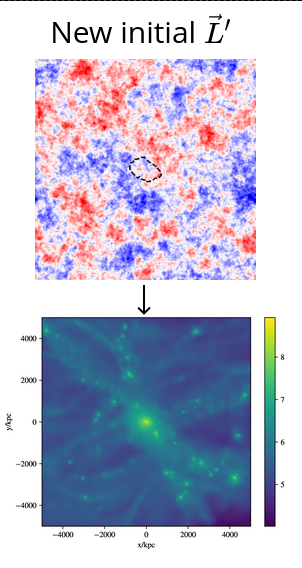
New initial \(\vec{L}_0'\)
\(N\)-body numerical integration
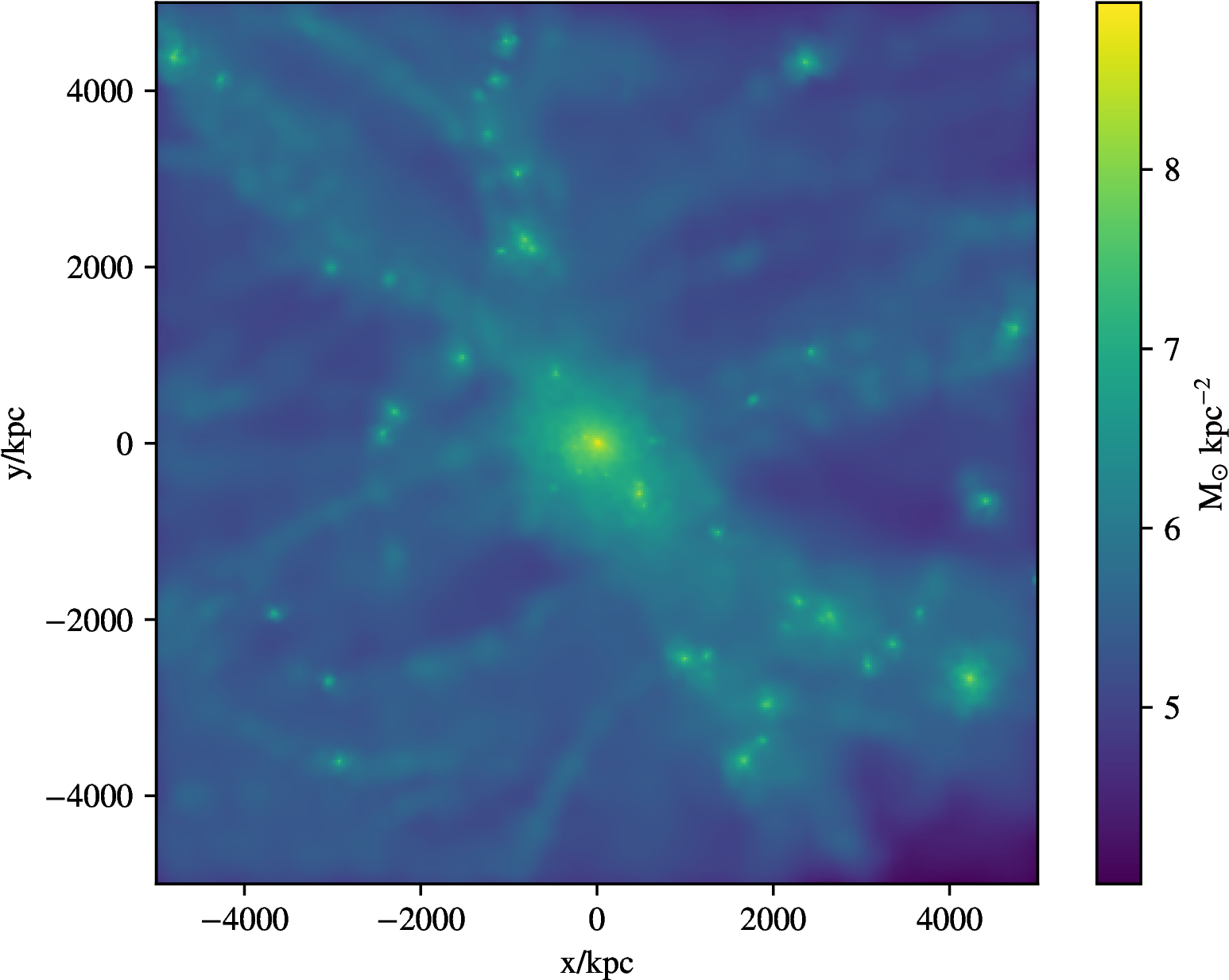
\(N\)-body numerical integration
Same region & environment
Different \(\color{blue} \vec{L}_0\)
Contrast x50
Roth+15, Stopyra+20



Original \(\vec{L}_0\)

New initial \(\vec{L}_0'\)







Original \(\vec{L}\)

New initial \(\vec{L}'\)







We can predict/control AM down to \(z = 0\).*
How does it compare to predictions from linear theory?
*AM of regions, not halos

Prediction from linear theory:
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad a^2\dot{D} \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}}.$$
Simulations with genetically modified initial conditions
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad f(t) \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}},$$
where \(\color{blue}f(t)\) is the measured growth rate of a given region in the ref. simu.
Comparison with tidal torque theory
Prediction from linear theory:
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad a^2\dot{D} \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}}.$$
Simulations with genetically modified initial conditions
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad f(t) \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}},$$where \(\color{blue}f(t)\) is the measured growth rate of a given region in the ref. simu.
Comparison with tidal torque theory




Comparison with tidal torque theory

Median of deviations
Prediction from linear theory:
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad a^2\dot{D} \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}}.$$
Simulations with genetically modified initial conditions
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad f(t) \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}},$$where \(\color{blue}f(t)\) is the measured growth rate of a given region in the ref. simu.
Comparison with tidal torque theory


Median of deviations
Scatter of deviations
Prediction from linear theory:
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad a^2\dot{D} \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}}.$$
Simulations with genetically modified initial conditions
$$ \vec{L}_\mathrm{pred.} = {\color{blue}\underbrace{\quad f(t) \quad}_{\color{blue}\mathrm{time}}} \times {\color{red}\underbrace{\quad\vec{L}_0\quad}_{\mathrm{space}}},$$where \(\color{blue}f(t)\) is the measured growth rate of a given region in the ref. simu.
Comparison with tidal torque theory


Median of deviations
Scatter of deviations
- Much more accurate pred. than linear theory
- Departure happen at later time
- The smaller the modif., the smaller the scatter
⇒ linear response
Conclusions
Explored how \(\vec{L}\) of given fixed regions changes with changed \(\color{blue}\vec{L}_0\)
- angular momentum can be predicted accurately from initial conditions (not chaotic)
- possible to improve linear theory by predicting growth rate \(\color{red} f(t)\)
- apparent stochasticity of AM of halos ⇒ result of particle membership
Read more in Cadiou, Pontzen & Peiris arXiv:2012.02201.
Conclusions
What's next?
- apply to baryons instead of DM only?
- extend analysis to halos?
- predict \(\color{red}f(t)\) rather than measure it?
Read more in Cadiou, Pontzen & Peiris arXiv:2012.02201.
Explored how \(\vec{L}\) of given fixed regions changes with changed \(\color{blue}\vec{L}_0\)
- angular momentum can be predicted accurately from initial conditions (not chaotic)
- possible to improve linear theory by predicting growth rate \(\color{red} f(t)\)
- apparent stochasticity of AM of halos ⇒ result of particle membership
AM genetic modifications

Initial \(\vec{L}\) given in initial conditions by
$$ L_{z,0} \propto \sum_{i,j,k}\left[ (q_{x}^{ijk} - \bar{q}_x) \nabla_y\phi^{ijk} - (q_{y}^{ijk} - \bar{q}_y) \nabla_x\phi^{ijk}\right]. $$
Genetic modifications: find \(\vec{u}\) such that
$$ \vec{u} \cdot \vec{\delta} = \vec{L}_0,$$
with \(\vec{\delta} = \{\delta^{i_0j_0k_0}, \dots, \delta^{i_nj_nk_n}\} \) and solve for
$$ \vec{u}\cdot \vec{\delta}' = \vec{L}_0'$$
with minimal change.
deck
By Corentin Cadiou
deck
- 624



