Intersecting classical groups is in P
Peter Brooksbank Bucknell
Martin Kassabov Cornell
James B. Wilson Colorado State University
Euclid: Classical Geometry
Ingredients: Rock, paper, scissors

Hamilton: Paper=Vector Space
Cauchy: Rock + scissors = Norm, Inner product;
In fact, you can use the scissors as your straight-edge if you want, i.e. Inner product gets you a norm.
Classified at this point
\(\langle|\rangle:M\times M\rightarrowtail \mathcal{O}\) where \[\langle u|v\rangle =\bar{u}^tDv^d\]
- Orthogonal: \(\mathcal{O}\) field \(K\), identity involution, \(M=K^d\), \(D\) diagonal, determinant square class defines the geometry.*
- Unitary: \(\mathcal{O}=\left(\frac{\alpha}{K}\right)\) involution swaps roots (note may be split), \(D=I_d\).
- Symplectic:\(\mathcal{O}=\left(\frac{\alpha,\beta}{K}\right)\), adjugate involution, \(M=(\mathcal{O}e)^{d}\) if split \(e^2=e\not\in \{0,1\}\); else \(M=\mathcal{O}^d\), \(D=I_d\).
- Exceptional:\(\mathcal{O}=\left(\frac{\alpha,\beta}{K}\right)\), \(M=\mathcal{O}^{1,3}\), octonion/Albert involution, \(D=I_d\).
*If \(2K=0\) then orthogonal uses norm not product.
Neo-Classical Geometry
Ingredients: One paper, but a pile of scissors

(Why did he replace compass with scissors?)
Cause he could find a photo of lots of scissor, duh!
The Kronecher Challenge

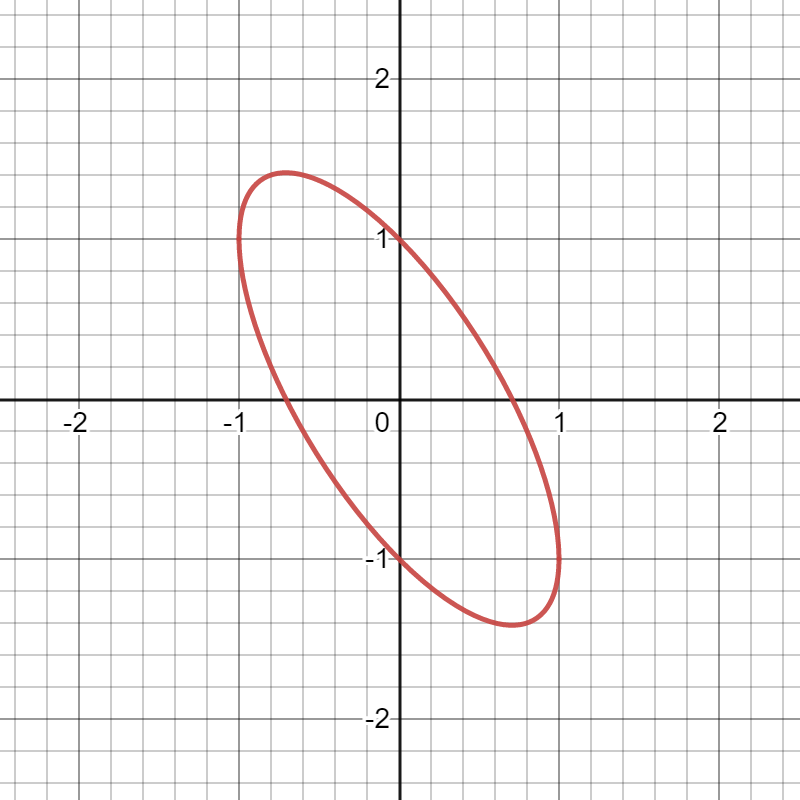
Start with Euclidean inner product
Unit circle Drawn
Find orthogonal basis
Everyone can see one,
the x,y axes.
Fix some other
inner product
Unit circle Drawn
Find orthogonal basis
Everyone knows it can be done...

...Gram-Schmidt should not be done in public...
Unit circle Drawn
- Start with \(e_1, e_2\)
- \(u_1=e_1\)
- Replace \(u_2=e_2-\frac{\langle e_2|u_1\rangle}{\langle u_1|u_1\rangle}u_1=\begin{bmatrix} -\frac{1}{2} \\ 1 \end{bmatrix}\)
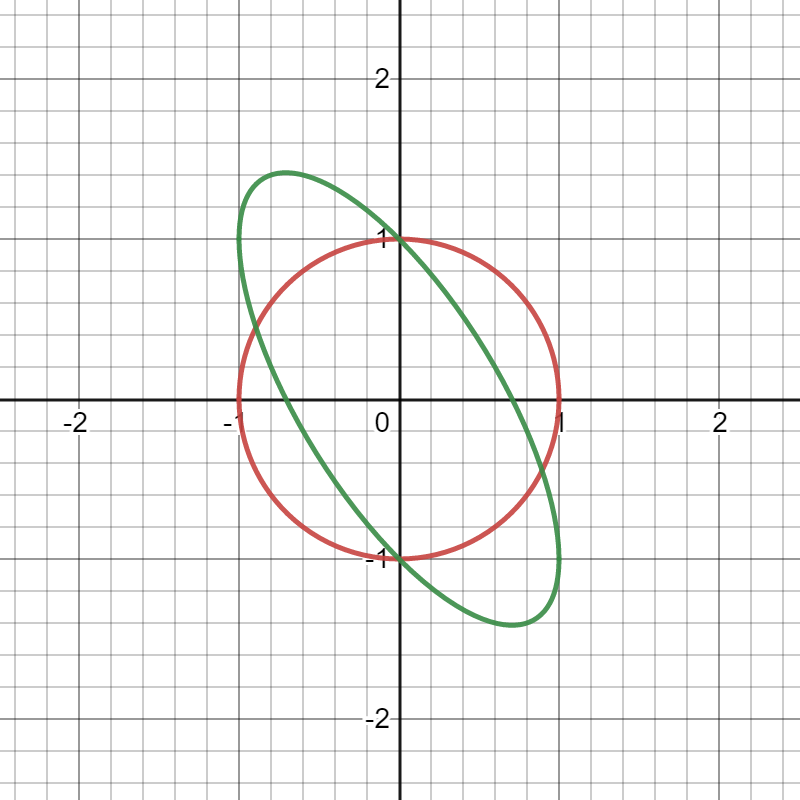
Now Find a Simultaneous
orthonormal basis for both!
Unit circles Drawn
\(e_1\perp e_2\) for Euclidean
but \(\langle e_1|e_2\rangle\neq 0\)
\(\langle u_1,u_2\rangle=0\) but \((u_1|u_2)\neq 0\)

...Gram-Schmidt should not be done in public...
Unit circle Drawn
- Start with \(e_1, e_2\)
- Normalize \(e_1\) as \(u=\frac{e_1}{\sqrt{\langle e_1|e_1\rangle}}=\begin{bmatrix} \frac{\sqrt{2}}{2}\\ 0 \end{bmatrix}\)
- Replace \(u_2=e_2-\frac{\langle e_2|u_1\rangle}{\langle u_1|u_1\rangle}u_1=\begin{bmatrix} -\frac{1}{2} \\ 1 \end{bmatrix}\)
- Norm. \(u_2=\frac{u_2}{\sqrt{\langle u_2|u_2\rangle}}=\begin{bmatrix} -\sqrt{2}/2\\\sqrt{2}\end{bmatrix}\)
The Problem
- ONE INNER PRODUCT: BE GREEDY. Every nonzero vector \(v\) is orthogonal to a hyperplane \(H=v^{\bot}\), recurse.
-
TWO INNER PRODUCTS:
- \(v\) is perpendicular to \(H_1=v^{\bot(1)}\) and \(H_2=v^{\bot(2)}\).
- Only get an simultaneous ortho. basis if \(H_1=H_2\).
- Generically \(H_1\cap H_2\) has codimension 2, so not equal.
- Greed fails. You must know where to start.
Specific Problems
Given bilinear forms \(\Phi=\{\phi:V\times V\rightarrowtail L_{\phi}\}\) (where each \(L_{\phi}/K\) is a field extension)
Describe the \(\Phi\)-geometry.
- Give the maximal simultaneous orthogonal decomposition; are these all equivalent?
- Find its group of isometires \[\mathrm{Isom}(\Phi)=\{f\in \mathrm{GL}(V)\mid \phi(f(u),f(v))=\phi(u,v)\}\]
- Decide if two such systems \(\Phi\) and \(\Gamma\) are isometric.
- Classify the \(\Phi\)-geometries
Classification \(\{\phi_1,\phi_2\}\)
- Kronecher: Over \(\mathbb{R}\), with gaps.
- Gantmacher: over \(\mathbb{C}\)
- Dieudonne: algebraically closed.
- Scharlau: Alternating but any field.
\(\Phi\)-geometry structure
- Serre: Is it wild? Bayer-Fluckiger: Yes.
- Tang: max \(\bot\)-decompositions are not the same length.
- Goldstein-Guralnick: generically for list of alternating.
- Brooksbank-O'Brien: approximation algorithm to intersection.
Theorem (W. 2008)
- If \(K\) has non-squares than \(\Phi\)-geometries can have any number of equivalence classes of max. \(\bot\)-decompositions.
- If \(K=2K\) all max. \(\bot\)-decomps are the same size.
- \(\exists\) a signature that labels each equiv. class.
Given a system \(\Phi=\{\varphi:V\times V\rightarrowtail L_{\phi}\}\) of bilinear forms, there is a polynomial-time algorithm to compute the group of isometries.
Theorem (Brooksbank-W. 2011)
Fix a field \(K=2K\) where you can factor polynomials and solve linear equations.
Given a system \(\Phi=\{\varphi:V\times V\rightarrowtail L_{\phi}\}\) of bilinear forms, there is a polynomial-time algorithm to compute the group of isometries.
Theorem (Ivanyos-Qiao 2016)
Fix a field \(K=2K\) where you can factor polynomials and solve linear equations.
Given two systems \(\Phi,\Gamma\) can decide isometry.
Some Consequences
Theorem. Ivanyos-Qiao
For \(p\neq 2\) average case class 2 \(p\)-group isomorphism is in polytime.
Theorem. Ivanyos-Qiao
For \(2K=K\) breaks the Patarin post-quantum cypher.
Theorem. Li-Qiao
For \(2K=K\) solve isometry in polytime.
Theorems...Li-Qiao, Li, Qiao, et. al.
Complexity of tensor graph analogs.
Theorems. Grochow-Qiao
Group isomorphism by extension in polytime; tensor isomorphism, complixity classes, etc.
Brooksbank-Maglione-O'Brien-W.
Theorem. Lewis-W.
For \(p\neq 2\) isomorphism of quotients of Heisenberg groups in polytime.
Find characteristic subgroups, isomorphism test, invariants.
Algorithm. Brooksbank-W.
For \(p\neq 2\) adjoint-tensor isomorphism test.
Theorem. W.
The optimal bound on Gowers profiles is \(\log n-2\).
Theorem. Brooksbank-Maglione-W.
Isomorphism testing genus 2 is polytime.
Theorem. Kassabov-Tybursky-W.
Subgroup lattices of simple groups are as diverse as possible.
Defn. W.
\(\alpha\)-Filter refinement.
\(\alpha\)-filters in polytime.
Theorem. Brooksbank-Li-Qiao-W.
A Weisefeller-Lehman for groups
Theorems. Maglione
Proof Sketch \(2K=K\)
Outline
- \(\mathrm{Isom}(\Phi) = \mathrm{Adj}(\Phi)^{\#}\)
- Taft: \(2K=K\) implies \((A,*) = (S,*)\ltimes (J(A),*)\)
- Albert: \((S,*)=(S_1,*_1)\oplus \cdots\oplus (S_{\ell},*_{\ell})\) *-simples
- Weil: \((S_i,*_i)\cong \mathrm{Adj}(\phi_i:V_i\times V_i\rightarrowtail L_i)\)
- Step I. Find ways to detect all this.
- Step II. write down \(\bot_i\)-bases of \(V_i\).
- Step III. Write down Steinberg generators.
- Step IV. Glue along diagonal.
- Step V. Magic power series \(z+\sqrt{1+z^2}\) a basis of \(\mathrm{Alt}\cap J(A)\)
Further Consequence
Precise structure formula \(\forall \Phi,\exists \tau_1,\ldots,\tau_{\ell}\) where \[\begin{aligned} \mathrm{Isom}(\Phi)&=(\mathrm{Isom}(\tau_1)\times\cdots\times \mathrm{Isom}(\tau_{\ell}))\ltimes O_p\\ O_p & = \{z+\sqrt{1+z^2}\mid \exists n.(z^n=0)\wedge(z^*=-z)\}.\end{aligned}\]
Informally: Neo-classical geometry decomposes into a series of classical geometries.
Why not \(2K=0\)?
\(2K=K\)
- Uni-triangular Isometries \[\{z+\sqrt{1+z^2}\mid \exists n.(z^n=0), z^*=-z\}\]
- Taft decomposition \[(A,*)=(S,*)\ltimes J(A)\]
- \((\mathbb{M}_d(K),*)=\mathrm{Sym}\oplus \mathrm{Alt}\)
- \(\mathrm{Isom}(\phi)=\mathrm{Adj}(\phi)^{\#}\)
\(2K=0\)
- \(z+\sqrt{1+z^2}\) divides by 0.
- \(\exists (A,*)\) where \(J(A)\) does not split.
- \(\mathrm{Alt}\leq \mathrm{Sym}\)
- Orthogonal groups are not unitary for ring involutions.
Why care about \(2K=0\)?
- Post-quant crypt. is over \(\mathbb{F}_2\)
- Quantum Information Theory is over \(\mathbb{F}_2\)
- 2-groups are the dominant obstacle to group iso. (and group(-oid) iso is the problem that impeded deciding high-order equivalence)
- When there is a hole in the bucket it just bothers you.
Theorem (Brooksbank-Kassabov-W. 2021)
It all works in \(2K=0\) case.
So what is new?
\(z+\sqrt{1+z^2}\) asks too much
- Use a semi-standard induction through linear layers
- Actually you discover along the way that this induction has infinitely many cut-downs, no power series can exist.
Almost always Taft decompositions exist for subalgebras that contain \(A^{\#}\)
In non-orthogonal type this happens and the invariant is to commute with a derivation (1-cocycle).
How do show this? Assume so, write down what it would require, call all failures "exceptional".
The exceptional case
Over \(L=K[t]/(t^2)\) in \(\mathbb{M}_{2m}(L)\) make
No Taft splitting.
Isometries of \((A,*)/(J(A),*)\) do not lift.
Those that lift are
\[S^{\diamond}=\{s\in S\mid s^*s\equiv 1\mod{J(A)}, \exist m.((s+m)^*(s+m)=1)\}\]
These are quadratic equations, no hope to solve.
Reconfigure
After some algebra
\[\exists m.(\delta(s)= sm+ms)\]
\[S^{\diamond}=\{s\in S\mid sm\bar{s}=m\}\]
Still quadratic, but concentrates on a single defect, \(m\).
Freshman's Dream
If \(2K=0\) then for all \(M\in \mathrm{Sym}\),
\[(A+B)M(A+B)^*\equiv AMA^*+BMB^*\pmod{\mathrm{Alt}}\]
Professor's Dream
If \(pK=0\) then \((a+b)^p=a^p+b^p\).
Fix involution \(M\mapsto M^*\) on \(\mathbb{M}_d(K)\),
\[\mathrm{Sym}=\{X\mid X^*=X\}\qquad \mathrm{Alt}=\{X\mid X^*=-X,\textnormal{0 diag}\}\]
\(2K=0\) need
If \(2K=0\) then for all \(M\in \mathrm{Sym}\),
\[(A+B)M(A+B)^*\equiv AMA^*+BMB^*\pmod{\mathrm{Alt}}\]
Professor's Dream
Fix involution \(M\mapsto M^*\) on \(\mathbb{M}_d(K)\),
\[\mathrm{Sym}=\{X\mid X^*=X\}\qquad \mathrm{Alt}=\{X\mid X^*=-X,\textnormal{0 diag}\}\]
Corollary. The isometries that lift are
\[\begin{aligned}S^{\diamond} & = \{s\mid \bar{s}s=1, sm\bar{s}=m\}\\ & = \{s\mid \bar{s}s=1, s\cdot m=m\}\\ & =\mathrm{Stab}_{\mathrm{Isom}(\phi)}(M)\end{aligned}\]
This is a known group: a max parabolic in an orthogonal group. You just write it down!
IsometriesChar2
By James Wilson
IsometriesChar2
Intersecting classical groups in characteristic 2 is in P.
- 532



