Legislative Bargaining
Lecture 5, Political Economics I
OSIPP, Osaka University
17 November, 2017
Masa Kudamatsu
Motivation #1
The models we have discussed so far in this course
Many elected politicians negotiate with each other to enact policies
The single elected politician can unilaterally implement policies
In reality
e.g. Fiscal budget has to be approved by legislature
The legislative bargaining model
is a framework to analyze such collective decision-making
Motivation #2
The legislative bargaining model
is another way to pin down an equilibrium policy in such cases
For policy issues where there is no median voter (cf. Lecture 1)
e.g. The division-of-a-pie problem
We need a model that allows us to find an equilibrium
e.g. Probabilistic Voting Model (cf. Lecture 3)
Motivation #3
The legislative bargaining model is an extension to allow
(1) multiple players
(2) majority voting, rather than unanimity
In game theory
The Rubinstein bargaining game is a framework
to analyze the negotiation between two persons
Basic Model
with one-dimensional policy space
Originally proposed by Baron and Ferejohn (1989)
Here we follow Section 5.4 of Persson and Tabellini (2000)
Players
Three legislators, \(J \in \{L,M,R\}\)
Think of each as a citizen-candidate elected in each constituency
Endowments
Each legislator \(J\) is endowed with income \(y^J\) with \(y^L<y^M<y^R\)
Preference
Government budget constraint
where \(y\) is per capita income w/ pop size 1
Each legislator's bliss point
Government budget constraint
where \(y\) is per capita income w/ pop size 1
Preference
Plugging the budget constraint into legislator J's preference...
Each legislator's bliss point
First order condition yields:
Each legislator's bliss point
First order condition yields:
So we have:
Timing of Events
1
2
3
4
Nature picks \(J \in \{R,M,L\}\) as the agenda setter
Denote this legislator by \(A\)
Legislator \(A\) proposes \(g_A\)
All legislators vote on the proposal \(g_A\)
If at least two parties vote yes, \(g_A\) will be implemented
Otherwise, the default policy, \(\bar{g}\), will be implemented
"Agenda setter" in reality
Cabinet ministers in parliamentary systems (UK, etc.)
Legislative committees in presidential systems (US etc.)
Agriculture Appropriations Armed Services Budget
Education and the Workforce Energy and Commerce Ethics
Financial Services Foreign Affairs Homeland Security
House Administration Judiciary Natural Resources
Oversight and Government Reform Rules
Science, Space, and Technology Small Business
Transportation and Infrastructure Veterans' Affairs Ways and Means
Source: www.congress.gov/committees
Committees in U.S. Lower House
We will see this committee in action later
Default policy in reality #1
Government shutdown in U.S.
October 1st-16th, 2013
Due to the deadlock between the Republican-controlled House
and the Democrat-controlled Senate over Obamacare

Most recent case:
Default policy in reality #2
e.g. Art. 312 of Treaty on the Functioning of the European Union
Where no Council regulation determining a new financial framework has been adopted by the end of the previous financial framework, the ceilings and other provisions corresponding to the last year of that framework shall be extended until such time as that act is adopted.
"
"
(Quoted by Piguillem and Riboni (2015), footnote 8)
The policy adopted in the previous fiscal year
Default policy in reality #1
Government shutdown in U.S.
October 1st-16th, 2013
Due to the deadlock between the Republican-controlled House
and the Democrat-controlled Senate over Obamacare

Most recent case:
Analysis
Backward induction
1
2
3
4
Nature picks \(J \in \{R,M,L\}\) as the agenda setter
Denote this legislator by \(A\)
Legislator \(A\) proposes \(g_A\)
All legislators vote on the proposal \(g_A\)
If at least two parties vote yes, \(g_A\) will be implemented
Otherwise, the default policy, \(\bar{g}\), will be implemented
Backward induction
Legislators \(J \neq A\) vote yes to \(g_A\) as long as
Since \(W^J(g)\) is single-peaked, this means
Backward induction
Legislators \(J \neq A\) vote yes to \(g_A\) as long as
Since \(W^J(g)\) is single-peaked, this means
Accept
Backward induction
1
2
3
4
Nature picks \(J \in \{R,M,L\}\) as the agenda setter
Denote this legislator by \(A\)
Legislator \(A\) proposes \(g_A\)
All legislators vote on the proposal \(g_A\)
If at least two parties vote yes, \(g_A\) will be implemented
Otherwise, the default policy, \(\bar{g}\), will be implemented
Case 1: Legislator M as the agenda setter
Case 1: Legislator M as the agenda setter (cont.)
Suppose
Case 1: Legislator M as the agenda setter (cont.)
Suppose
Legislator R will vote yes if
Case 1: Legislator M as the agenda setter (cont.)
Suppose
Legislator L will vote yes if
Legislator M's bliss point \(g^M\) will be accepted by L
It's optimal to propose \(g_A = g^M\)
Case 1: Legislator M as the agenda setter (cont.)
Suppose
Case 1: Legislator M as the agenda setter (cont.)
Legislator L will vote yes if
Suppose
Case 1: Legislator M as the agenda setter (cont.)
Legislator R will vote yes if
Suppose
Legislator M's bliss point \(g^M\) will be accepted by R
It's optimal to propose \(g_A = g^M\)
Case 1: Legislator M as the agenda setter (cont.)
For all \(\bar{g}\),
Legislator M proposes \(g_A = g^M\)
Then
R votes in favor if \(\bar{g} > g^M \)
L votes in favor otherwise
So far the prediction is the same as the median voter theorem
Case 2: Legislator L as the agenda setter
Case 2: Legislator L as the agenda setter
Suppose
Case 2: Legislator L as the agenda setter
Suppose
Legislator R will vote yes if
Case 2: Legislator L as the agenda setter
Suppose
Legislator M will vote yes if
Case 2: Legislator L as the agenda setter
Suppose
Legislator M will vote yes if
Legislator R will vote yes if
Legislator L's bliss point \(g^L\) will be accepted by both
It's optimal to propose \(g_A = g^L\)
Case 2: Legislator L as the agenda setter
| Default policy | Equilibrium policy |
|---|---|
|
|
|
|
|
|
|
|
Case 2: Legislator L as the agenda setter
Suppose
Case 2: Legislator L as the agenda setter
Suppose
Legislator R will vote yes if
Case 2: Legislator L as the agenda setter
Suppose
Legislator M will vote yes if
Case 2: Legislator L as the agenda setter
Suppose
Legislator M will vote yes if
Legislator R will vote yes if
Default policy \(\bar{g}\) yields the highest payoff to L
among those to be approved
It's optimal to propose \(g_A = \bar{g}\)
Case 2: Legislator L as the agenda setter
| Default policy | Equilibrium policy |
|---|---|
|
|
|
|
|
|
|
|
Case 2: Legislator L as the agenda setter
Suppose
Case 2: Legislator L as the agenda setter
Suppose
Legislator R will vote yes if
Case 2: Legislator L as the agenda setter
Suppose
Legislator M will vote yes if
If
it's optimal to propose
and M will vote yes
Case 2: Legislator L as the agenda setter
Suppose
Legislator M will vote yes if
If
it's optimal to propose
and M will vote yes
Case 2: Legislator L as the agenda setter
| Default policy | Equilibrium policy |
|---|---|
|
|
|
|
|
|
|
|
Case 2: Legislator L as the agenda setter
| Default policy | Equilibrium policy |
|---|---|
|
|
|
|
|
|
|
|
Whatever \(\bar{g}\) is, L can implement \(g_A \in [g^M, g^L] \)
Case 2: Legislator L as the agenda setter
Whatever \(\bar{g}\) is, L can implement \(g_A \in [g^M, g^L] \)
This is the agenda-setting power
Case 2: Legislator L as the agenda setter
Whatever \(\bar{g}\) is, L can implement \(g_A \in [g^M, g^L] \)
M is always better off than R
because M's support is cheaper for L to buy than R's
Case 3: Legislator R as the agenda setter
Case 3: Legislator R as the agenda setter
By the symmetric argument, R can implement
Extension to
the division-of-a-pie problem
Players
Three legislators, \(J \in \{1,2,3\}\)
Think of each as a citizen-candidate elected in each constituency
Preference
Government budget constraint
where \(T\) is exogenous revenue
Timing of Events
1
2
3
4
Nature picks \(J \in \{1,2,3\}\) as the agenda setter
Without loss of generality, let legisator 1 be chosen
Legislator 1 proposes the allocation \(\mathbf{g} = (g^1, g^2, g^3)\)
All legislators vote on the proposal \(\mathbf{g}\)
If at least two parties vote yes, \(\mathbf{g}\) will be implemented
Otherwise, the default policy, \(\bar{\mathbf{g}} = (\bar{g}^1,\bar{g}^2,\bar{g}^3)\), will be implemented (with \(\sum_J \bar{g}^J < T\))
Analysis
Legislator \(J \neq 1 \) will vote yes if
Legislator 1's payoff
To minimize \(\sum_{J\neq 1}g^J\)
Majority voting implies only one more legislator's support is needed
for all \(J \neq 1\)
if \(\bar{g}^2 \leq \bar{g}^3\)
if \(\bar{g}^2 \geq \bar{g}^3\)
Implications
1
2
The equilibrium exists where the Downsian model has none
Agenda-setter keeps the largest share of the pie
If \(\bar{g}^2 < \bar{g}^3\)
Implications
1
2
3
4
The equilibrium exists where the Downsian model has none
Agenda-setter keeps the largest share of the pie
Minimum winning coalition: one legislator gets zero
The legislator with worse outside option (i.e. default policy)
is chosen to be part of the winning coalition
Extension to multiple rounds of bargaining
We can modify the timing of events in the following way
If the proposal is voted down,
nature picks a new agenda-setter
and repeat the same bargaining protocol
Then the legislator who's likely to be a next agenda setter
has a higher outside option
Will be excluded from the winning coalition
Likelihood of becoming an agenda-setter
e.g. Negotiation on the coalition government formation
when no political party won the majority of legislative seats
The largest party is usually given the role of proposer
If the negotiation breaks down, the second largest party is chosen
Probability of becoming an agenda setter
= Seat share in the legislature
See Merlo (1997 JPE)
Endogenous default policy
Pioneer: Baron (1996 APSR) on one-dimensional policy model
If the proposed bill is rejected
the previous-period policy continues to be implemented
Kalandrakis (2004 JET) considers the division-of-a-pie policy
Diermeier and Fong (2011 QJE) allow the same legislator to remain as the agenda-setter
Evidence
Predictions from legislative bargaining model
Knight (2005 AER) tests these two predictions in U.S.
1
Majority
voting
Some non-agenda
setters receive zero
Agenda setters
propose the allocation
Their district receives
more funds
2
Consider the allocation of funds across districts each represented by a legislator
Highway Trust Fund in U.S.
Drivers
Federal govt
Gasoline tax
State govts
Fiscal transfer
Highways
Construct & Maintain
Congress chooses
which highways to be financed
($5b in 1991, $8b in 1998)
Testing ground:
Legislative process for Highway Trust Fund
House of Representatives (435 members)
House Committee
on Transportation
and Infrastructure
(55 or 72 members)
Fund allocation bill
Propose
Vote
Testing ground:
Data
Based on project descriptions in the bill,
match each highway project with a congressional district
Predictions from legislative bargaining model
Knight (2005 AER) tests these two predictions in U.S.
1
Majority
voting
Some non-agenda
setters receive zero
Agenda setters
propose the allocation
Their district receives
more funds
2
Consider the allocation of funds across districts each represented by a legislator
% of congressional districts receiving zero
| Committee members' | The others | |
|---|---|---|
| 1991 | 0 | 72 |
| 1998 | 0 | 21 |
Source: Table 1 of Knight (2005)
Predictions from legislative bargaining model
Knight (2005 AER) tests these two predictions in U.S.
1
Majority
voting
Some non-agenda
setters receive zero
Agenda setters
propose the allocation
Their district receives
more funds
2
Consider the allocation of funds across districts each represented by a legislator
Average Allocated Spendings
| Committee members' | The others | |
|---|---|---|
| 1991 | $54.8m | $6.1m |
| 1998 | $38.5m | $13.8m |
Source: Table 1 of Knight (2005)
Empirical specification
For each year of 1991 and 1998, estimate with OLS:
Highway fund spending in district \(d\) (in millions of 1998 dollars)
Indicator of district \(d\)'s representative being in the committee
District \(d\)'s characteristics
OLS estimation results

Source: Table 2 of Knight (2005)
Are you convinced?
Alternative explanation of OLS results
Legislators may join the committee
because their districts need more highways
In need of highways
Alternative empirical specification #1
Restrict the sample to those districts that can be matched
District fixed effects
Control for unobservable time-invariant district characteristics
But due to the redrawing of districts in 1992,
some districts cannot be matched between 1991 and 1998
Aggregate at the state level by taking the averages
State fixed effects
Fixed effects estimation results
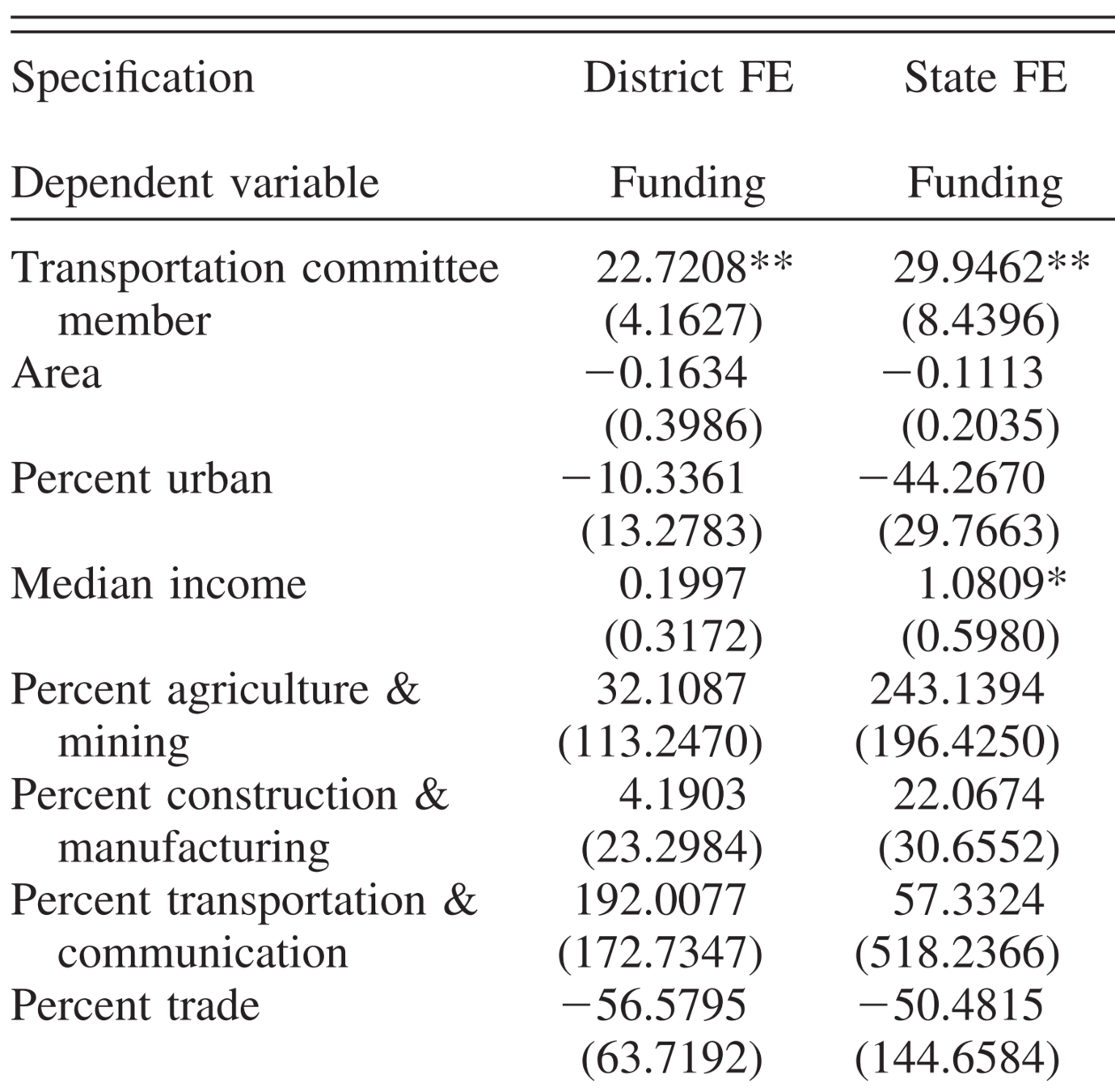

Source: Table 5 of Knight (2005)
Are you convinced?
Alternative explanation of FE results
Committee members in 1991 leave the committee
because their districts no longer need more highways

Decline in the need of highways
Alternative empirical specification #2
Newly elected legislators are more likely to join the committee
Use the indicator of being newly elected as an instrument
Are you convinced?
First-stage does work: 8.5 pt increase (sample mean 15%)
Threats to the exclusion restriction
Newly elected legislators are less powerful
This may directly affect the allocation of funds to their district
Takeaway
The positive correlation
between committee membership & allocated funds
Consistent with theoretical predictions
from legislative bargaining model
Application:
Presidentialism
vs
Parliamentarism
Adapted from Persson et al. (2000 JPE)
(See also sections 10.2-10.3 of Persson and Tabellini 2000)
Motivation:
Impacts of political institutions
on economic policies
We have seen the impacts of
Electoral rules (Lecture 3)
Term limits (Lecture 4)
Another major difference in political institutions across countries
Presidentialism vs Parliamentarism
A Model of
Fiscal Policy-making
Players
Three legislators, \(J \in \{1,2,3\}\)
Each represents a region (or a group of voters)
Without loss of generality, assume:
Legislator 1 is in charge of proposing the tax policy
Legislator 2 is in charge of proposing the expenditure policy
Legislator 3 is the minority party member
Preference
Legislator \(J\)'s payoff
Exogenous income
Lump-sum tax
Public goods (benefiting all legislators)
Transfer to region \(J\)
Preference
Legislator \(J\)'s payoff
Each legislator can be thought of as
a citizen-candidate who won the election in each region
Actions
Legislator 1 proposes \(\tau\)
Legislator 2 proposes \(g\), \(\{f^J\}\)
e.g. Finance Minister
Member of Congress Committee on Taxation
e.g. Other Ministers
Members of Congress Committees on Expenditure
All legislators vote on these proposals
Actions
Legislator 1 proposes \(\tau\)
Legislator 2 proposes \(g\), \(\{f^J\}\)
All legislators vote on these proposals
Which action follows which
depend on the form of government
Modelling
forms of government
Differences between
Presidentialism and Parliamentarism
Major difference: how the chief executive is elected
Other important differences: How legislature makes policies
But we do not model this here
Presidentialism
Different congressional committees hold proposal power over different policy issues
Separation of Power
=
Taxation


Spending
Parliamentarism
Ruling party legislators propose almost all bills
A disagreement within ruling party members leads to a government crisis (vote of no confidence 「内閣不信任決議」)
June 1993: Vote of no confidence against Prime Minister Miyazawa was approved as some LDP members voted in favour

Legislative cohesion
=
Fiscal policy in
presidentialism
Timing of Events in Presidentialism
1
2
3
4
Legislator 1 proposes total tax revenue, \(\tau\)
Legislators vote on the tax proposal
If rejected, the default policy, \(g=f^1=f^2=f^3=0\), is implemented
Legislator 2 proposes expenditures on public goods (\(g\)) and transfer to each region (\(f^1, f^2, f^3\))
Legislators vote on the expenditure proposal
If rejected, the default policy, \(\bar{\tau}=0\), is implemented
Backward induction
1
2
3
4
Legislator 1 proposes total tax revenue, \(\tau\)
Legislators vote on the tax proposal
If rejected, the default policy, \(g=f^1=f^2=f^3=0\), is implemented
Legislator 2 proposes expenditures on public goods (\(g\)) and transfer to each region (\(f^1, f^2, f^3\))
Legislators vote on the expenditure proposal
If rejected, the default policy, \(\bar{\tau}=0\), is implemented
Legislators 1 & 3's optimal voting
on the expenditure proposal
Both prefer anything only slightly better than the default policy
Legislator \(J\) accept the proposal if
(Assuming they will accept when indifferent between the bill and the default policy)
Backward induction
1
2
3
4
Legislator 1 proposes total tax revenue, \(\tau\)
Legislators vote on the tax proposal
If rejected, the default policy, \(g=f^1=f^2=f^3=0\), is implemented
Legislator 2 proposes expenditures on public goods (\(g\)) and transfer to each region (\(f^1, f^2, f^3\))
Legislators vote on the expenditure proposal
If rejected, the default policy, \(\bar{\tau}=0\), is implemented
Legislator 2's optimal proposal on expenditure
Choose policies to solve
subject to
First, there is no need to provide positive \(f^1, f^3\) to pass the bill
Legislator 2's optimal proposal on expenditure
First order condition:
The remaining tax revenue is used for transfer to region 2
Backward induction
1
2
3
4
Legislator 1 proposes total tax revenue, \(\tau\)
Legislators vote on the tax proposal
If rejected, the default policy, \(g=f^1=f^2=f^3=0\), is implemented
Legislator 2 proposes expenditures on public goods (\(g\)) and transfer to each region (\(f^1, f^2, f^3\))
Legislators vote on the expenditure proposal
If rejected, the default policy, \(\bar{\tau}=0\), is implemented
Legislator 1's optimal tax proposal
Choose \(\tau\) to solve
subject to
Starting with \(\tau=0\), legislator 2 will first spend any increase in \(\tau\) on \(g\)
Legislator 1's optimal tax proposal
Starting with \(\tau=0\), legislator 2 will first spend any increase in \(\tau\) on \(g\)
Until the marginal utility from \(g\) reaches 1
Then legislator 2 will spend any extra increase in \(\tau\) on \(f^2\)
Legislator 1 benefits as well
Legislator 1 no longer benefits
So it's optimal for legislator 1 to set
Both legislators are happy to accept
Fiscal policy in
parliamentarism
Timing of Events in Parliamentarism
1
2
Legislators 1 & 2 (i.e. the cabinet) jointly propose the policy package, \(\tau, g, f^1, f^2, f^3\)
Legislators vote on the proposal
If rejected, the cabinet resigns; the default policy, \(\bar{\tau}=g=f^1=f^2=f^3=0\), is implemented
Transfer to opposition's region
No support from the opposition is needed to pass the bill
Public goods & transfer to ruling party's regions
Legislators 1 & 2 jointly maximize their payoffs
Given \(\tau\), they choose \(g\) to solve
Govt budget constraint & \(f^3=0\)
FOC w.r.t. \(g\)
Size of government
Legislators 1 & 2 jointly maximize their payoffs
Given the optimal \(g^{PA}\), they choose \(\tau\) to solve
FOC w.r.t. \(\tau\)
Extracting income from region 3
to split it among the two regions
Comparative politics
Presidentialism
Parliamentarism
Public good provision
Regional transfer
Tax revenue
Evidence on the impact of forms of government
Causal evidence?
Hard to prove causality running from forms of govt to policies
Forms of government rarely change
Thus impossible to separate their impact
from that of country characteristics

Causal evidence?
We can only check
if correlation is consistent with the theoretical prediction

See also Persson and Tabellini (2003) and Acemoglu (2005)
Run cross-country regressions of fiscal policies on forms of govt
Indicator of presidentialism in country \(i\)
Theory predicts \(\beta < 0\) when \(y_i\) is the size of government
See also Persson and Tabellini (2003) and Acemoglu (2005)
Run cross-country regressions of fiscal policies on forms of govt
Per capita GDP
Trade openness
Population
% of those aged 16-54
% of those aged over 65
Years of being democracy
Quality of democracy (Freedom House Index)
Dummy for majoritarian elections
(cf. Lecture 3)
Dummy for federal states
Dummy for OECD countries
Dummies for continents
Dummies for legal origins
Forms of government across the world in 1998
Source: Figure 4.1 of Persson and Tabellini (2003)
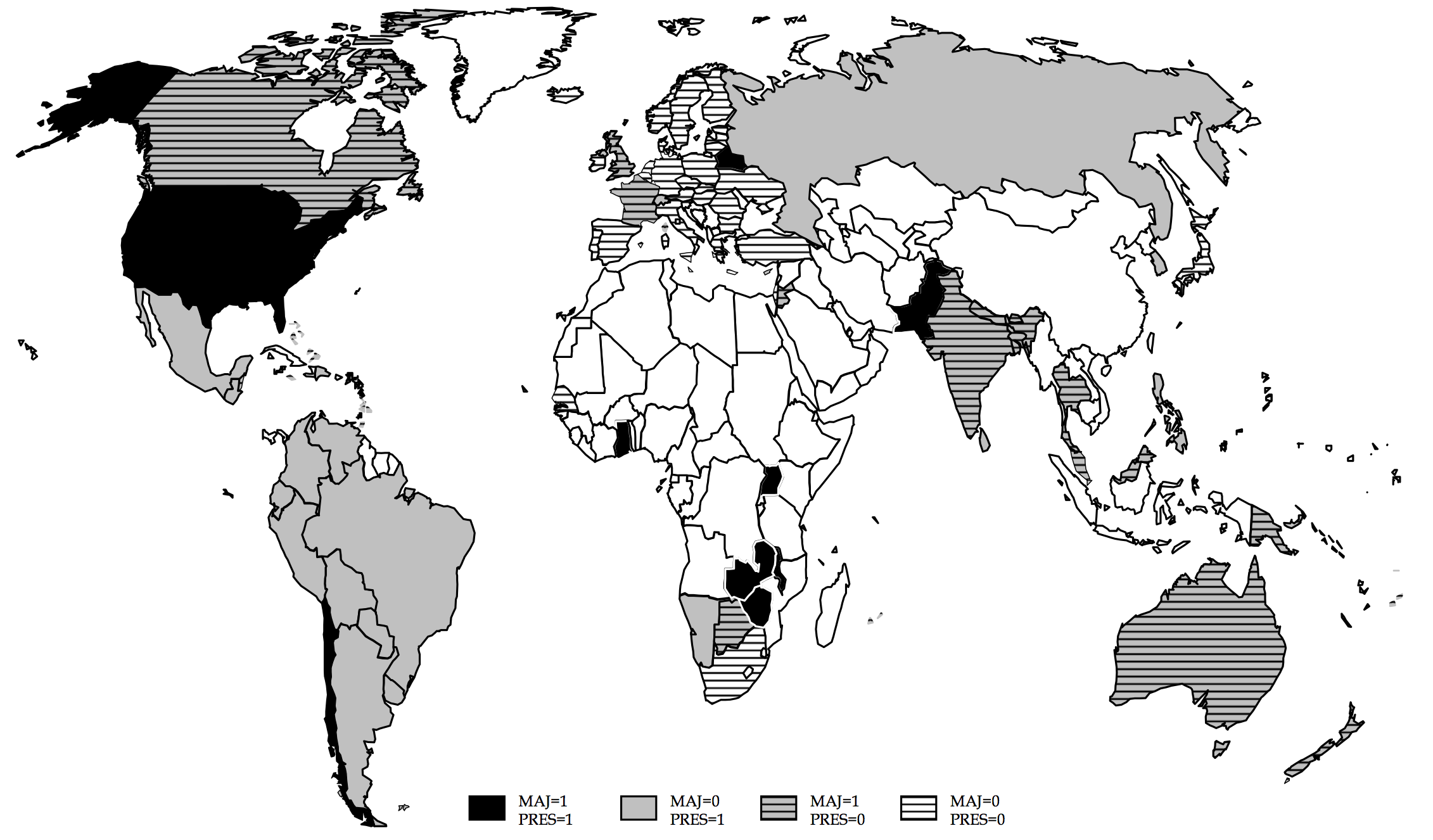
Presidential
Parliamentary
Not democratic
(excluded from the sample)
Unconditional mean comparison
| Fiscal policy (as % of GDP) |
Central govt expenditure | Social protection |
| Presidential system | 22.2% (7.2) |
4.8% (4.6) |
| Parliamentary system | 33.3% (10.0) |
9.9% (7.0) |
| p-value for two-sample t-test |
Source: Table 1 of Persson and Tabellini (2004)
Note: Standard deviation in parentheses
0.00
0.00
OLS estimation results
| Dep. Var. (as % of GDP) |
Central govt expenditure | Central govt revenue | Government deficit | Social protection |
| Presidentialism | -5.18*** (1.93) |
-5.00** (2.47) |
0.16 (1.15) |
-2.24* (1.11) |
| # observations | 80 | 76 | 72 | 69 |
Source: Tables 2 and 4 of Persson and Tabellini (2004)
Countries with presidential system
have a smaller size of government
spend less on social protection (pension, unemployment benefits, child allowance, etc.)
Endogenous presidentialism
Motivation
Almost all countries in Latin America and Africa
have adopted presidentialism
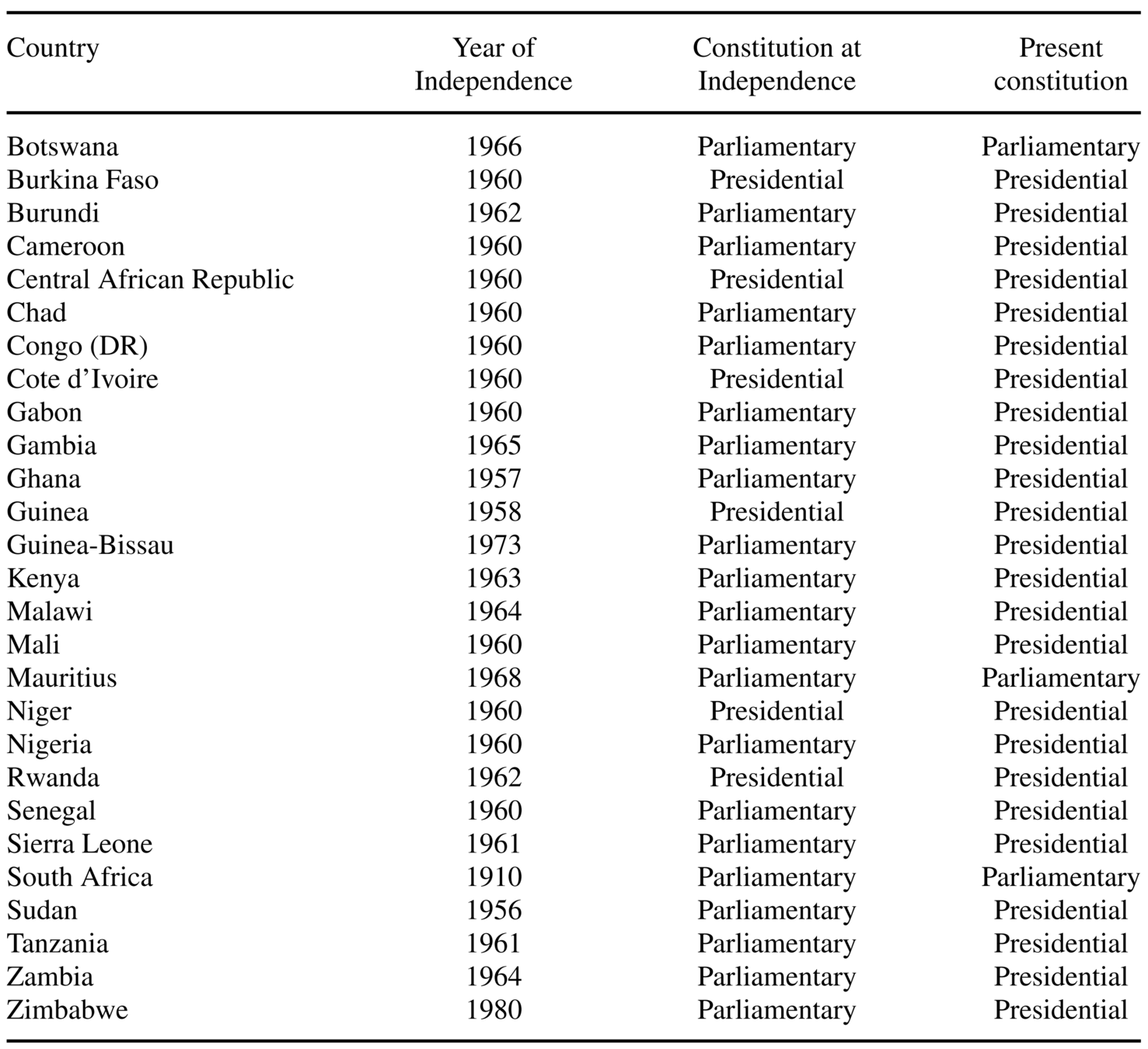
Source: Table 1 of Robinson and Torvik (2016)
Only 3 countries are parliamentary
No transition to parliamentary
Endogenous presidentialism (cont.)
Minority parties: more powerful in parliamentarism
They can hold a minority government
President as agenda-setter always excludes them from coalition (at least until next presidential election)
Presidents: more powerful than prime minister in parliamentarism
Cannot be kicked out by parliament
Prime minister needs to maintain the support of MPs
Endogenous presidentialism (cont.)
Politicians from the majority group
Parliamentarism is better to control political leader
Presidentialism is better to silence minority groups
Support presidentialism if losing power to minority groups is very costly
Preference between groups is polarized
Govt budget is small so the benefit of parliamentarism is small as well
e.g. Ethnic diversity in Africa
e.g. Weak fiscal capacity in Latin America and Africa
Present-biased legislators
When legislators want to increase current spending and procrastinate spending cut...
Disagreement in legislature attenuates over-spending
Derived from the assumption that more legislators in favor
only increase the prob. of passing the bill.
e.g. Minority opposition can delay the discussion of the bill
Other theoretical applications
Formation of political parties
Formation of coalition government (structural estimation)
Macroeconomic policies
Scheduling
term paper workshop
Political Economics lecture 5: Legislative Bargaining
By Masayuki Kudamatsu
Political Economics lecture 5: Legislative Bargaining
- 1,867



