1.10 Vectors and Matrices
What are they and why to we care about them ?
Vectors
What are they ?
(c) One Fourth Labs
- Magnitude of a vector
- Direction of a vector
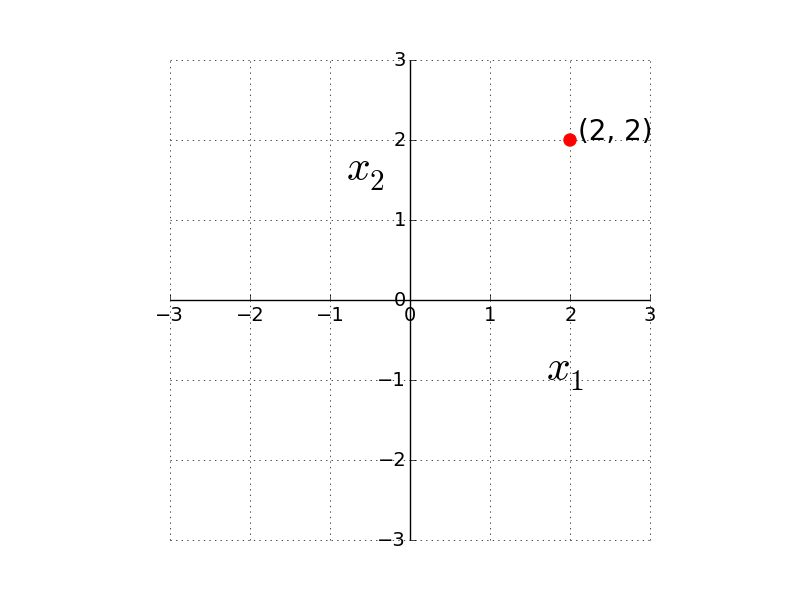
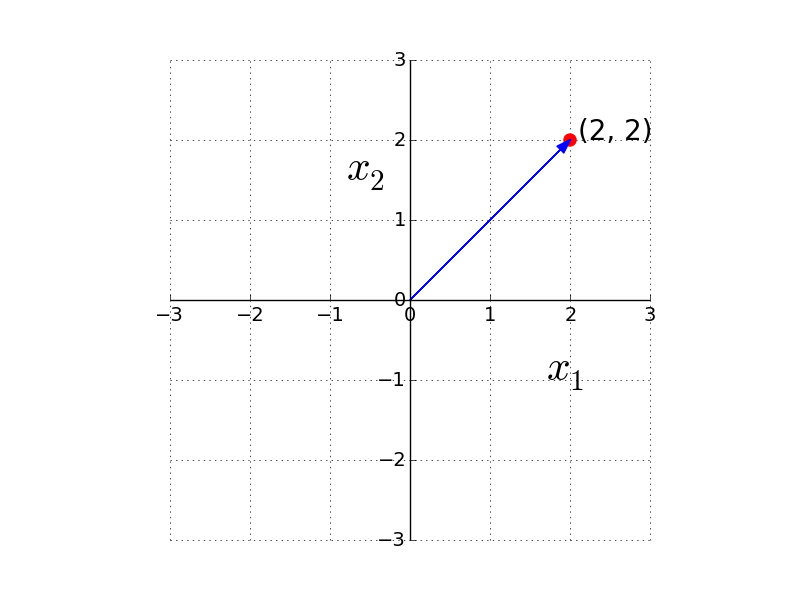
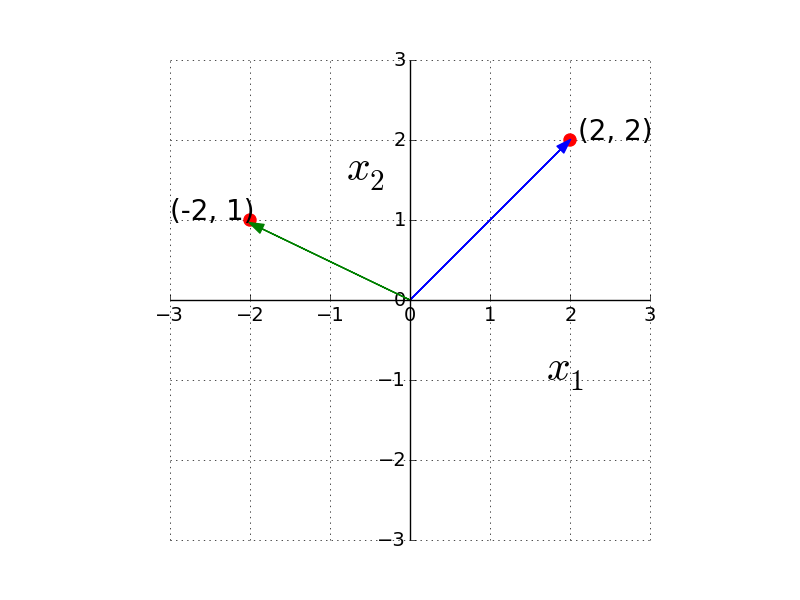
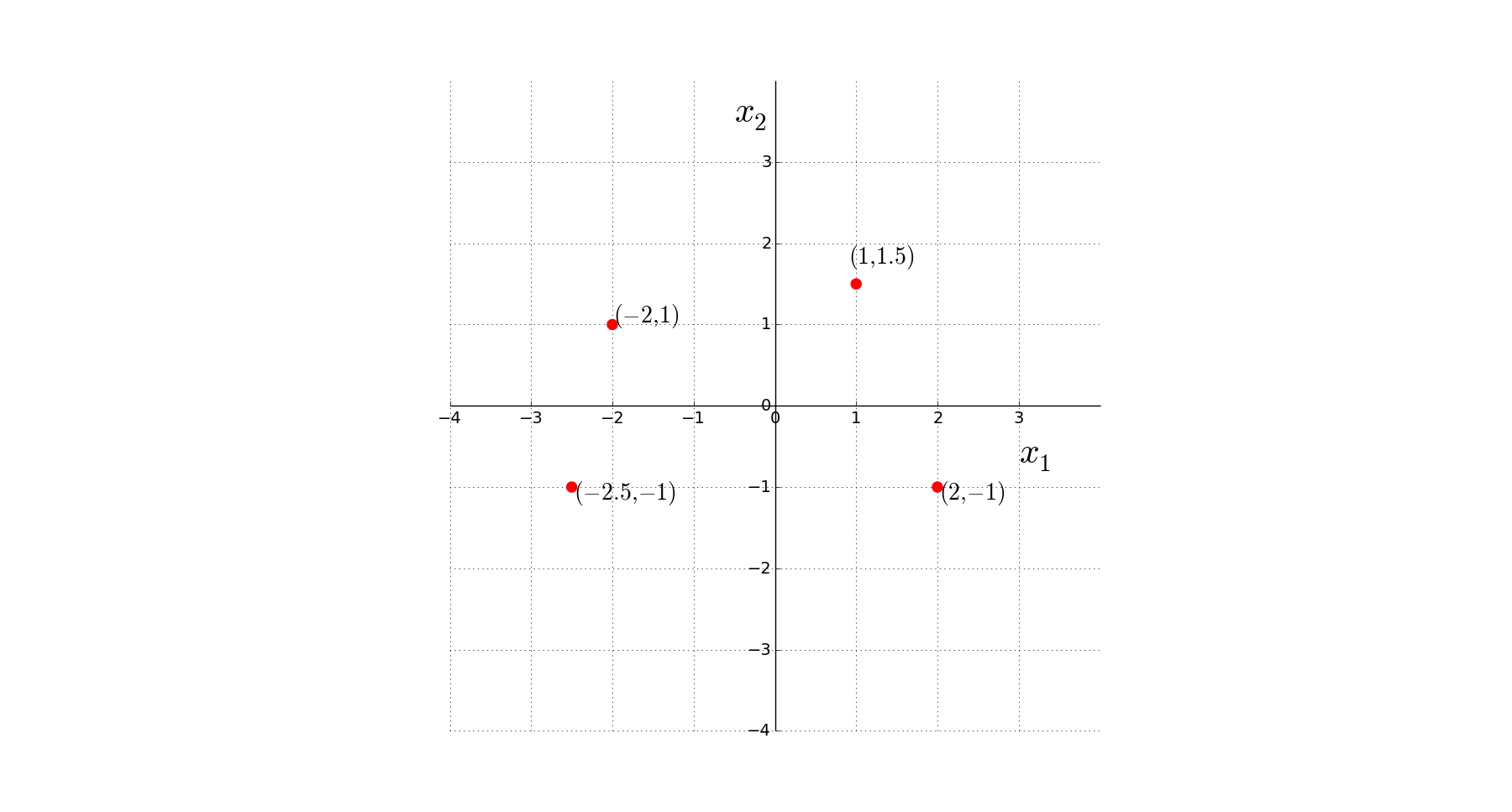
Magnitude of a vector
\sqrt{(x_1^2 + x_2^2)}
\sqrt{2^2 + 2^2} = 2\sqrt{2}
\sqrt{(-2)^2+1^2} = \sqrt{5}
\sqrt{1.5^2+(-2)^2} = 2.5
\sqrt{1^2+1^2} = \sqrt{2}
\sqrt{(-1)^2+1^2} = \sqrt{2}
Magnitude of (2,2)
Magnitude of (-2,1)
Magnitude of (1.5,-2)
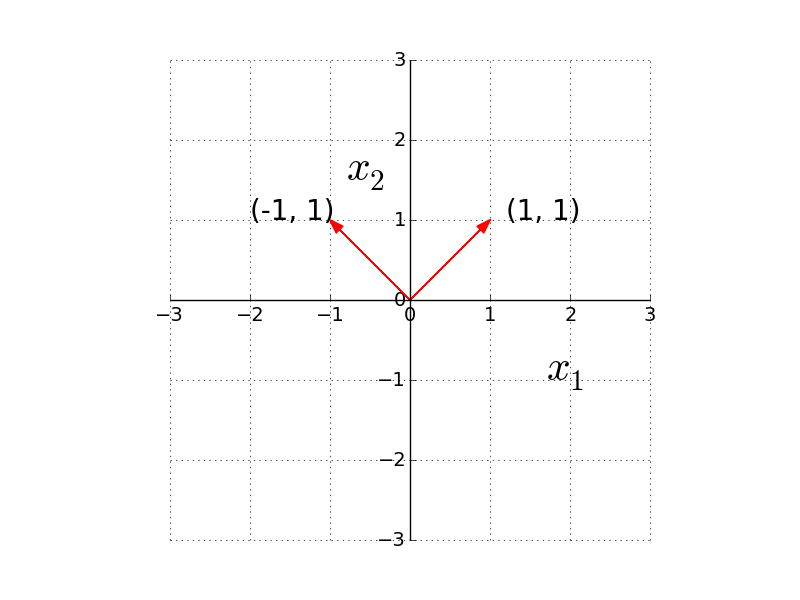
Magnitude of (1,1)
Magnitude of (-1,1)
Vectors
What are they ? (some jargon)
(c) One Fourth Labs
Euclidean Space
In geometry, a two- or three-dimensional space in which the axioms and postulates of Euclidean geometry apply is a Euclidean space. A space in any finite number of dimensions, in which points are designated by coordinates (one for each dimension) and the distance between two points is given by a distance formula.
2D Euclidean Space
3D Euclidean Space
In geometry, a two- or three-dimensional space in which the axioms and postulates of Euclidean geometry apply is a Euclidean space. A space in any finite number of dimensions, in which points are designated by coordinates (one for each dimension) and the distance between two points is given by a distance formula. L2 Norm is also called Euclidean norm.
In geometry, a two- or three-dimensional space in which the axioms and postulates of Euclidean geometry apply is a Euclidean space. A space in any finite number of dimensions, in which points are designated by coordinates (one for each dimension) and the distance between two points is given by a distance formula
In geometry, a two- or three-dimensional space in which the axioms and postulates of Euclidean geometry apply is a Euclidean space. A space in any finite number of dimensions, in which points are designated by coordinates (one for each dimension) and the distance between two points is given by a distance formula
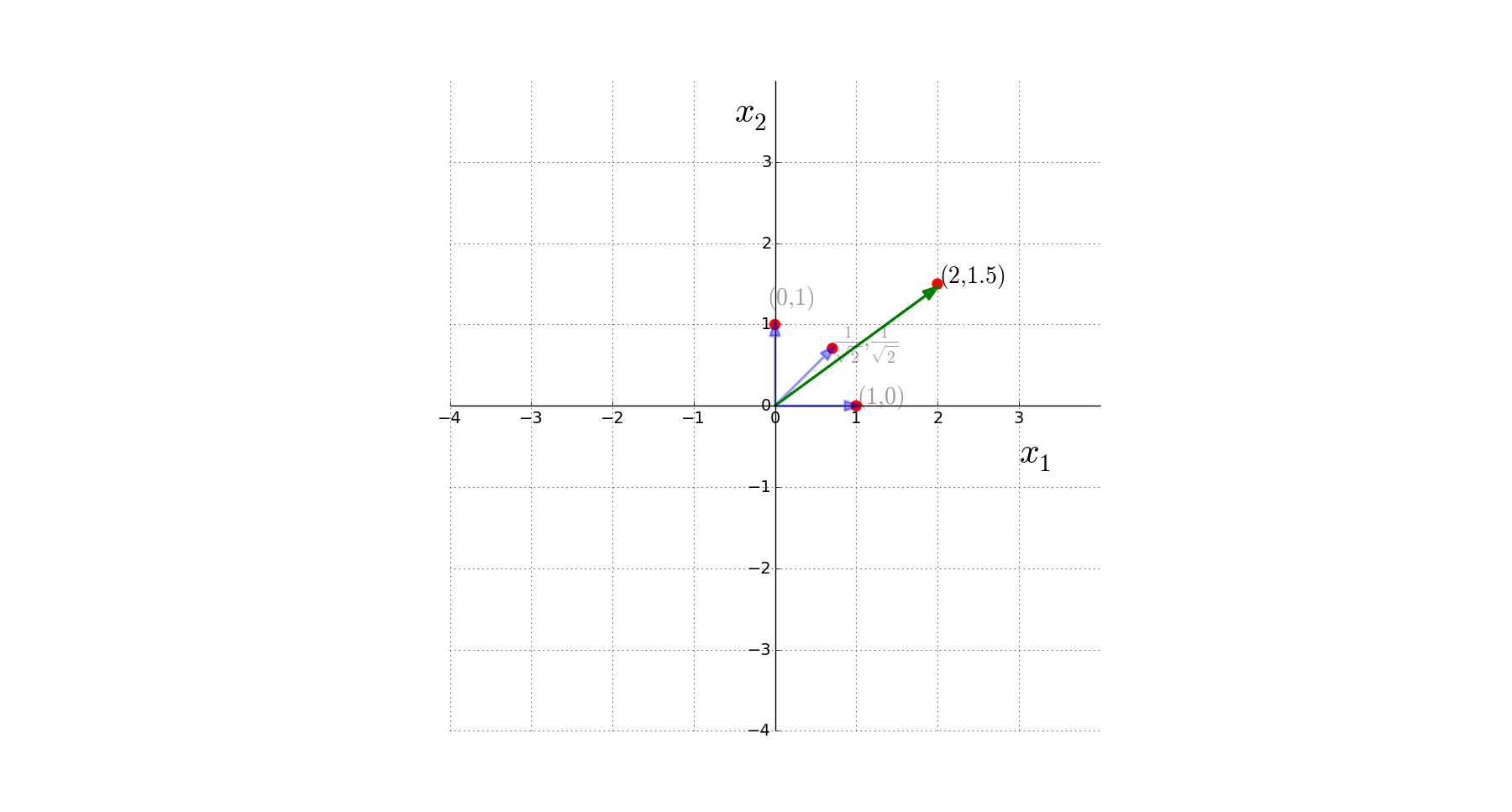
Vectors
How do you add two vectors ?
(c) One Fourth Labs
\begin{bmatrix} -2 \\1 \end{bmatrix} + \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} ? \\? \end{bmatrix}
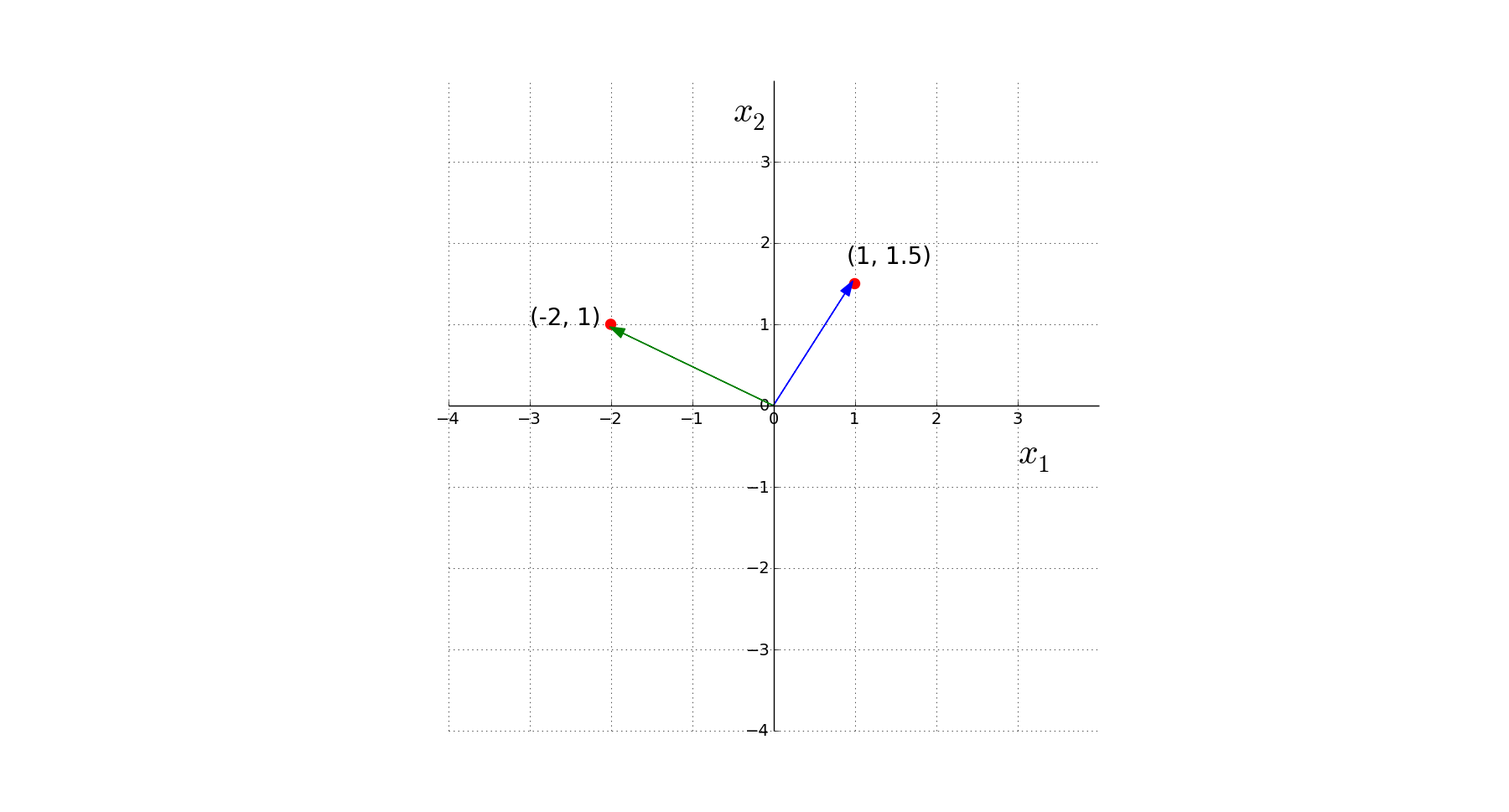
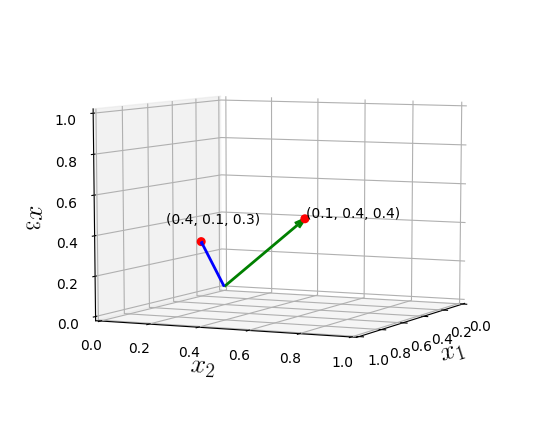
Addition
\begin{bmatrix} 0.1 \\0.4 \\0.4 \end{bmatrix} + \begin{bmatrix} 0.4 \\0.1 \\0.3 \end{bmatrix} = \begin{bmatrix} ? \\? \\? \end{bmatrix}
\begin{bmatrix} -2 \\1 \end{bmatrix} + \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} -1 \\2.5 \end{bmatrix}
\begin{bmatrix} 0.1 \\0.4 \\0.4 \end{bmatrix} + \begin{bmatrix} 0.4 \\0.1 \\0.3 \end{bmatrix} = \begin{bmatrix} 0.5 \\0.5 \\0.7 \end{bmatrix}
\begin{bmatrix} -2 \\1 \end{bmatrix} + \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} -1 \\2.5 \end{bmatrix}
\begin{bmatrix} -2 \\1 \end{bmatrix} + \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} -1 \\2.5 \end{bmatrix}
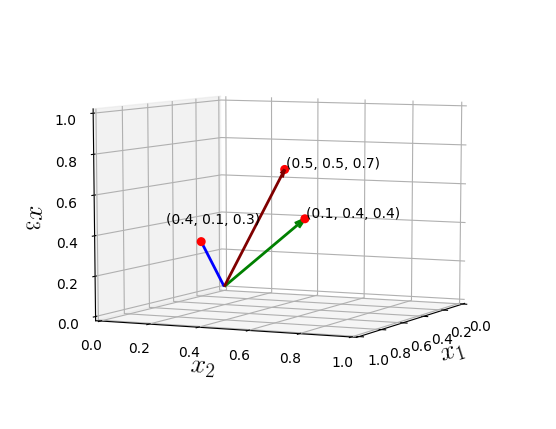
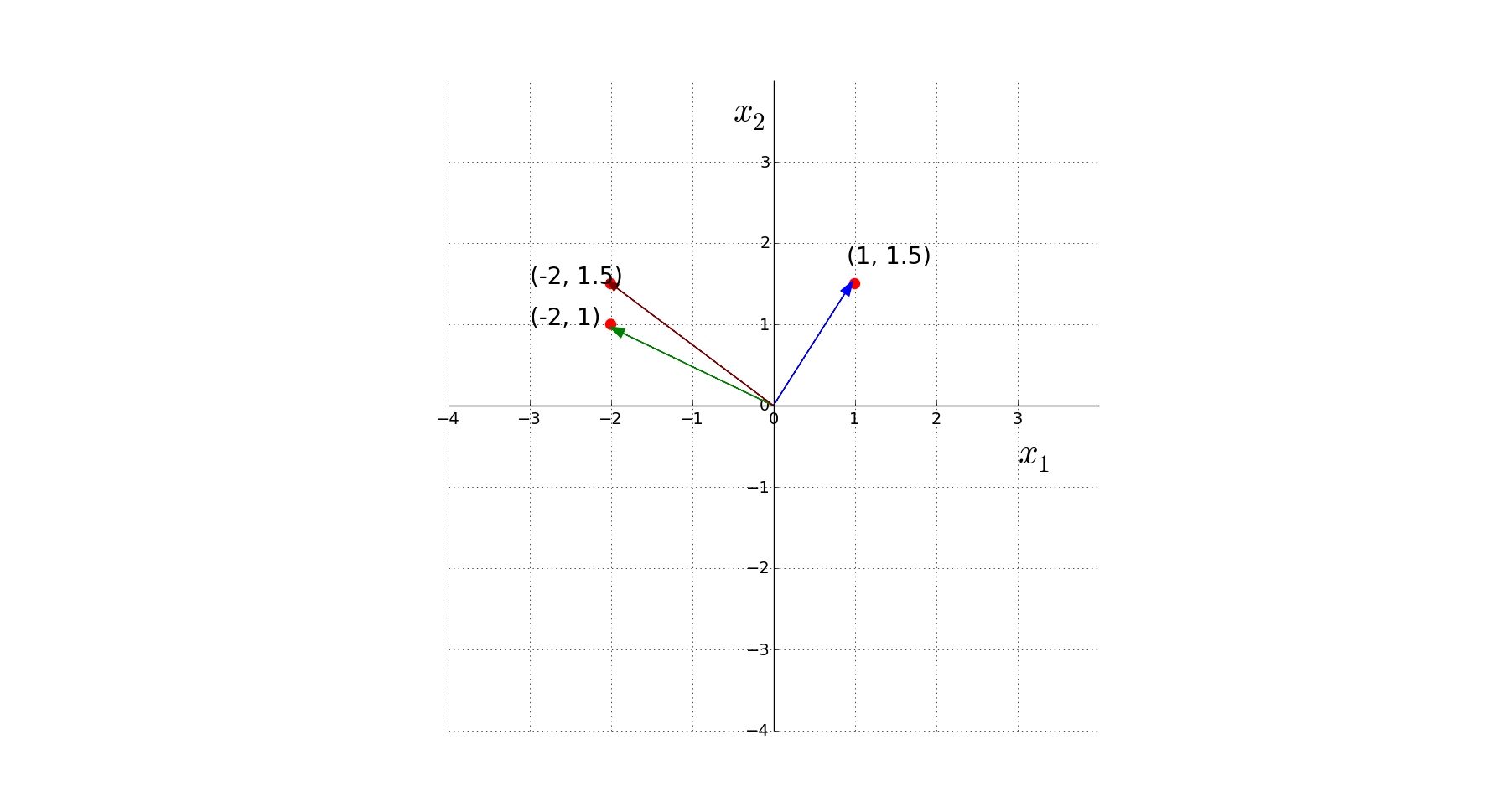
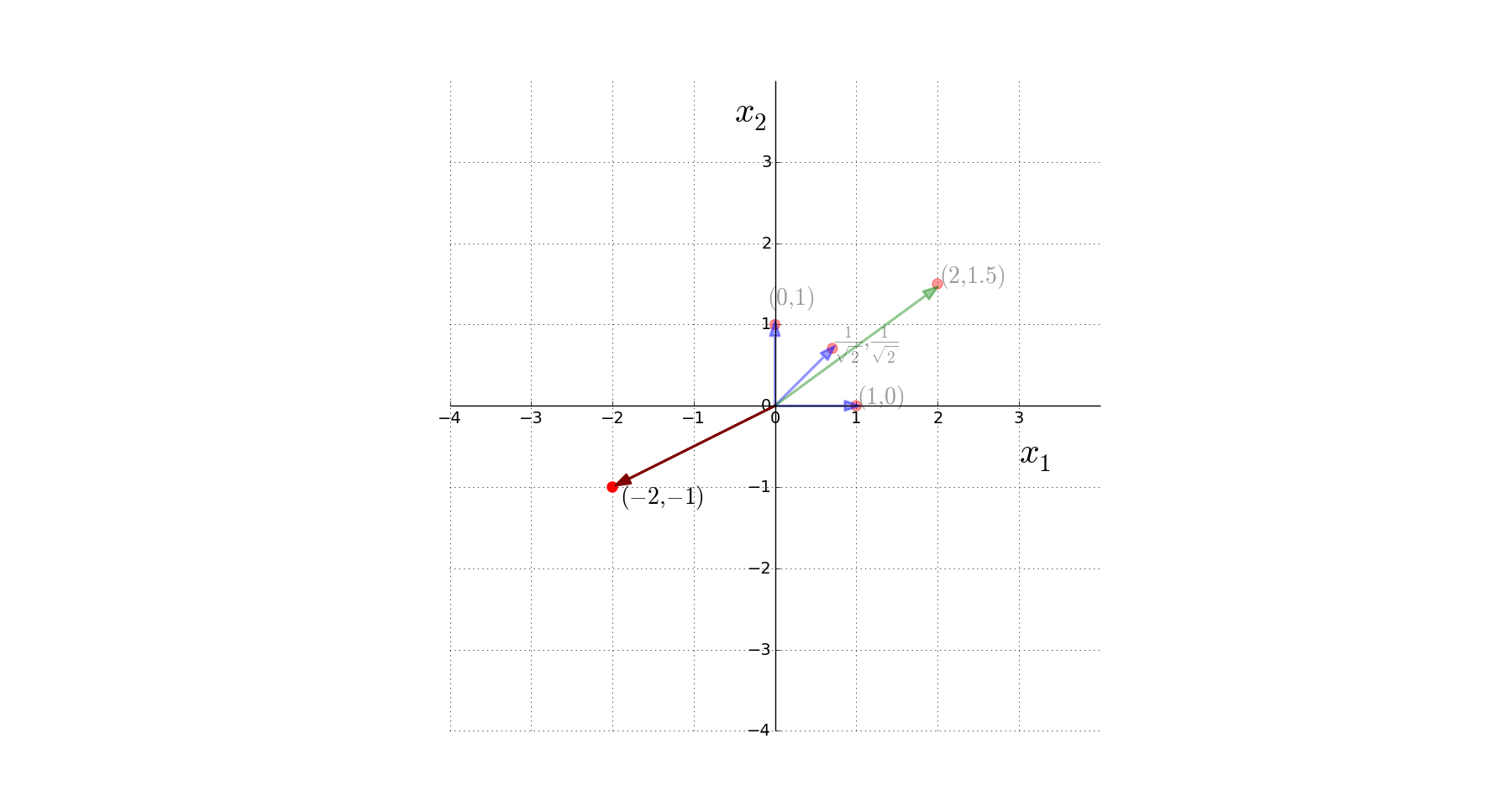
Vectors
How do you subtract two vectors ?
(c) One Fourth Labs
\begin{bmatrix} 1 \\1.5 \end{bmatrix} - \begin{bmatrix} -2 \\1 \end{bmatrix} = \begin{bmatrix} ? \\? \end{bmatrix}
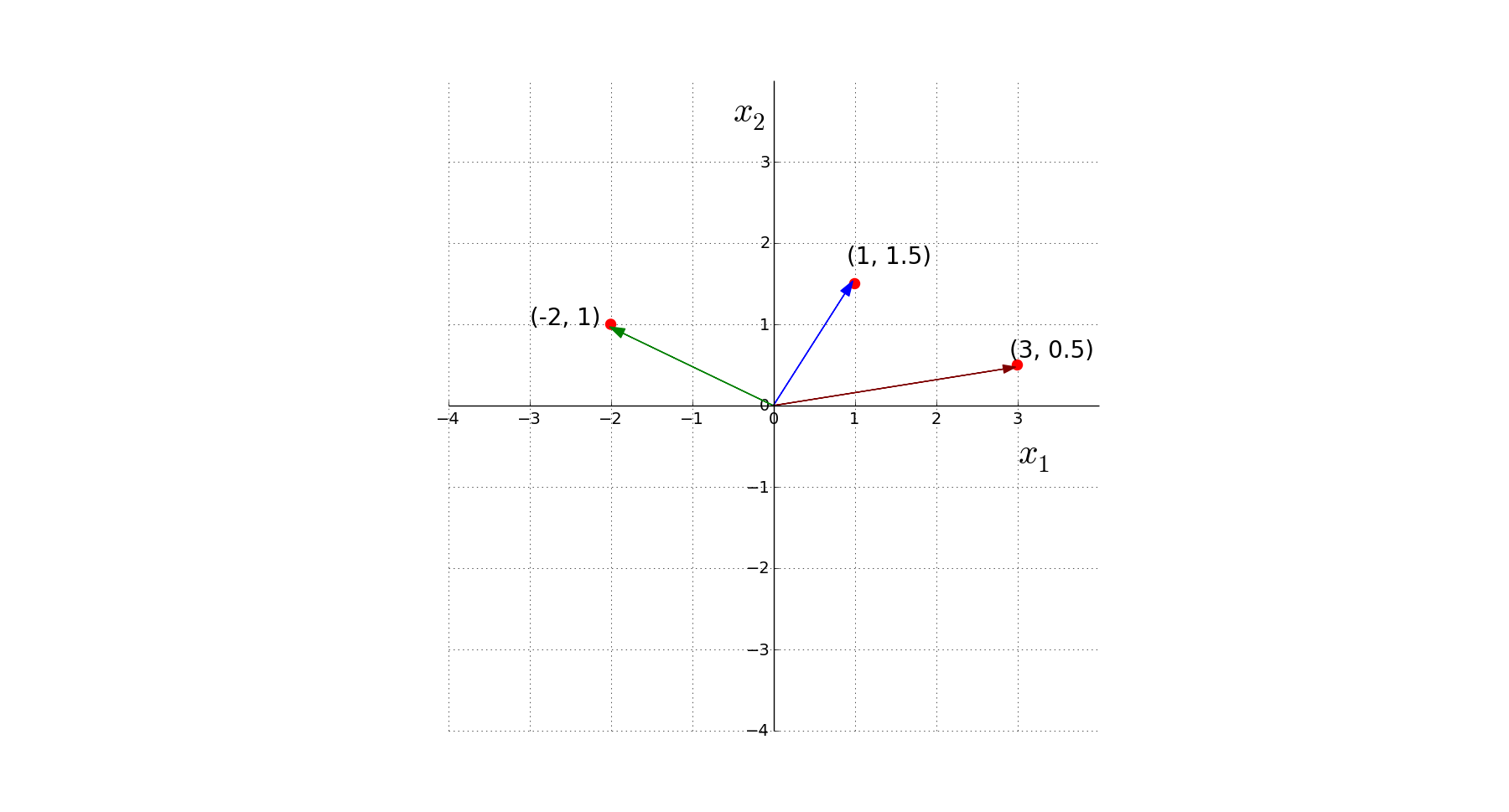
Subtraction
\begin{bmatrix} 0.4 \\0.9 \\0.6 \end{bmatrix} - \begin{bmatrix} 0.1 \\0.2 \\0.3 \end{bmatrix} = \begin{bmatrix} ? \\? \\? \end{bmatrix}
\begin{bmatrix} -2 \\1 \end{bmatrix} - \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} 3 \\0.5 \end{bmatrix}
\begin{bmatrix} 0.4 \\0.9 \\0.6 \end{bmatrix} - \begin{bmatrix} 0.1 \\0.2 \\0.3 \end{bmatrix} = \begin{bmatrix} 0.3 \\0.7 \\0.3\end{bmatrix}
\begin{bmatrix} 1\\1.5 \end{bmatrix} - \begin{bmatrix} -2 \\ 1 \end{bmatrix} = \begin{bmatrix} 3 \\0.5 \end{bmatrix}
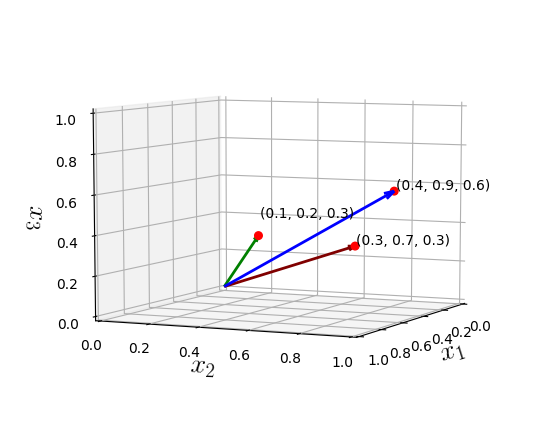
Vectors
How do you multiply two vectors ?
(c) One Fourth Labs
\begin{bmatrix} -2 \\1 \end{bmatrix} . \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} ? \\? \end{bmatrix}
Multiply
\begin{bmatrix} 2.1 \\2.4 \\4.1 \end{bmatrix} . \begin{bmatrix} 1.4 \\2.1 \\1.1 \end{bmatrix} = \begin{bmatrix} ? \\? \\? \end{bmatrix}
\begin{bmatrix} -2 \\1 \end{bmatrix} . \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} -2 \\1.5 \end{bmatrix}
\begin{bmatrix} 2.1 \\2.4 \\4.1 \end{bmatrix} . \begin{bmatrix} 1.4 \\2.1 \\1.1 \end{bmatrix} = \begin{bmatrix} 2.94 \\5.04 \\4.51 \end{bmatrix}
\begin{bmatrix} -2 \\ 1 \end{bmatrix} . \begin{bmatrix} 1 \\1.5 \end{bmatrix} = \begin{bmatrix} -2 \\1.5 \end{bmatrix}
Vectors
How do you project a vector onto another ?
(c) One Fourth Labs
\vec{y}
\vec{x}
proj_{\vec{y}}\vec{x}
proj_{\vec{y}}\vec{x} =
\Big( \ \ \ \ \ \ \ \Big) \vec{y}
\Big(\frac{\vec{x} ⋅ \vec{y}}{||\vec{y}||^2} \Big) \vec{y}
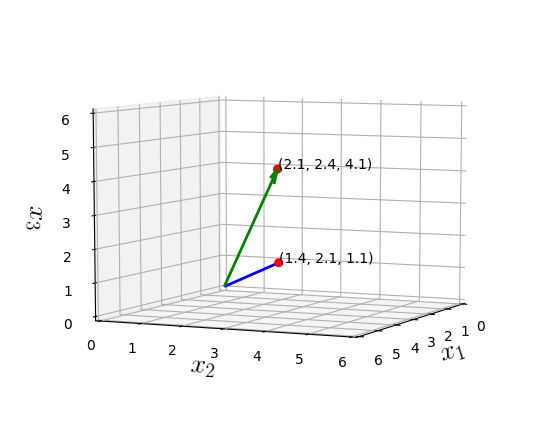
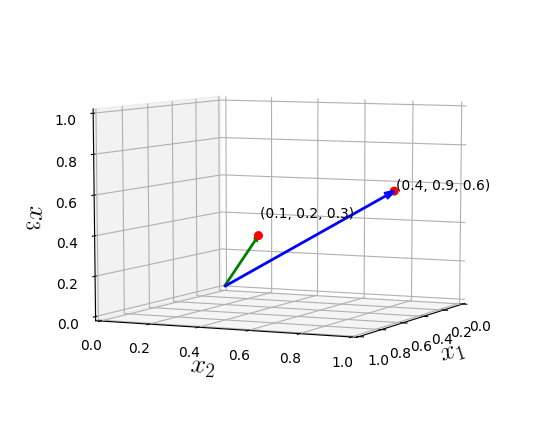
Vectors
How do you project a vector onto another ?
(c) One Fourth Labs
\vec{y}
\vec{x}
proj_{\vec{x}}\vec{y}
proj_{\vec{x}}\vec{y} =
\Big( \ \ \ \ \ \ \ \Big) \vec{x}
\Big(\frac{\vec{x} ⋅ \vec{y}}{||\vec{x}||^2} \Big) \vec{x}
Vectors
How do you project a vector onto another ?
(c) One Fourth Labs
\vec{x} = \begin{bmatrix}
2 \\
2
\end{bmatrix}
\vec{y} = \begin{bmatrix}
0 \\
1
\end{bmatrix}
\vec{x} . \vec{y} = 2 \times 0 + 2 \times 1 = 2
proj_{\vec{y}}\vec{x} =
\Big(\frac{\vec{x} ⋅ \vec{y}}{||\vec{y}||^2} \Big) \vec{y}
||y||^2 = \sqrt{0^2 + 1^2} = 1
= \Big( \frac{2}{1} \Big) \begin{bmatrix}
0 \\
1
\end{bmatrix}
= \begin{bmatrix}
0 \\
2
\end{bmatrix}
?
\vec{x} = \begin{bmatrix}
2 \\
2
\end{bmatrix}
\vec{y} = \begin{bmatrix}
0 \\
1
\end{bmatrix}
Vectors
How do you project a vector onto another ?
(c) One Fourth Labs
proj_{\vec{y}}\vec{x} =
\Big(\frac{\vec{x} ⋅ \vec{y}}{||\vec{y}||^2} \Big) \vec{y}
||y||^2 = \sqrt{2^2 + 2^2 + 1^2} = 3
= \Big( \frac{11}{3} \Big) \begin{bmatrix}
2 \\
2 \\
1
\end{bmatrix}
?
\vec{x}.\vec{y} = 1\times2 + 2\times2 + 5\times1 = 11
\vec{y} =
\begin{bmatrix}
2 \\
2 \\
1
\end{bmatrix}
\vec{x} =
\begin{bmatrix}
1 \\
2 \\
5
\end{bmatrix}
= \begin{bmatrix}
7.33 \\
7.33 \\
3.66
\end{bmatrix}
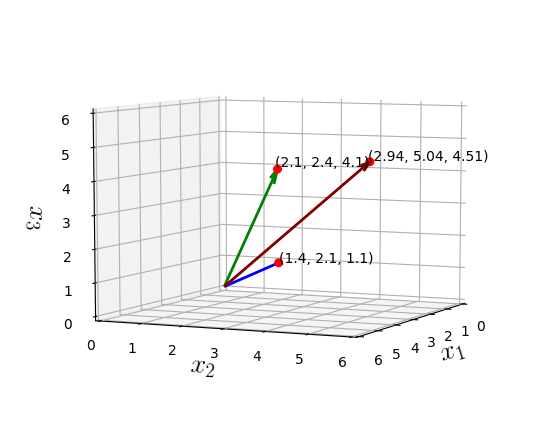
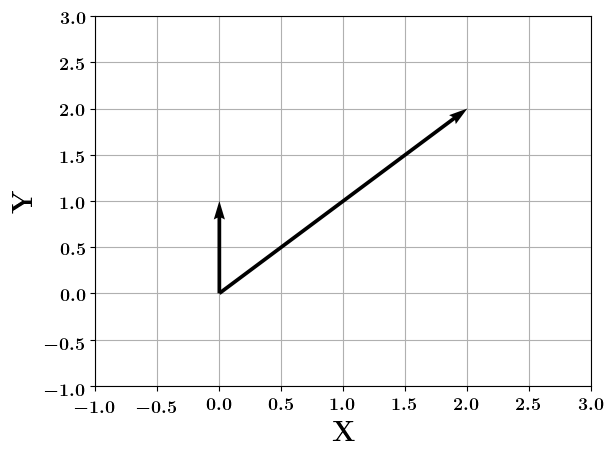
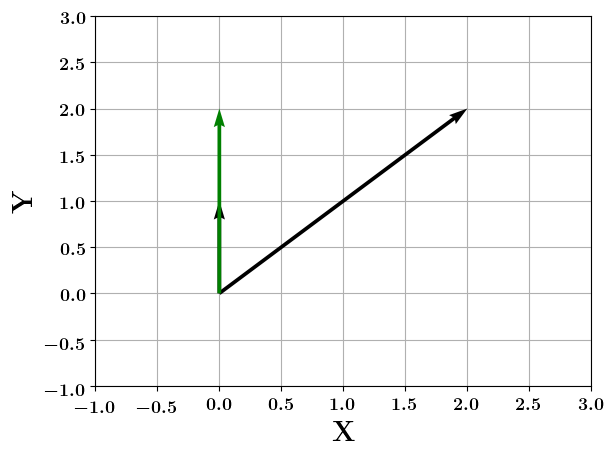
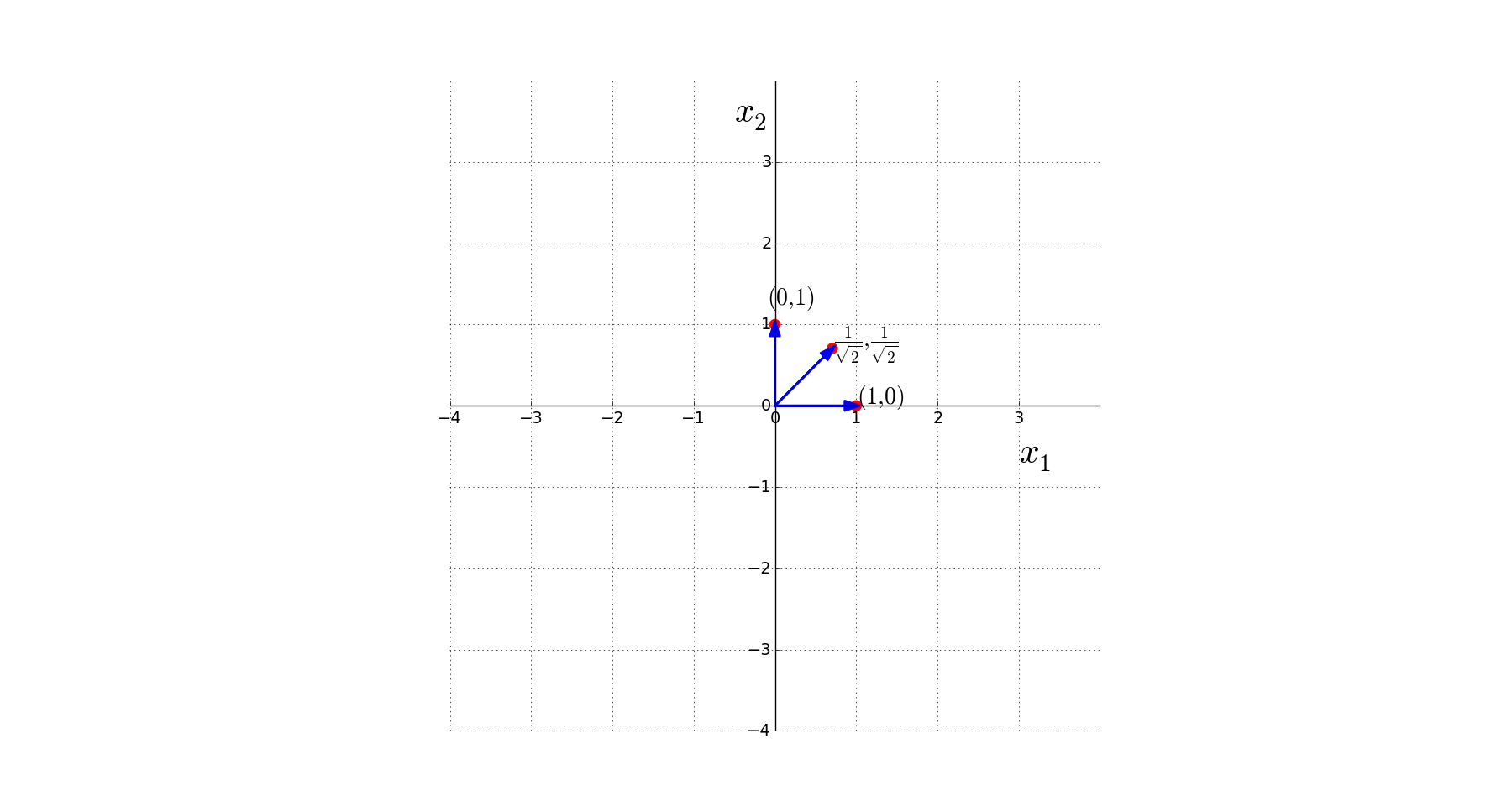
Vectors
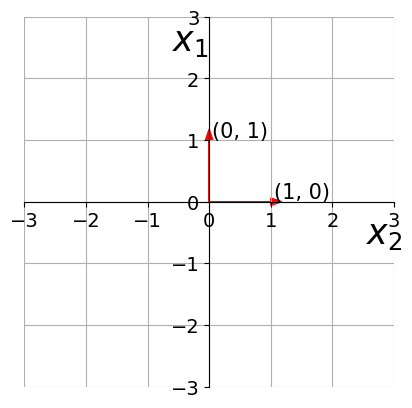
What is a unit vector ?
(c) One Fourth Labs
Any vector whose magnitude is 1?
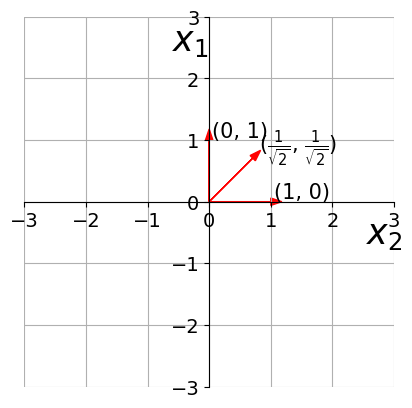
To obtain the unit vector in the direction of a non-unit vector, divide by the magnitude of the given vector.
|| (0, 1) || = \sqrt(0^2 + 1^2) = 1
|| (1, 0) || = \sqrt(1^2 + 0^2) = 1
|| (\frac{1}{\sqrt{2}}, \frac{1}{\sqrt{2}}) || = \sqrt((\frac{1}{\sqrt{2}})^2 + (\frac{1}{\sqrt{2}})^2) = 1
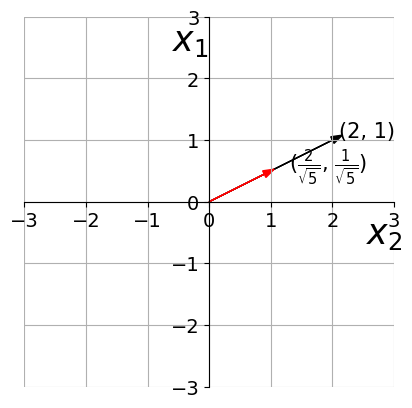
Any vector whose magnitude is 1?
|| (2, 1) || = \sqrt(2^2 + 1^2) = \sqrt5
u(2, 1) = \frac{( 2, 1)}{\sqrt5}
u(2, 1) = (\frac{2}{\sqrt5}, \frac{1}{\sqrt5})
Vectors
How do you compute the angle between two vectors ?
(c) One Fourth Labs
\vec{x} =
\begin{bmatrix}
2 \\
2
\end{bmatrix}
\vec{y} =
\begin{bmatrix}
0 \\
1
\end{bmatrix}
\theta = ?
\vec{x}.\vec{y} = ||\vec{x}||||\vec{y}||cos \theta
\vec{x}.\vec{y} =
2\times0 + 2\times1
= 2
|\vec{x}| =
\sqrt{2^2 + 2^2}
= \sqrt{8}
|\vec{y}| =
\sqrt{0^2 + 1^2}
= \sqrt{1}
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}|| ||\vec{y}||}
\therefore
cos \theta = \frac{2}{\sqrt{8} \sqrt{1}}
cos \theta = \frac{1}{\sqrt{2}}
\theta = 45 \degree
\theta = 45 \degree
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}|| ||\vec{y}||}
\theta = 45 \degree
\vec{y}
\vec{x}
Vectors
How do you compute the angle between two vectors ?
(c) One Fourth Labs
\vec{x} =
\begin{bmatrix}
1 \\
2 \\
5
\end{bmatrix}
\vec{y} =
\begin{bmatrix}
2 \\
2 \\
1
\end{bmatrix}
\theta = ?
\vec{x}.\vec{y} = ||\vec{x}||||\vec{y}||cos \theta
\vec{x}.\vec{y} =
1\times2 + 2\times2 + 5\times1
= 11
|\vec{x}| =
\sqrt{1^2 + 2^2 + 5^2}
= \sqrt{30}
|\vec{y}| =
\sqrt{2^2 + 2^2 + 1^2}
= \sqrt{9}
\therefore
cos \theta = \frac{11}{\sqrt{30} \sqrt{9}}
\theta = cos^{-1} (\frac{11}{\sqrt{9}\sqrt{30}}) \degree
\theta = cos^{-1} (\frac{11}{\sqrt{9}\sqrt{30}}) \degree
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
\theta = cos^{-1} (\frac{11}{\sqrt{9}\sqrt{30}}) \degree
\vec{y}
\vec{x}
Vectors
What are orthogonal vectors?
(c) One Fourth Labs
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
- Orthogonal
\implies \theta = 90 \degree
\implies
Perpendicular
\implies cos \theta = cos(90 \degree)
\implies cos \theta = 0
\implies \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||} = 0
\implies \vec{x}.\vec{y} = 0
Vectors
What are orthogonal vectors?
(c) One Fourth Labs
\vec{x} =
\begin{bmatrix}
1 \\
2
\end{bmatrix}
\vec{y} =
\begin{bmatrix}
2 \\
-1
\end{bmatrix}
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
\vec{x}.\vec{y} =
2 + (-2)
= 0
|\vec{x}| =
\sqrt{1^2 + 2^2}
= \sqrt{5}
|\vec{y}| =
\sqrt{2^2 + (-1)^2}
= \sqrt{5}
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}|| ||\vec{y}||}
\therefore
cos \theta = \frac{0}{\sqrt{5} \sqrt{5}}
cos \theta = 0
\theta = 90 \degree
\theta = 90 \degree
\theta = 90 \degree
\vec{x}
\vec{y}
Vectors
What are orthogonal vectors?
(c) One Fourth Labs
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
\vec{x} =
\begin{bmatrix}
2 \\
3 \\
5
\end{bmatrix}
\vec{y} =
\begin{bmatrix}
1 \\
6 \\
-4
\end{bmatrix}
\vec{x}.\vec{y} =
2 + 18 + (-20)
= 0
|\vec{x}| =
\sqrt{2^2 + 3^2 + 5^2}
= \sqrt{38}
|\vec{y}| =
\sqrt{1^2 + 6^2 + (-4)^2}
= \sqrt{53}
\therefore
cos \theta = \frac{0}{\sqrt{38} \sqrt{53}}
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
\theta = 90 \degree
\theta = 90 \degree
cos \theta = 0
\theta = 90 \degree
\vec{x}
\vec{y}
Vectors
What are orthogonal vectors?
(c) One Fourth Labs
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
\vec{x} =
\begin{bmatrix}
1 \\
2 \\
1 \\
2 \\
3
\end{bmatrix}
\vec{y} =
\begin{bmatrix}
0 \\
1 \\
-2 \\
-3 \\
2
\end{bmatrix}
\vec{x}.\vec{y} =
0 + 2 + (-2) + (-6) + 6
= 0
|\vec{x}| =
\sqrt{1^2 + 2^2 + 1^2 + 2^2 + 3^2}
= \sqrt{19}
|\vec{y}| =
\sqrt{0^2 + 1^2 + (-2)^2 + (-3)^2 + 2^2}
= \sqrt{18}
\therefore
cos \theta = \frac{0}{\sqrt{19} \sqrt{18}}
cos \theta = \frac{\vec{x}.\vec{y}}{||\vec{x}||||\vec{y}||}
\theta = 90 \degree
\theta = 90 \degree
cos \theta = 0
Vectors
What is a unit vector ?
(c) One Fourth Labs
Any vector of magnitude 1 ?"
|| (0, 1) || = \sqrt(0^2 + 1^2) = 1
|| (1, 0) || = \sqrt(1^2 + 0^2) = 1
|| (\frac{1}{\sqrt{2}}, \frac{1}{\sqrt{2}}) || = \sqrt((\frac{1}{\sqrt{2}})^2 + (\frac{1}{\sqrt{2}})^2) = 1
|| (2, 1.5) || = \sqrt(2^2 + (1.5)^2) = \sqrt5.25
u(2, 1.5) = \frac{( 2, 1.5)}{\sqrt5.25}
u(2, 1.5) = (\frac{2}{\sqrt5.25}, \frac{1}{\sqrt5.25})
|| (-2, -1) || = \sqrt((-2)^2 + (-1)^2) = \sqrt5
u(-2, -1) = \frac{(-2, -1)}{\sqrt{5}}
u(-2, -1) = \frac{-2}{\sqrt5} - \frac{1}{\sqrt5}
Vectors
What is a unit vector ?
(c) One Fourth Labs
Any vector of magnitude 1 ?"
|| (0, 1) || = \sqrt(0^2 + 1^2) = 1
|| (1, 0) || = \sqrt(1^2 + 0^2) = 1
|| (\frac{1}{\sqrt{2}}, \frac{1}{\sqrt{2}}) || = \sqrt((\frac{1}{\sqrt{2}})^2 + (\frac{1}{\sqrt{2}})^2) = 1
|| (2, 1.5) || = \sqrt(2^2 + (1.5)^2) = \sqrt5.25
u(2, 1.5) = \frac{( 2, 1.5)}{\sqrt5.25}
u(2, 1.5) = (\frac{2}{\sqrt5.25}, \frac{1}{\sqrt5.25})
|| (-2, -1) || = \sqrt((-2)^2 + (-1)^2) = \sqrt5
u(-2, -1) = \frac{(-2, -1)}{\sqrt{5}}
u(-2, -1) = \frac{-2}{\sqrt5} - \frac{1}{\sqrt5}
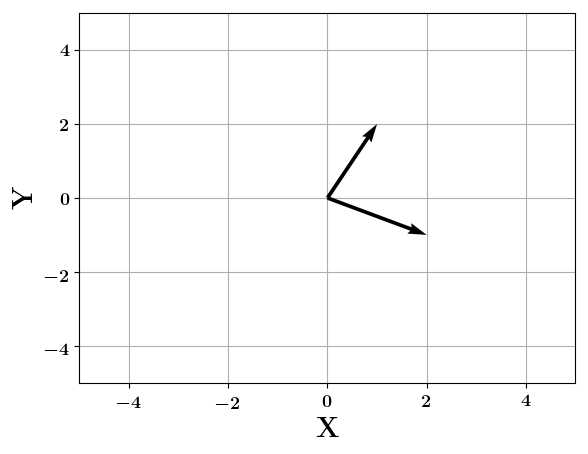
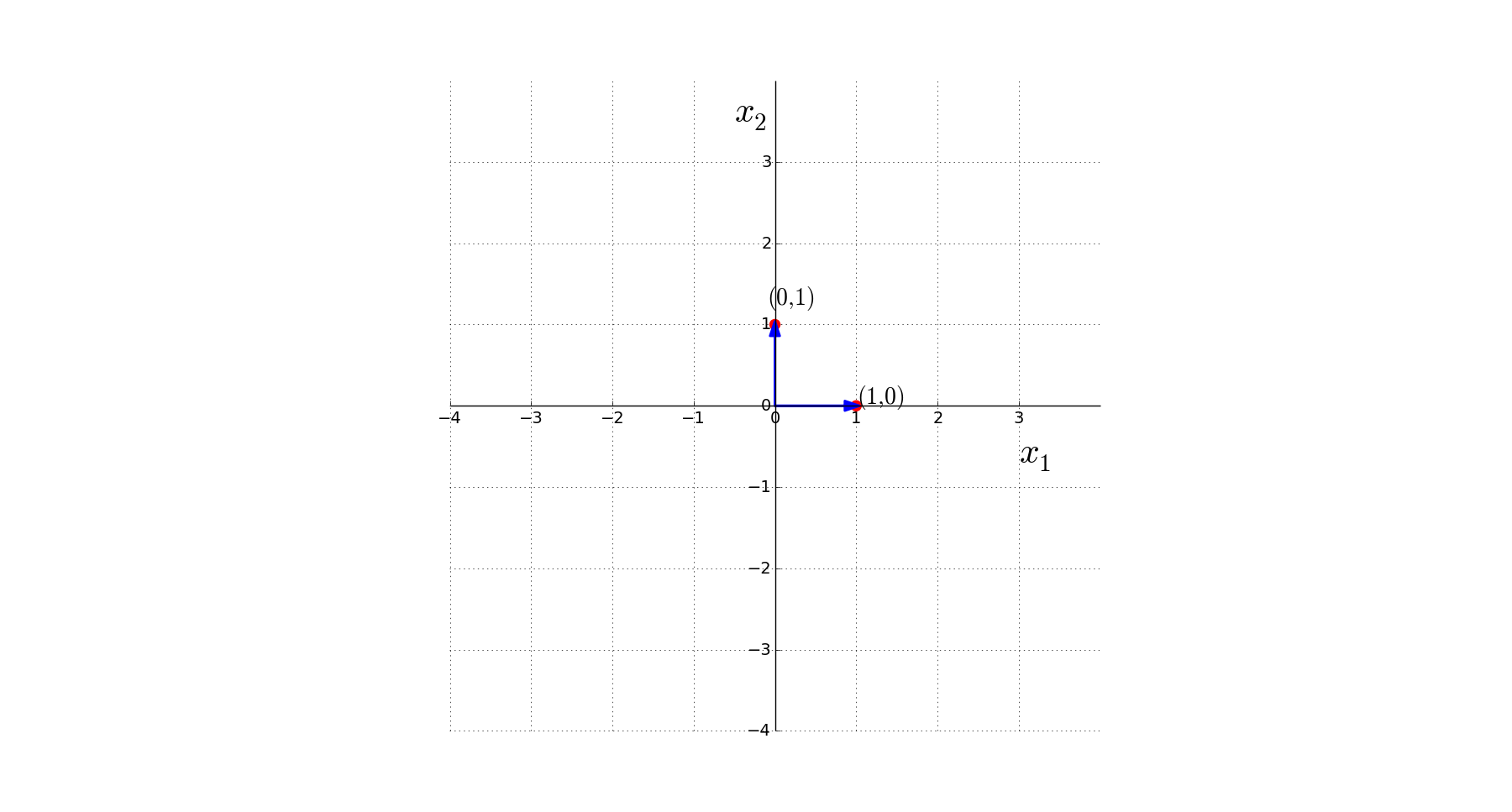
Vectors
Why do we care about them ?
(c) One Fourth Labs
\begin{bmatrix}
151 & 5.8 & 1 & 1 & 3060 & 15000 & 64
\end{bmatrix}
\begin{bmatrix}
165 & 72 & 60000 & 3 & 32 & 5
\end{bmatrix}
\mathbb{R}^{1 \times 7}
\mathbb{R}^{1 \times 6}
\begin{bmatrix}
0 & 1 & 0 & 1 & 1 & 1 & 0 & 0 & 1 & ... & 1
\end{bmatrix}
\mathbb{R}^{1 \times 784}
Matrices
What are they ?
(c) One Fourth Labs
\begin{bmatrix}
1 \\
2 \\
3
\end{bmatrix}
\begin{bmatrix}
1 & 4\\
2 & 5\\
3 & 6
\end{bmatrix}
\begin{bmatrix}
1 & 4 & 7\\
2 & 5 & 8\\
3 & 6 & 9
\end{bmatrix}
column vector
row vector
Matrices
How do you add two matrices ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 4 & 7 \\
2 & 5 & 8 \\
3 & 6 & 9
\end{bmatrix}
\begin{bmatrix}
1 & 2 & 3 \\
4 & 5 & 6 \\
7 & 8 & 9
\end{bmatrix}
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
+
=
\begin{bmatrix}
1+1 & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & 4+2 & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & 6 & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & 6 & 10 \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & 6 & 10 \\
6 & 10 & 14 \\
10 & 14 & 18
\end{bmatrix}
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & 6 & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
2 & 6 & 7+3 \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
Matrices
How do you multiply a matrix with a vector ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 3 \\
2 & 4
\end{bmatrix}
\begin{bmatrix}
5 \\
6
\end{bmatrix}
\times
\begin{bmatrix}
? \\
?
\end{bmatrix}
=
\begin{bmatrix}
? \\
?
\end{bmatrix}
\begin{bmatrix}
? \\
?
\end{bmatrix}
\begin{bmatrix}
(1 \times 5) + \\
?
\end{bmatrix}
\begin{bmatrix}
(1 \times 5) + (3 \times 6) \\
?
\end{bmatrix}
\begin{bmatrix}
5 + 18 \\
?
\end{bmatrix}
\begin{bmatrix}
23 \\
?
\end{bmatrix}
\begin{bmatrix}
23 \\
(2 \times 5) +
\end{bmatrix}
\begin{bmatrix}
23 \\
(2 \times 5) + (4 \times 6)
\end{bmatrix}
\begin{bmatrix}
23 \\
10 + 24
\end{bmatrix}
\begin{bmatrix}
23 \\
34
\end{bmatrix}
\begin{bmatrix}
(1 \times 5) + \\
?
\end{bmatrix}
\begin{bmatrix}
23 \\
?
\end{bmatrix}
\begin{bmatrix}
23 \\
(2 \times 5) +
\end{bmatrix}
Matrices
How do you multiply a matrix with a vector ?
(c) One Fourth Labs
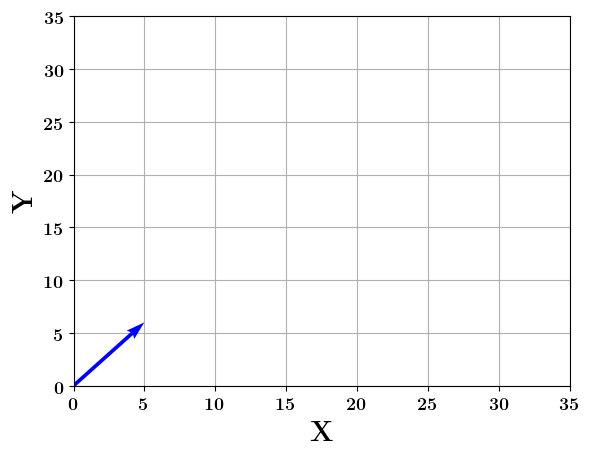
- What happens when a matrix hits a vector?
- The vector gets transformed into a new vector (it strays from its path).
- The vector may also get scaled (elongated or shortened) in the process.
v =
\begin{bmatrix}
5 \\
6 \\
\end{bmatrix}
M =
\begin{bmatrix}
1 & 3 \\
2 & 4 \\
\end{bmatrix}
Mv =
\begin{bmatrix}
23 \\
34 \\
\end{bmatrix}
M =
\begin{bmatrix}
1 & 3 \\
2 & 4 \\
\end{bmatrix}
v =
\begin{bmatrix}
5 \\
6 \\
\end{bmatrix}
Matrices
How do you multiply a matrix with a vector ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\begin{bmatrix}
1 \\
6 \\
5
\end{bmatrix}
\begin{bmatrix}
? \\
? \\
?
\end{bmatrix}
\times
=
\begin{bmatrix}
? \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
(1 \times 1) + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
? \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
1 + (0 \times 6) + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
1 + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
1 + 0 + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
1 + 0 + (3 \times 5)\\
? \\
?
\end{bmatrix}
\begin{bmatrix}
16 \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
16 \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
16\\
(3 \times 1) + \\
?
\end{bmatrix}
\begin{bmatrix}
16\\
3 + \\
?
\end{bmatrix}
\begin{bmatrix}
16\\
3 + (1 \times 6) + \\
?
\end{bmatrix}
\begin{bmatrix}
16\\
3 + 6 +\\
?
\end{bmatrix}
\begin{bmatrix}
16\\
3 + 6 + (1 \times 5) \\
?
\end{bmatrix}
\begin{bmatrix}
16\\
14\\
?
\end{bmatrix}
\begin{bmatrix}
16\\
14\\
?
\end{bmatrix}
\begin{bmatrix}
16\\
14 \\
(0 \times 1) +
\end{bmatrix}
\begin{bmatrix}
16\\
14\\
0+
\end{bmatrix}
\begin{bmatrix}
16\\
14\\
0 + 12 +
\end{bmatrix}
\begin{bmatrix}
16\\
14 \\
0 + (2 \times 6) +
\end{bmatrix}
\begin{bmatrix}
16\\
14 \\
0 + 12 + (5 \times 5)
\end{bmatrix}
\begin{bmatrix}
16\\
14\\
0 + 12 + 25
\end{bmatrix}
\begin{bmatrix}
16 \\
14 \\
37
\end{bmatrix}
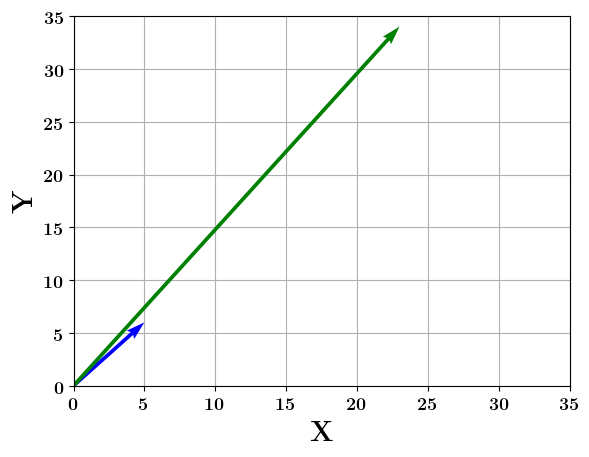
Matrices
How do you multiply a matrix with a vector ?
(c) One Fourth Labs
Mv =
\begin{bmatrix}
16 \\
14 \\
37
\end{bmatrix}
M =
\begin{bmatrix}
1 & 0 & 3\\
3 & 1 & 1\\
0 & 2 & 5\\
\end{bmatrix}
v =
\begin{bmatrix}
1 \\
6 \\
5
\end{bmatrix}
Similarly, in a 3D space, when a vector is hit by a matrix, it gets transformed into a new vector.
Matrices
How do you multiply a matrix with a vector ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\begin{bmatrix}
7 \\
1
\end{bmatrix}
\times
=
\begin{bmatrix}
? \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
? \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
? \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
(1 \times 7) + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
7 + (0 \times 1) + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
7 + \\
? \\
?
\end{bmatrix}
?
\begin{bmatrix}
7 + 0 + \\
? \\
?
\end{bmatrix}
\begin{bmatrix}
7 + 0 + (3 \times ?)\\
? \\
?
\end{bmatrix}
\begin{bmatrix}
7 + 0 + (3 \times ?)\\
? \\
?
\end{bmatrix}
illegal operation
Matrices
How do you multiply a matrix with a vector ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1
\end{bmatrix}
\begin{bmatrix}
7 \\
1 \\
2
\end{bmatrix}
\times
=
\begin{bmatrix}
? \\
?
\end{bmatrix}
\begin{bmatrix}
? \\
?
\end{bmatrix}
\begin{bmatrix}
? \\
?
\end{bmatrix}
\begin{bmatrix}
(1 \times 7) + \\
?
\end{bmatrix}
\begin{bmatrix}
7 + (0 \times 1) + \\
?
\end{bmatrix}
\begin{bmatrix}
7 + \\
?
\end{bmatrix}
\begin{bmatrix}
7 + 0 + \\
?
\end{bmatrix}
\begin{bmatrix}
7 + 0 + (3 \times 2)\\
?
\end{bmatrix}
\begin{bmatrix}
13 \\
?
\end{bmatrix}
\begin{bmatrix}
13 \\
?
\end{bmatrix}
\begin{bmatrix}
13 \\
(3 \times 7) +
\end{bmatrix}
\begin{bmatrix}
13 \\
21 +
\end{bmatrix}
\begin{bmatrix}
13 \\
21 + (1 \times 1) +
\end{bmatrix}
\begin{bmatrix}
13 \\
21 + 1 +
\end{bmatrix}
\begin{bmatrix}
13 \\
21 + 1 + (1 \times 2)
\end{bmatrix}
\begin{bmatrix}
13 \\
24
\end{bmatrix}
legal operation
Conclusion : Number of columns in the matrix should be the same as the number of rows in the vector.
Matrices
How do you multiply two matrices ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\begin{bmatrix}
0 & 1 & 2\\
3 & 0 & 5 \\
1 & 2 & 1
\end{bmatrix}
\times
=
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\times
\begin{bmatrix}
0 \\
3 \\
1
\end{bmatrix}
=
\begin{bmatrix}
3 \\
4 \\
11
\end{bmatrix}
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\times
\begin{bmatrix}
0 \\
3 \\
1
\end{bmatrix}
=
\begin{bmatrix}
3 & ? & ? \\
4 & ? & ? \\
11 & ? & ?
\end{bmatrix}
\begin{bmatrix}
7 \\
5 \\
10
\end{bmatrix}
\begin{bmatrix}
3 & ? & ? \\
4 & ? & ? \\
11 & ? & ?
\end{bmatrix}
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\times
\begin{bmatrix}
1 \\
0 \\
2
\end{bmatrix}
=
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\begin{bmatrix}
1 \\
0 \\
2
\end{bmatrix}
=
\begin{bmatrix}
3 & ? & ? \\
4 & ? & ? \\
11 & ? & ?
\end{bmatrix}
\times
\begin{bmatrix}
3 & ? & ? \\
4 & ? & ? \\
11 & ? & ?
\end{bmatrix}
\begin{bmatrix}
3 & 7 & ? \\
4 & 5 & ? \\
11 & 10 & ?
\end{bmatrix}
\begin{bmatrix}
3 & 7 & ? \\
4 & 5 & ? \\
11 & 10 & ?
\end{bmatrix}
\begin{bmatrix}
3 & 7 & ? \\
4 & 5 & ? \\
11 & 10 & ?
\end{bmatrix}
\begin{bmatrix}
3 & 7 & ? \\
4 & 5 & ? \\
11 & 10 & ?
\end{bmatrix}
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\times
=
\begin{bmatrix}
2 \\
5 \\
1
\end{bmatrix}
\begin{bmatrix}
5 \\
12 \\
15
\end{bmatrix}
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\times
=
\begin{bmatrix}
2 \\
5 \\
1
\end{bmatrix}
\begin{bmatrix}
3 & 7 & 5 \\
4 & 5 & 12 \\
11 & 10 & 15
\end{bmatrix}
Matrices
How do you multiply two matrices ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\begin{bmatrix}
0 & 1 & 2\\
3 & 0 & 1
\end{bmatrix}
\times
=
\begin{bmatrix}
? & ? & ? \\
? & ? & ? \\
? & ? & ?
\end{bmatrix}
Number of columns in the matrix is not equal to the number of rows in the vector.
\begin{bmatrix}
1 & 0 & 3 \\
3 & 1 & 1 \\
0 & 2 & 5
\end{bmatrix}
\begin{bmatrix}
0 \\
3
\end{bmatrix}
\times
=
Matrices
How do you multiply two matrices ?
(c) One Fourth Labs
\begin{bmatrix}
1 & 0 \\
3 & 1 \\
0 & 2
\end{bmatrix}
\begin{bmatrix}
0 & 1 & 2 & 4\\
3 & 0 & 1 & 0
\end{bmatrix}
\times
=
\begin{bmatrix}
? & ? & ? & ? \\
? & ? & ? & ? \\
? & ? & ? & ?
\end{bmatrix}
\begin{bmatrix}
1 & 0 \\
3 & 1 \\
0 & 2
\end{bmatrix}
\times
\begin{bmatrix}
0\\
3
\end{bmatrix}
=
Number of columns in the matrix is equal to the number of rows in the vector.
\begin{bmatrix}
1 & 0 \\
3 & 1 \\
0 & 2
\end{bmatrix}
\times
\begin{bmatrix}
0\\
3
\end{bmatrix}
=
\begin{bmatrix}
1 & 0 \\
3 & 1 \\
0 & 2
\end{bmatrix}
\times
\begin{bmatrix}
0\\
3
\end{bmatrix}
=
\begin{bmatrix}
0 \\
3 \\
6
\end{bmatrix}
\begin{bmatrix}
0 & 1 & 2 & 4 \\
3 & 3 & 7 & 12 \\
6 & 0 & 2 & 0
\end{bmatrix}
\begin{bmatrix}
? & ? & ? & ? \\
? & ? & ? & ? \\
? & ? & ? & ?
\end{bmatrix}
\begin{bmatrix}
? & ? & ? & ? \\
? & ? & ? & ? \\
? & ? & ? & ?
\end{bmatrix}
\begin{bmatrix}
? & ? & ? & ? \\
? & ? & ? & ? \\
? & ? & ? & ?
\end{bmatrix}
\begin{bmatrix}
0 & ? & ? & ? \\
3 & ? & ? & ? \\
6 & ? & ? & ?
\end{bmatrix}
\begin{bmatrix}
? & ? & ? & ? \\
? & ? & ? & ? \\
? & ? & ? & ?
\end{bmatrix}
Matrices
How do you multiply two matrices ?
(c) One Fourth Labs
\begin{bmatrix}
a_{11} & a_{12} & ... & a_{1n} \\
a_{21} & a_{22} & ... & a_{2n} \\
... & ... & ... & ...\\
a_{m1} & a_{m2} & ... & a_{mn}
\end{bmatrix}
\times
=
Conclusion : Any m x n matrix can be multiplied with a n x k matrix to get a m x k output.
\begin{bmatrix}
b_{11} & ... & b_{1k} \\
... & ... & ... \\
b_{n1} & ... & b_{nk}
\end{bmatrix}
\begin{bmatrix}
c_{11} & ... & c_{1k} \\
c_{21} & ... & c_{2k} \\
... & ... & ... \\
c_{m1} & ... & c_{mk}
\end{bmatrix}
Matrices
Is there an alternate way of multiplying matrices ?
(c) One Fourth Labs
\begin{bmatrix}
a_{11} & a_{12} \\
a_{21} & a_{22} \\
\end{bmatrix}
\begin{bmatrix}
b_{11} \\
b_{21} \\
\end{bmatrix}
\times
=
\begin{bmatrix}
a_{11} \times b_{11} + a_{12} \times b_{21} \\
a_{21} \times b_{11} + a_{22} \times b_{21} \\
\end{bmatrix}
\begin{bmatrix}
a_{11} \\
a_{21} \\
\end{bmatrix}
\begin{bmatrix}
a_{12} \\
a_{22} \\
\end{bmatrix}
b_{11}
\times
b_{21}
\times
+
=
Matrices
Is there an alternate way of multiplying matrices ?
(c) One Fourth Labs
\begin{bmatrix}
a_{11} & a_{12} \\
a_{21} & a_{22} \\
\end{bmatrix}
\begin{bmatrix}
b_{11} & b_{12} \\
b_{21} & b_{22} \\
\end{bmatrix}
\times
=
\begin{bmatrix}
a_{11} \times b_{11} + a_{12} \times b_{21} & a_{11} \times b_{12} + a_{12} \times b_{22} \\
a_{21} \times b_{11} + a_{22} \times b_{21} & a_{21} \times b_{12} + a_{22} \times b_{22}
\end{bmatrix}
\begin{bmatrix}
a_{11} \times b_{11} + a_{12} \times b_{21} \\
a_{21} \times b_{11} + a_{22} \times b_{21}
\end{bmatrix}
\begin{bmatrix}
a_{11} \times b_{12} + a_{12} \times b_{22} \\
a_{21} \times b_{12} + a_{22} \times b_{22}
\end{bmatrix}
=
\begin{bmatrix}
a_{11} & a_{12} \\
a_{21} & a_{22} \\
\end{bmatrix}
\begin{bmatrix}
b_{11} \\
b_{21}
\end{bmatrix}
\times
\begin{bmatrix}
a_{11} & a_{12} \\
a_{21} & a_{22} \\
\end{bmatrix}
\begin{bmatrix}
b_{12} \\
b_{22}
\end{bmatrix}
\times
=
\begin{bmatrix}
a_{11} \times b_{11} & a_{11} \times b_{12} \\
a_{21} \times b_{11} & a_{21} \times b_{12}
\end{bmatrix}
\begin{bmatrix}
a_{12} \times b_{21} & a_{12} \times b_{22} \\
a_{22} \times b_{21} & a_{22} \times b_{22}
\end{bmatrix}
=
+
\begin{bmatrix}
a_{11} \\
a_{21}
\end{bmatrix}
\begin{bmatrix}
a_{12} \\
a_{22}
\end{bmatrix}
\begin{bmatrix}
b_{11} & b_{12}
\end{bmatrix}
\begin{bmatrix}
b_{21} & b_{22}
\end{bmatrix}
\times
\times
+
=
Matrices
What is the common operation that you will see in this course ?
(c) One Fourth Labs
W \ \ x \ \ + \ \ b
R^{m \times n}
R^{? \times ?}
R^{? \times ?}
R^{n \times 1}
R^{m \times 1}
| Weight (g) | Screen Size(in.) | Dual SIM | Radio | Battery (mAh) | Price (INR) | . . . | Internal Memory (GB) |
|---|
| 151 | 5.8 | 1 | 1 | 3060 | 15000 | . . . | 64 |
| 180 | 6.18 | 1 | 0 | 3500 | 32000 | . . . | 64 |
| 160 | 5.84 | 0 | 0 | 3060 | 25000 | . . . | 64 |
| 205 | 6.2 | 0 | 1 | 5000 | 18000 | . . . | 64 |
| 162 | 5.9 | 0 | 1 | 3000 | 14000 | . . . | 64 |
| 182 | 6.26 | 1 | 1 | 4000 | 12000 | . . . | 64 |
| 138 | 4.7 | 0 | 0 | 1960 | 35000 | . . . | 64 |
| 185 | 6.41 | 1 | 0 | 3700 | 42000 | . . . | 64 |
| 170 | 5.5 | 0 | 0 | 3260 | 44000 | . . . | 64 |
Why do we care about them ?
(c) One Fourth Labs
Matrices
Why do we care about them ?
(c) One Fourth Labs
Matrices
\begin{bmatrix}
151 & 5.8 & 1 & 1 & 3060 & 15000 & ... & 64 \\
180 & 6.18 & 1 & 0 & 3500 & 32000 & ... & 64 \\
160 & 5.84 & 0 & 0 & 3060 & 25000 & ... & 64 \\
205 & 6.2 & 0 & 1 & 5000 & 18000 & ... & 64 \\
162 & 5.9 & 0 & 1 & 3000 & 14000 & ... & 64 \\
182 & 6.26 & 1 & 1 & 4000 & 12000 & ... & 64 \\
138 & 4.7 & 0 & 0 & 1960 & 35000 & ... & 64 \\
185 & 6.41 & 1 & 0 & 3700 & 42000 & ... & 64 \\
170 & 5.5 & 0 & 0 & 3260 & 44000 & ... & 64 \\
\end{bmatrix}
| F1 | F2 | F3 | F4 | F5 | F6 | ... | Fn |
|---|
Why do we care about them ?
(c) One Fourth Labs
Matrices
| F1 | F2 | F3 | F4 | F5 | F6 | ... | Fn |
|---|
\begin{bmatrix}
0 & 1 & 1 & 0 & 0 & 0 & ... & 0 \\
1 & 0 & 1 & 1 & 0 & 1 & ... & 1 \\
0 & 0 & 0 & 0 & 0 & 0 & ... & 0 \\
0 & 0 & 0 & 1 & 0 & 1 & ... & 1 \\
0 & 1 & 0 & 1 & 1 & 1 & ... & 1 \\
1 & 1 & 1 & 1 & 1 & 0 & ... & 0 \\
0 & 0 & 0 & 0 & 1 & 0 & ... & 1 \\
0 & 1 & 1 & 0 & 0 & 0 & ... & 1 \\
1 & 0 & 0 & 0 & 1 & 0 & ... & 0 \\
0 & 0 & 1 & 1 & 0 & 0 & ... & 0
\end{bmatrix}
Copy of Final Copy of 1.10 Vectors and Matrices
By preksha nema
Copy of Final Copy of 1.10 Vectors and Matrices
matrices
