Chapter 4
\text{Chapter 4}
Chapter 4
\text{Chapter 4}
Chapter 4
\text{Chapter 4}
Chapter 4
\text{Chapter 4}
A particle is accelerated by air over a horizontal xy-plane with a constant acceleration
\text{A particle is accelerated by air over a horizontal $xy$-plane with a constant acceleration}
a= (4.00^+8.00^)m/s2. At time t=0, the velocity is (4.00 m/s)^.
\text{ $\vec{a}=$ $(4.00 \hat{\imath}+8.00 \hat{\jmath}) \mathrm{m} / \mathrm{s}^2$. At time $t=0$, the velocity is $(4.00 \mathrm{~m} / \mathrm{s}) \hat{\imath}$.}
What is the speed of the particle at t=1.65 s ?
\text{ What is the speed of the particle at $t=1.65 \mathrm{~s}$ ?}
A) 16.9 m/sB) 16.2 m/sC) 32.1 m/sD) 20.4 m/sE) 14.6 m/s
\text{A) 16.9 m/s}\\
\text{B) 16.2 m/s}\\
\text{C) 32.1 m/s}\\
\text{D) 20.4 m/s}\\
\text{E) 14.6 m/s}
A particle is accelerated by air over a horizontal xy-plane with a constant acceleration
\text{A particle is accelerated by air over a horizontal $xy$-plane with a constant acceleration}
a= (4.00^+8.00^)m/s2. At time t=0, the velocity is (4.00 m/s)^.
\text{ $\vec{a}=$ $(4.00 \hat{\imath}+8.00 \hat{\jmath}) \mathrm{m} / \mathrm{s}^2$. At time $t=0$, the velocity is $(4.00 \mathrm{~m} / \mathrm{s}) \hat{\imath}$.}
What is the speed of the particle at t=1.65 s ?
\text{ What is the speed of the particle at $t=1.65 \mathrm{~s}$ ?}
A) 16.9 m/sB) 16.2 m/sC) 32.1 m/sD) 20.4 m/sE) 14.6 m/s
\text{\textcolor{red}{A) 16.9 m/s}}\\
\text{B) 16.2 m/s}\\
\text{C) 32.1 m/s}\\
\text{D) 20.4 m/s}\\
\text{E) 14.6 m/s}
vx=vx0+axt
v_x=v_x^0+a_x t
vy=vy0+ayt
v_y=v_y^0+a_y t
vx=4+4.1.65=10.6 m/s
v_x=4+4 . 1.65=10.6 \text{ m/s}
vy=0+81.65=13.2 m/s
v_y=0+8 1.65=13.2 \text{ m/s}
v=vx2+vy2=10.62+13.22=16.9 m/s
\displaystyle v=\sqrt{v_x^2+v_y^2}=\sqrt{10.6^2+13.2^2}=16.9 \text{ m/s}
Motion with constant acceleration along the two axis:
\text{Motion with constant acceleration along the two axis:}
⇒
\Rightarrow
A projectile is fired from level ground with a speed of 12.0 m/s at a target located at a height
\text{A projectile is fired from level ground with a speed of $12.0 \mathrm{~m} / \mathrm{s}$ at a target located at a height }
A) 55.10B) 33.20C) 41.50D) 64.30E) 44.70
\text{\textcolor{black}{A) }}55.1^0\\
\text{B) }33.2^0\\
\text{C) }41.5^0\\
\text{D) }64.3^0\\
\text{E) }44.7^0
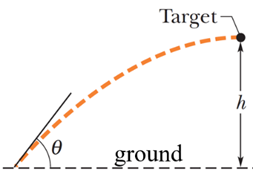
h=5.00 m above the ground as shown in Figure 2. The projectile’s velocity to be horizontal
\text{$h=5.00 \mathrm{~m}$ above the ground as shown in Figure 2. The projectile's velocity to be horizontal}
at the instant it reaches the target.
\text{ at the instant it reaches the target. }
At what angle above the horizontal must the projectile be fired?
\text{At what angle above the horizontal must the projectile be fired?}
A projectile is fired from level ground with a speed of 12.0 m/s at a target located at a height
\text{A projectile is fired from level ground with a speed of $12.0 \mathrm{~m} / \mathrm{s}$ at a target located at a height }
A) 55.10B) 33.20C) 41.50D) 64.30E) 44.70
\text{\textcolor{red}{A) }}55.1^0\\
\text{B) }33.2^0\\
\text{C) }41.5^0\\
\text{D) }64.3^0\\
\text{E) }44.7^0

h=5.00 m above the ground as shown in Figure 2. The projectile’s velocity to be horizontal
\text{$h=5.00 \mathrm{~m}$ above the ground as shown in Figure 2. The projectile's velocity to be horizontal}
at the instant it reaches the target.
\text{ at the instant it reaches the target. }
At what angle above the horizontal must the projectile be fired?
\text{At what angle above the horizontal must the projectile be fired?}
θ=sin−10.82=55.10
\displaystyle \theta=\sin^{-1}0.82=55.1^0
The velocity at the target is horizontal. This means that vy=0
\text{The velocity at the target is horizontal. This means that } v_y=0
We conclude that the target is the maximum height we can reach
\text{We conclude that the target is the \textcolor{red}{maximum height} we can reach}
h=2gv02sin2θ
\displaystyle h=\frac{v_0^2 \sin^2\theta}{2 g}
sinθ=v022gh
\displaystyle \sin\theta=\sqrt{\frac{2 g h}{v_0^2 }}
⇒
\Rightarrow
⇒
\Rightarrow
sinθ=1222×9.8×5=0.82
\displaystyle \sin\theta=\sqrt{\frac{2\times9.8\times5}{12^2 }}=0.82
⇒
\Rightarrow
A ball thrown horizontally at 2.5 m/s travels a horizontal distance of 1.6 m before hitting
\text{A ball thrown horizontally at $2.5 \mathrm{~m} / \mathrm{s}$ travels a horizontal distance of $1.6 \mathrm{~m}$ before hitting }
A) 3.7mB) 4.1mC) 3.2mD) 2.0mE) 1.7m
\text{\textcolor{black}{A) }}3.7\text {m}\\
\text{B) }4.1\text {m}\\
\text{C) }3.2\text {m}\\
\text{D) }2.0\text {m}\\
\text{E) }1.7\text {m}
the ground. From what height was the ball thrown?
\text{the ground. From what height was the ball thrown?}
(old exam)
\text{(old exam)}
A ball thrown horizontally at 2.5 m/s travels a horizontal distance of 1.6 m before hitting
\text{A ball thrown horizontally at $2.5 \mathrm{~m} / \mathrm{s}$ travels a horizontal distance of $1.6 \mathrm{~m}$ before hitting }
A) 3.7mB) 4.1mC) 3.2mD) 2.0mE) 1.7m
\text{\textcolor{black}{A) }}3.7\text {m}\\
\text{B) }4.1\text {m}\\
\text{C) }3.2\text {m}\\
\text{\textcolor{red}{D) }}2.0\text {m}\\
\text{E) }1.7\text {m}
the ground. From what height was the ball thrown?
\text{the ground. From what height was the ball thrown?}
y=xtanθ+21g(v0cosθ)2x2
\displaystyle y=x\tan\theta+\frac{1}{2}g \frac{x^2}{(v_0 \cos\theta)^2}
x0=0,y0=0,θ=0
x_0=0,y_0=0, \theta=0
We choose a reference at the point the object was fired.
\text{We choose a reference at the point the object was fired.}
⇒
\Rightarrow
y=−219.8(2.5)21.62=−2.0 m
\displaystyle y=-\frac{1}{2}9.8 \frac{1.6^2}{(2.5)^2}=-2.0 \text{ m}
The object hits the ground with y=−2.0 m, so the height is 2.0 m
\text{The object hits the ground with }y=-2.0 \text{ m} ,\text{ so the height is } 2.0\text{ m}
(old exam)
\text{(old exam)}
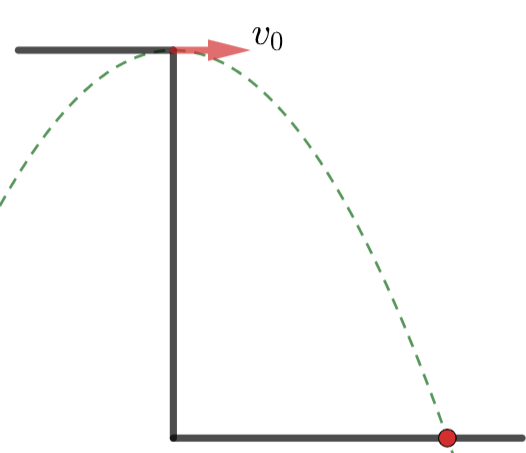
An object falls from a bridge that is 45 m above the water. It falls directly into a small boat,
\text{An object falls from a bridge that is $45 \mathrm{~m}$ above the water. It falls directly into a small boat,}
A)4.0 m/sB) 8.0 m/sC) 6.0 m/sD) 5.0 m/sE) 2.5 m/s
\textcolor{black}{A) \hspace{1mm} 4.0\text { m/s}}\\
\text{B) }8.0\text { m/s}\\
\text{C) }6.0\text { m/s}\\
\text{\textcolor{black}{D) }}5.0\text { m/s}\\
\text{E) }2.5\text { m/s}
(old exam)
\text{(old exam)}
moving with constant speed v as shown in Figure 2. The boat was 12 m away from the point
\text{ moving with constant speed $v$ as shown in Figure 2. The boat was $12 \mathrm{~m}$ away from the point }
of impact (the point at which the object falls on the boat) when the object was released.
\text{of impact (the point at which the object falls on the boat) when the object was released.}
What is the speed v of the boat? [Ignore air resistance.]
\text{ What is the speed $v$ of the boat? [Ignore air resistance.]}

An object falls from a bridge that is 45 m above the water. It falls directly into a small boat,
\text{An object falls from a bridge that is $45 \mathrm{~m}$ above the water. It falls directly into a small boat,}
A)4.0 m/sB) 8.0 m/sC) 6.0 m/sD) 5.0 m/sE) 2.5 m/s
\textcolor{red}{A) \hspace{1mm} 4.0\text { m/s}}\\
\text{B) }8.0\text { m/s}\\
\text{C) }6.0\text { m/s}\\
\text{\textcolor{black}{D) }}5.0\text { m/s}\\
\text{E) }2.5\text { m/s}
(old exam)
\text{(old exam)}
moving with constant speed v as shown in Figure 2. The boat was 12 m away from the point
\text{ moving with constant speed $v$ as shown in Figure 2. The boat was $12 \mathrm{~m}$ away from the point }
of impact (the point at which the object falls on the boat) when the object was released.
\text{of impact (the point at which the object falls on the boat) when the object was released.}
What is the speed v of the boat? [Ignore air resistance.]
\text{ What is the speed $v$ of the boat? [Ignore air resistance.]}

y−y0=v0t+21at2
y-y_0=v_0t+\frac{1}{2}at^2
x−x0=vt
x-x_0=v t
For the ball:
\text{For the ball:}
Let us choose the origin of the frame at the position of the ballon the boat
\text{Let us choose the origin of the frame at the position of the ballon the boat}
⇒
\Rightarrow
0−h=−21gt2
0-h=-\frac{1}{2}gt^2
⇒
\Rightarrow
t=g2h=9.8245=3.0s
\displaystyle t=\sqrt{\frac{2h}{g}}=\frac{2 45}{9.8}=3.0s
For the boat:
\text{For the boat:}
⇒
\Rightarrow
0−x0=vt
0-x_0=v t
v=−x0/t=12/3.0=4.0 m/s
\displaystyle v=-x_0/t=12/3.0=4.0 \text{ m/s}
After flying for 15 min in a wind blowing 44 km/h at an angle of 30∘ south of east, an airplane
\text{After flying for $15 \mathrm{~min}$ in a wind blowing $44 \mathrm{~km} / \mathrm{h}$ at an angle of $30^{\circ}$ south of east, an airplane
}
A) 245 km/hB) 38.1 km/hC) 202 km/hD) 220 km/hE) 44.0 km/h
\textcolor{black}{\text{A) $245 \mathrm{~km} / \mathrm{h}$}}\\
\text{B) $38.1 \mathrm{~km} / \mathrm{h}$}\\
\text{C) $202 \mathrm{~km} / \mathrm{h}$}\\
\text{D) $220 \mathrm{~km} / \mathrm{h}$}\\
\text{E) $44.0 \mathrm{~km} / \mathrm{h}$}
(old exam)
\text{(old exam)}
pilot is over a town that is 55 km due north of the starting point.
\text{pilot is over a town that is $55 \mathrm{~km}$ due north of the starting point.}
What is the speed of the airplane relative to the wind?
\text{ What is the speed of the airplane relative to the wind? }
After flying for 15 min in a wind blowing 44 km/h at an angle of 30∘ south of east, an airplane
\text{After flying for $15 \mathrm{~min}$ in a wind blowing $44 \mathrm{~km} / \mathrm{h}$ at an angle of $30^{\circ}$ south of east, an airplane
}
A) 245 km/hB) 38.1 km/hC) 202 km/hD) 220 km/hE) 44.0 km/h
\textcolor{red}{\text{A) $245 \mathrm{~km} / \mathrm{h}$}}\\
\text{B) $38.1 \mathrm{~km} / \mathrm{h}$}\\
\text{C) $202 \mathrm{~km} / \mathrm{h}$}\\
\text{D) $220 \mathrm{~km} / \mathrm{h}$}\\
\text{E) $44.0 \mathrm{~km} / \mathrm{h}$}
(old exam)
\text{(old exam)}
pilot is over a town that is 55 km due north of the starting point.
\text{pilot is over a town that is $55 \mathrm{~km}$ due north of the starting point.}
What is the speed of the airplane relative to the wind?
\text{ What is the speed of the airplane relative to the wind? }

VPG=VPW+VWG
\vec{V}_\text{PG}=\vec{V}_\text{PW}+\vec{V}_\text{WG}
We project over the x-axis
\text{We project over the x-axis}
VPWx=VPGx−VWGx
{V}_\text{PW}^x={V}_\text{PG}^x-{V}_\text{WG}^x
VPW=VPG−VWG
\vec{V}_\text{PW}=\vec{V}_\text{PG}-\vec{V}_\text{WG}
VPWx=0−44cos(−300)=−223 km/h
{V}_\text{PW}^x=0-44 \cos(-30^0)=-22\sqrt{3} \text{ km/h}
We project over the y-axis
\text{We project over the y-axis}
VPWy=VPGy−VWGy
{V}_\text{PW}^y={V}_\text{PG}^y-{V}_\text{WG}^y
VPWy=220−44sin(−300)=242 km/h
{V}_\text{PW}^y=220-44 \sin(-30^0)=242\text{ km/h}
VPW=(223)2+2422=245 km/h
V_\text{PW}=\sqrt{(22\sqrt{3})^2+242^2}=245 \text{ km/h}
VPG=55 km/0.25h=220 km/h
{V}_\text{PG}=55\text{ km}/0.25\text{h}=220 \text{ km/h}
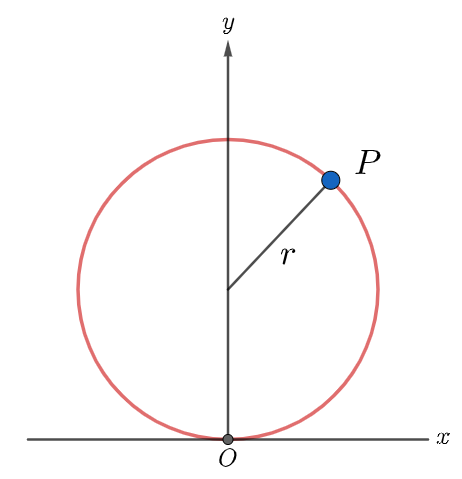
A particle P travels with constant speed on a circle of radius r=3.00 m as shown in Figure 2
\text{A particle $P$ travels with constant speed on a circle of radius $r=3.00 \mathrm{~m}$ as shown in Figure 2 }
and completes one revolution in 20.0 s. The particle passes through O at time t=0.
\text{and completes one revolution in $20.0 \mathrm{~s}$. The particle passes through $O$ at time $t=0$.}
With respect to O, find the particle’s position vector and acceleration at the time t=15 s.
\text{With respect to $O$, find the particle's position vector and acceleration at the time $t=15 \mathrm{~s}$. }
(old exam)
\text{(old exam)}
A particle P travels with constant speed on a circle of radius r=3.00 m as shown in Figure 2
\text{A particle $P$ travels with constant speed on a circle of radius $r=3.00 \mathrm{~m}$ as shown in Figure 2 }
and completes one revolution in 20.0 s. The particle passes through O at time t=0.
\text{and completes one revolution in $20.0 \mathrm{~s}$. The particle passes through $O$ at time $t=0$.}
With respect to O, find the particle’s position vector and acceleration at the time t=15 s.
\text{With respect to $O$, find the particle's position vector and acceleration at the time $t=15 \mathrm{~s}$. }

The distance travelled on the circle is
\text{The distance travelled on the circle is}
d=vt=T2πrt
d=v t =\frac{2\pi r}{T} t
d=rθ
d=r\theta
The length of an arc of circle is:
\text{The length of an arc of circle is:}
θ=2πTt=23π=2700
\displaystyle \theta=2 \pi\frac{t}{T}=\frac{3 \pi}{2}= 270^0
We deduce that:
\text{We deduce that:}
The position is therefore:
\text{The position is therefore:}
x=rcosθ=3.00cos(2700)=−3.00 m
x=r \cos\theta=3.00\cos(270^0)=-3.00\text{ m}
y=rcosθ=3.00sin(2700)=3.00 m
y=r \cos\theta=3.00\sin(270^0)=3.00 \text{ m}
a=rv2=r(2πr/T)2=T2(2π)2r=(2×3.14)2/202×3=0.296 m/s2
\displaystyle a=\frac{v^2}{r}=\frac{(2\pi r/T)^2}{r}=\frac{(2\pi)^2 r}{T^2}=(2\times 3.14)^2/20^2 \times3=0.296 \text{ $m/s^2$}
The particle is at 2700. The acceleration points out towards the center. So,
\text{The particle is at $270^0$. The acceleration points out towards the center. So,}
a=−0.296i^ m/s2
\displaystyle \vec{a}=-0.296 \hspace{1mm}\hat{ i}\text{ $m/s^2$}
r=−3.00i^+3.00j^(m)
\vec{r}=-3.00 \hat{i}+3.00 \hat{j} (m)
(old exam)
\text{(old exam)}
Challenge:
\text{Challenge:}
An object is thrown with a speed v0 with an angle θ from the horizontal. It reaches
\text{An object is thrown with a speed $v_0$ with an angle $\theta$ from the horizontal. It reaches }
a maximum height H=5 m and a range R=10 m
\text{a maximum height $H=5 $ m and a range $R=10 $ m}
Find v0 and θ.
\text{Find $v_0$ and $\theta$.}
H=2gv02sin2θ
\displaystyle H=\frac{v_0^2 \sin^2\theta}{2 g}
R=2gv02sin2θ
\displaystyle R=\frac{v_0^2 \sin2\theta}{2 g}
RH=sin2θsin2θ=2sinθcosθsin2θ=2tanθ
\displaystyle \frac{H}{R}=\frac{\sin^2 \theta}{\sin2\theta}=\frac{\sin^2 \theta}{2\sin\theta\cos\theta}=\frac{\tan\theta}{2}
θ=tan−1(R2H)
\displaystyle \theta=\tan^{-1}\Big(\frac{2H}{R}\Big)
θ=tan−1(102×5)=450
\displaystyle \theta=\tan^{-1}\Big(\frac{2\times 5}{10}\Big)=45^0
⇒
\Rightarrow
⇒
\Rightarrow
⇒
\Rightarrow
An object is thrown with a speed v0 with an angle θ from the horizontal. It reaches
\text{An object is thrown with a speed $v_0$ with an angle $\theta$ from the horizontal. It reaches }
a maximum height H=5 m and a range R=10 m
\text{a maximum height $H=5 $ m and a range $R=10 $ m}
Find v0 and θ.
\text{Find $v_0$ and $\theta$.}
H=2gv02sin2θ
\displaystyle H=\frac{v_0^2 \sin^2\theta}{2 g}
R=2gv02sin2θ
\displaystyle R=\frac{v_0^2 \sin2\theta}{2 g}
RH=sin2θsin2θ=2sinθcosθsin2θ=2tanθ
\displaystyle \frac{H}{R}=\frac{\sin^2 \theta}{\sin2\theta}=\frac{\sin^2 \theta}{2\sin\theta\cos\theta}=\frac{\tan\theta}{2}
θ=tan−1(R2H)
\displaystyle \theta=\tan^{-1}\Big(\frac{2H}{R}\Big)
θ=tan−1(102×5)=450
\displaystyle \theta=\tan^{-1}\Big(\frac{2\times 5}{10}\Big)=45^0
⇒
\Rightarrow
⇒
\Rightarrow
⇒
\Rightarrow
To be updated
\text{To be updated}
C h a p t e r 4 \text{Chapter 4} C h a p t e r 4 \text{Chapter 4} C h a p t e r 4 \text{Chapter 4} C h a p t e r 4 \text{Chapter 4}
Chapter 4-Phys101
By smstry
Chapter 4-Phys101
- 285



