\text{Chapter 15}
\text{Chapter 15}
\text{Chapter 15}
\text{Chapter 15}
\text{A block of mass $2.0 \mathrm{~kg}$ attached to a spring oscillates in simple harmonic motion along the}
\text{$x$ axis. The limits of its motion are $x=-20 \mathrm{~cm}$ and $x=+$ $20 \mathrm{~cm}$ and it goes from one of}
\text{ these extremes to the other in $0.25 \mathrm{~s}$. The mechanical energy of the block-spring system is:}

\text{A $2.0-\mathrm{kg}$ mass connected to a spring of force constant $8.0 \mathrm{~N} / \mathrm{m}$ is displaced $5.0 \mathrm{~cm}$ from}
\text{ its equilibrium position and released. It oscillates on a horizontal, frictionless surface.}
\text{ Find the speed of the mass when it is at $3.0 \mathrm{~cm}$ from its equilibrium position.}

\text{The displacement of a particle moving in simple harmonic motion is given by the equation:}
\text{ $x(t)=2.0 \cos (6.0 t)$, where $x$ is in meters and $t$ is in seconds. }
\text{What is the maximum acceleration of the particle and where does it occur?}

\text{The displacement of a particle moving in simple harmonic motion is given by the equation:}
\text{ $x(t)=2.0 \cos (6.0 t)$, where $x$ is in meters and $t$ is in seconds. }
\text{What is the maximum acceleration of the particle and where does it occur?}
x(t)=2 \cos(6.0 t)
\omega=6 \text{ rad/s}
a(t)=-\omega^2 x(t)
a(t) \text{ will be maximum for } x(t)=-x_m
a(t)=\omega^2 xm=6^2\times 2=72 \text{ $m/s^2$}
\text{ for $x(t)$ minimum}

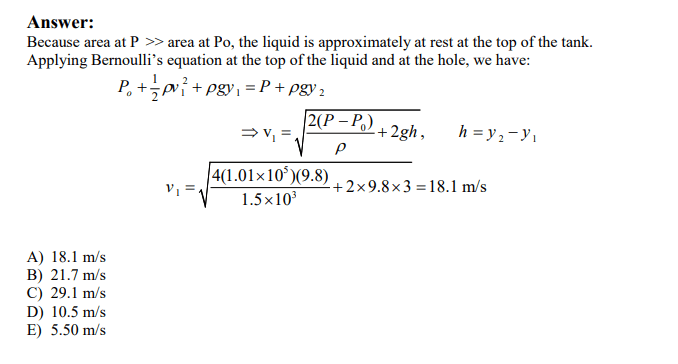

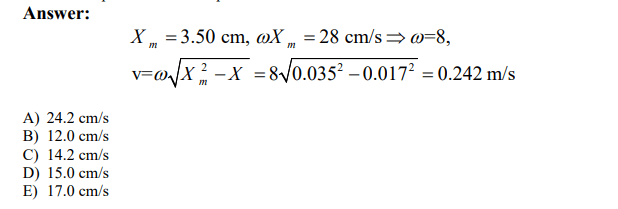


Chapter 15-Phys101
By smstry
Chapter 15-Phys101
- 97



