Chapter 19
\text{Chapter 19}
Chapter 19
\text{Chapter 19}
Chapter 19
\text{Chapter 19}
Chapter 19
\text{Chapter 19}
Dr. Adel Abbout
\text{Dr. Adel Abbout}
The lowest pressure attainable in the laboratory is 5.0×10−18 Pa at 20∘C
\text{The lowest pressure attainable in the laboratory is 5.}0\times10^{-18}\text{ Pa at 20}^{\circ} C
How many gas molecules are there per m3 at this pressure?
\text{How many gas molecules are there per m}^{3}\text{ at this pressure?}
A)1.2×103
A) 1.2 \times 10^3
B)2.3×103
B) 2.3 \times 10^3
C)4.4×103
C) 4.4 \times 10^3
D)3.1×103
D) 3.1 \times 10^3
E)5.6×103
E) 5.6 \times 10^3
PV=NkTVN=kTP=1.38×10−23×(20+273)5×10−18=1.2×103m3 molelule
\begin{aligned}
& P V=N k T \\
& \frac{\mathrm{N}}{\mathrm{V}}=\frac{P}{k T}=\frac{5 \times 10^{-18}}{1.38 \times 10^{-23} \times(20+273)}=1.2 \times 10^3 \frac{\text { molelule }}{\mathrm{m}^3}
\end{aligned}
The lowest pressure attainable in the laboratory is 5.0×10−18 Pa at 20∘C
\text{The lowest pressure attainable in the laboratory is 5.}0\times10^{-18}\text{ Pa at 20}^{\circ} C
How many gas molecules are there per m3 at this(mwessure?
\text{How many gas molecules are there per m}^{3}\text{ at this(mwessure?}
Two moles of a monatomic ideal gas with an RMS speed of 254 m/s are contained in a tank that has a volume of 0.150 m3. If the molar mass of the gas is 0.390 kg/mole, what is the pressure of the gas?
\text{Two moles of a monatomic ideal gas with an RMS speed of 254 m/s are contained in a tank }\\
\hspace{-9.2 cm}\text{that has a volume of 0.150 m}^3.\\
\hspace{-2.2 cm}\text{ If the molar mass of the gas is 0.390 kg/mole, what is the pressure of the gas?}
A) 1.12×105 PaB) 7.17×105 PaC)2.22×104 Pa.D)3.25×106Pa.E) 6.87×104 Pa.
\begin{aligned}
&\text{A) }1.12\times10^5\text{ Pa} \\
&\text{B) }7.17\times10^5\text{ Pa} \\
&\text{C})2.22\times10^4\text{ Pa}. \\
&\text{D})3.25\times10^6\text{Pa.} \\
&\text{E) }6.87\times10^4\text{ Pa}.
\end{aligned}
VrmspVp=M3RT⇒T=3RMVrms2=nRT⇒p=VnRT=VnR3RMVrms2=3VnMVrms2=3(0.150)2(0.390)(254)2=1.12×105 Pa
\begin{aligned}
V_{r m s} & =\sqrt{\frac{3 R T}{M}} \Rightarrow T=\frac{M V_{r m s}^2}{3 R} \\
p V & =n R T \Rightarrow p=\frac{n R T}{V}=\frac{n R}{V} \frac{M V_{r m s}^2}{3 R} \\
p & =\frac{n M V_{r m s}^2}{3 V}=\frac{2(0.390)(254)^2}{3(0.150)}=1.12 \times 10^5 \mathrm{~Pa}
\end{aligned}
Two moles of a monatomic ideal gas with an RMS speed of 254 m/s are contained in a tank that has a volume of 0.150 m3. If the molar mass of the gas is 0.390 kg/mole, what is the pressure of the gas?
\text{Two moles of a monatomic ideal gas with an RMS speed of 254 m/s are contained in a tank }\\
\hspace{-9.2 cm}\text{that has a volume of 0.150 m}^3.\\
\hspace{-2.2 cm}\text{ If the molar mass of the gas is 0.390 kg/mole, what is the pressure of the gas?}
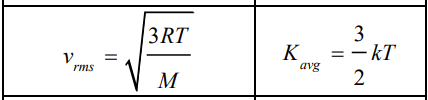
Formula sheet
\text{Formula sheet}
An ideal gas initially at a pressure of 1.2 atm and temperature 74°C undergoes an isothermal expansion to twice its original volume. During the expansion, the gas absorbs 20 kJ of heat.Find the number of moles for this gas?
\begin{aligned}
&\text{An ideal gas initially at a pressure of 1.2 atm and temperature 74°C undergoes an isothermal }\\
&\text{expansion to twice its original volume. During the expansion, the gas absorbs 20 kJ of heat.}\\
&\text{Find the number of moles for this gas?}\end{aligned}
A) 10B) 12C) 15D) 18E) 20
\begin{gathered}
\text{A) 10} \\
\text{B) 12} \\
\text{C) 15} \\
\text{D) 18} \\
\text{E) 20}
\end{gathered}
⇒ΔEint =0=Q−W⇒Q=W=nRTlnViVfn=RTlnViVfQ=(8.31)(74+273)ln220×103=10 moles
\begin{aligned}
& \Rightarrow \Delta E_{\text {int }}=0=Q-W \\
& \Rightarrow Q=W=n R T \ln \frac{V_f}{V_i} \\
& n=\frac{Q}{R T \ln \frac{V_f}{V_i}}=\frac{20 \times 10^3}{(8.31)(74+273) \ln 2}=10 \text { moles }
\end{aligned}
An ideal gas initially at a pressure of 1.2 atm and temperature 74°C undergoes an isothermal expansion to twice its original volume. During the expansion, the gas absorbs 20 kJ of heat.Find the number of moles for this gas?
\begin{aligned}
&\text{An ideal gas initially at a pressure of 1.2 atm and temperature 74°C undergoes an isothermal }\\
&\text{expansion to twice its original volume. During the expansion, the gas absorbs 20 kJ of heat.}\\
&\text{Find the number of moles for this gas?}
\end{aligned}
A) 10B) 12C) 15D) 18E) 20
\begin{gathered}
\text{A) 10} \\
\text{B) 12} \\
\text{C) 15} \\
\text{D) 18} \\
\text{E) 20}
\end{gathered}
Isothermal (ΔT=0)
\text { Isothermal ($\Delta T=0$)}
ΔEint =nCVΔT
\Delta E_{\text {int }}=n C_V \Delta T
(always valid)
\text{(always valid)}
When an amount of heat of 35.1 J was added to a particular ideal gas, the volume of the gaschanged from 50.0 cm3 to 100 cm3 while the pressure remained at 1.00 atm. If the quantity of gas present was 2.00×10−3 mol, find the value of specific heats CV and Cp (in J/mol.K)
\begin{aligned}
&\text{When an amount of heat of 35.1 J was added to a particular ideal gas, the volume of the gas}\\
&\text{changed from $50.0\mathrm{~cm}^3$ to $100\mathrm{~cm}^3$ while the pressure remained at 1.00 atm. If the quantity }\\
&\text{of gas present was $2.00\times10^{-3}$ mol, find the value of specific heats $\mathrm{C}_V$ and $\mathrm{C}_\mathfrak{p}$ (in J/mol.K)}
\end{aligned}
A) 49.5 and 57.8B) 57.8 and 49.5C) 26.1 and 34.4D) 51.1 and 61.5E) 29.5 and 37.8
\text{A) 49.5 and 57.8}\\\text{B) 57.8 and 49.5}\\\text{C) 26.1 and 34.4}\\\text{D) 51.1 and 61.5}\\\text{E) 29.5 and 37.8}
Q=nCpΔT
Q=n C_p \Delta T \\
pV=nRT⇒pΔV=nRΔT
p V=n R T \Rightarrow p \Delta V=n R \Delta T \\
Cp=nΔTQ=pΔVQ=pΔVRQ=(1.01×105)(100−50)×10−6(8.31)(35.1)
C_p=\frac{Q}{n \Delta T}=\frac{Q}{p \Delta V}=\frac{R Q}{p \Delta V}=\frac{(8.31)(35.1)}{\left(1.01 \times 10^5\right)(100-50) \times 10^{-6}} \\
Cp=57.8mol⋅KJCp=CV+R⇒CV=49.4mol⋅KJ
C_p=57.8 \frac{\mathrm{J}}{\mathrm{mol} \cdot K} \quad C_p=C_V+R \Rightarrow C_V=49.4 \frac{\mathrm{J}}{\mathrm{mol} \cdot \mathrm{K}}
When an amount of heat of 35.1 J was added to a particular ideal gas, the volume of the gaschanged from 50.0 cm3 to 100 cm3 while the pressure remained at 1.00 atm. If the quantity of gas present was 2.00×10−3 mol, find the value of specific heats CV and Cp (in J/mol.K)
\begin{aligned}
&\text{When an amount of heat of 35.1 J was added to a particular ideal gas, the volume of the gas}\\
&\text{changed from $50.0\mathrm{~cm}^3$ to $100\mathrm{~cm}^3$ while the pressure remained at 1.00 atm. If the quantity }\\
&\text{of gas present was $2.00\times10^{-3}$ mol, find the value of specific heats $\mathrm{C}_V$ and $\mathrm{C}_\mathfrak{p}$ (in J/mol.K)}
\end{aligned}
Cp=CV+R, so choices B) and D) are not possible
\text{$C_p=C_V+R$, so choices B) and D) are not possible }
(constant pressure)
\text { (constant pressure) }
The figure shows a cycle undergone by 1.0 mole of an ideal diatomic gas. The temperatures are T1=400K,T2=700K,and T3=555K. Calculate the net work done in one cycle.
\begin{aligned}
&\text{The figure shows a cycle undergone by 1.0 mole of an ideal diatomic gas. The temperatures }\\
&\text{are $T_1=400 \mathrm{ K},T_2=700 K$}, \text{and } T_3=555 \mathrm{K}. \text{ Calculate the net~work~done~in~one~cycle}.
\end{aligned}
A) 1.7 kJ by the gasB) 1.7 kJ on the gasC) 3.8 kJ on the gasD) 3.8 kJ by the gasE) 0.52 kJ by the gas
\begin{array}{l}\mathrm{A)~1.7~kJ~by~the~gas}\\\mathrm{B)~1.7~kJ~on~the~gas}\\\mathrm{C)~3.8~kJ~on~the~gas}\\\mathrm{D)~3.8~kJ~by~the~gas}\\\mathrm{E)~0.52~kJ~by~the~gas}\end{array}
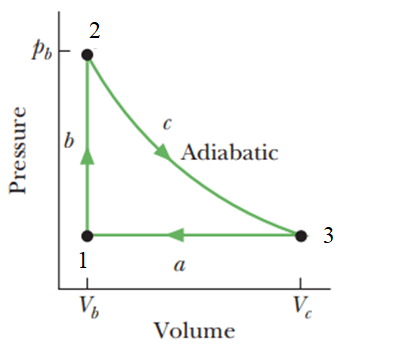
ΔEint=Q−W0=Q−W(cycle)W=Q=Qa+Qb+QcW=nCpΔT31+nCvΔT12+0
\begin{array}{l}{{\Delta E_{int}=Q-W}}\\{0=Q-W\quad(cycle)}\\{{W=Q=Q_{a}+Q_{b}+Q_{c}}}\\{{W=nC_{p}\Delta T_{31}+nC_{v}\Delta T_{12}+0}}\\\end{array}
The figure shows a cycle undergone by 1.0 mole of an ideal diatomic gas. The temperatures are T1=400K,T2=700K,and T3=555K. Calculate the net work done in one cycle.
\begin{aligned}
&\text{The figure shows a cycle undergone by 1.0 mole of an ideal diatomic gas. The temperatures }\\
&\text{are $T_1=400 \mathrm{ K},T_2=700 K$}, \text{and } T_3=555 \mathrm{K}. \text{ Calculate the net~work~done~in~one~cycle}.
\end{aligned}

W=27R(T1−T3)+25R(T2−T1)
W=\frac{7}{2}R(T_{1}-T_{3})+\frac{5}{2}R(T_{2}-T_{1})
W=8.31[27(400−555)+25(700−400)]=+1.7 kJ
W=8.31[\frac{7}{2}(400-555)+\frac{5}{2}(700-400)]=+1.7\text{ kJ}
The work we calculate along the cycle is the work done by the gas
\text{The work we calculate along the cycle is the work done $\underline{\text{by the gas}}$}
answer A
\text{answer A}
An ideal gas with a volume V0 and a pressure P0 undergoes a free expansion to volume V1and pressure P1 where V1=32V0. The gas is then compressed adiabatically to the original volume V0 and pressure 4P0. The ratio of specific heats, γ, of the ideal gas is:
\begin{aligned}
&\text{An ideal gas with a volume }V_0\text{ and a pressure }P_0\text{ undergoes a free expansion to volume }V_1\\
&\text{and pressure } P_1\mathrm{~where~}V_1=32V_0.\text{ The gas is then compressed adiabatically to the original }\\
&\text{volume $V_0$ and pressure } 4\text{P}_0.\text{ The ratio of specific heats, }\gamma,\text{ of the ideal gas is:}
\end{aligned}
A) 7/5B) 2/5C) 3/5D) 1/5E) 9/5
\begin{array}{c}\mathrm{A)~7/5}\\\mathrm{B)~2/5}\\\mathrm{C)~3/5}\\\mathrm{D)~1/5}\\\mathrm{E)~9/5}\end{array}
Reminder for free expansion:ΔE=0,Q=0,W=0,ΔT=0, PV=const
\text{Reminder for free expansion:}\\
\Delta E=0, Q=0,W=0, \Delta T=0,\text{ $PV=$const}
An ideal gas with a volume V0 and a pressure P0 undergoes a free expansion to volume V1and pressure P1 where V1=32V0. The gas is then compressed adiabatically to the original volume V0 and pressure 4P0. The ratio of specific heats, γ, of the ideal gas is:
\begin{aligned}
&\text{An ideal gas with a volume }V_0\text{ and a pressure }P_0\text{ undergoes a free expansion to volume }V_1\\
&\text{and pressure } P_1\mathrm{~where~}V_1=32V_0.\text{ The gas is then compressed adiabatically to the original }\\
&\text{volume $V_0$ and pressure } 4\text{P}_0.\text{ The ratio of specific heats, }\gamma,\text{ of the ideal gas is:}
\end{aligned}
PV=const⇒P1V1=P0V0
PV=\text{const} \Rightarrow P_1V_1=P_0V_0
⇒P1=P0V1V0=P032V0V0=32P0
\Rightarrow P_1=P_0\frac{V_0}{V_1}=P_0\frac{V_0}{32 V_0}=\frac{P_0}{32}
adiabatic expansion:
\text{adiabatic expansion:}
PVγ=const
PV^\gamma=\text{const}
PVγ=const
PV^\gamma=\text{const}
P1V1γ=P2V2γ
P_1V_1^\gamma=P_2V_2^\gamma
P2=4P0
P_2=4P_0
V2=V0
V_2=V_0
32P0(32V0)γ=4P0V0γ⇒3232γ=4⇒γln32=ln128
\frac{P_0}{32}(32 V_0)^\gamma=4P_0 V_0^\gamma\Rightarrow \frac{32^\gamma}{32}=4\Rightarrow \gamma \ln 32=\ln 128
γ=ln32ln128=1.4=7/5
\gamma=\frac{\ln 128}{\ln32}=1.4=7/5
C h a p t e r 1 9 \text{Chapter 19} C h a p t e r 1 9 \text{Chapter 19} C h a p t e r 1 9 \text{Chapter 19} C h a p t e r 1 9 \text{Chapter 19} D r . A d e l A b b o u t \text{Dr. Adel Abbout}
Chapter 19
By smstry
Chapter 19
- 432



