Chapter 16
\text{Chapter 16}
Chapter 16
\text{Chapter 16}
Chapter 16
\text{Chapter 16}
Chapter 16
\text{Chapter 16}
Dr. Adel Abbout
\text{Dr. Adel Abbout}
ym′=2ymcos21ϕ (amplitude).
y_m^{\prime}=\left|2 y_m \cos \frac{1}{2} \phi\right| \quad \text { (amplitude). }
y′(x,t)=[2ymsinkx]cosωt.
y^{\prime}(x, t)=\left[2 y_m \sin k x\right] \cos \omega t .
y′(x,t)=[2ymcos21ϕ]sin(kx−ωt+21ϕ).
y^{\prime}(x, t)=\left[2 y_m \cos \frac{1}{2} \phi\right] \sin \left(k x-\omega t+\frac{1}{2} \phi\right) \text {. }

Pavg=21μνω2ym2
P_{\mathrm{avg}}=\frac{1}{2} \mu \nu \omega^2 y_m^2
v=μτ
v=\sqrt{\frac{\tau}{\mu}}
v=kω=Tλ=λf
v=\frac{\omega}{k}=\frac{\lambda}{T}=\lambda f
k=λ2π
k=\frac{2 \pi}{\lambda}
2πω=f=T1
\frac{\omega}{2 \pi}=f=\frac{1}{T}
y(x,t)=ymsin(kx−ωt)
y(x, t)=y_m \sin (k x-\omega t)
propagating wave
\text{propagating wave}
wave on a stretched string
\text{wave on a stretched string}
wave interference
\text{wave interference}
wave speed doesn’t depend on the frequency
\text{wave speed doesn't depend on the frequency}
standing wave
\text{standing wave}
Resonance
\text{Resonance}
stretched string with fixed ends
\text{stretched string with fixed ends}
f=λv=n2Lv, for n=1,2,3,…
f=\frac{v}{\lambda}=n \frac{v}{2 L}, \quad \text { for } n=1,2,3, \ldots
two waves shifted by Δx have a differnce of phase
\text{two waves shifted by $\Delta x$ have a differnce of phase}
Δϕ=kΔx=2πλΔx
\Delta \phi=k\Delta x=2\pi \frac{\Delta x}{\lambda}
n=1 is the fundamental
\text{$n=1$ is the fundamental}
The displacement of a string carrying a traveling sinusoidal wave is given by:y(x,t)=ymsin(kx−ωt+φ).
\text{The displacement of a string carrying a traveling sinusoidal wave is given by:}\\
y(x, t)=y_m \sin (k x-\omega t+\varphi) .
At time t=0 the point at x=0 has a displacement of zero and is moving in the positive
\text{At time $t=0$ the point at $x=0$ has a displacement of zero and is moving in the positive}
y direction. Find the value of the phase constant φ.
\text{ $y$ direction. Find the value of the phase constant $\varphi$.}
A) 180 degreesB) 90 degreesC) 135 degreesD) 0 degreesE) 270 degrees
\begin{aligned}
&\text{A) 180 degrees} \\
&\text{B) 90 degrees} \\
&\text{C) 135 degrees} \\
&\text{D) 0 degrees} \\
&\text{E) 270 degrees}
\end{aligned}
The displacement of a string carrying a traveling sinusoidal wave is given by:y(x,t)=ymsin(kx−ωt+φ).
\text{The displacement of a string carrying a traveling sinusoidal wave is given by:}\\
y(x, t)=y_m \sin (k x-\omega t+\varphi) .
At time t=0 the point at x=0 has a displacement of zero and is moving in the positive
\text{At time $t=0$ the point at $x=0$ has a displacement of zero and is moving in the positive}
y direction. Find the value of the phase constant φ.
\text{ $y$ direction. Find the value of the phase constant $\varphi$.}
y=ymsinϕ=0⇒sinϕ=0
\displaystyle y=y_m \sin\phi=0\Rightarrow \sin \phi=0
at x=0, t=0, we have
\text{at $x=0$, $t=0$, we have }
⇒ϕ={0π
\Rightarrow
\phi = \begin{cases}
0 \\
\pi
\end{cases}
u(0,0)=−ωymcosϕ>0⇒cosϕ<0
\displaystyle u(0,0)=-\omega y_m \cos\phi>0 \Rightarrow \cos \phi<0
We deduce that: ϕ=π
\text{We deduce that: $\phi=\pi$}
Reminder:
\text{Reminder:}
The equation sinθ=a
\text{The equation } \sin\theta=a
has two solutions in [0,2π]:
\text{has two solutions in } [0, 2\pi]:
θ=θ0
\theta=\theta_0
θ=π−θ0
\theta=\pi-\theta_0
where θ0=sin−1a
\text{where } \theta_0=\sin^{-1}a
Your calculator gives only one of them
\text{Your calculator gives only one of them}
(We use the transverse speed to find which angle is correct)
\text{(We use the transverse speed to find which angle is correct)}
(moving upward, ⇒u>0)
\text{(moving upward, $\Rightarrow u>0$)}
A transverse sinusoidal wave is travelling on a stretched string. The maximum transverse
\text{A transverse sinusoidal wave is travelling on a stretched string. The maximum transverse }
speed of a particle on the string is 24.0 m/s.
\text{speed of a particle on the string is $24.0 \mathrm{~m} / \mathrm{s}$.}
The frequency of oscillations of a particle
\text{The frequency of oscillations of a particle }
in the string is 120 Hz. What is the amplitude of the wave?
\text{in the string is $120 \mathrm{~Hz}$. What is the amplitude of the wave?}
A) 31.8 mm
\text{A) $31.8 \mathrm{~mm}$}
B) 25.1 mm
\text{B) $25.1 \mathrm{~mm}$}
C) 12.0 mm
\text{C) $12.0 \mathrm{~mm}$}
D) 43.3 mm
\text{D) $43.3 \mathrm{~mm}$}
E) 53.2 mm
\text{E) $53.2 \mathrm{~mm}$}
A transverse sinusoidal wave is travelling on a stretched string. The maximum transverse
\text{A transverse sinusoidal wave is travelling on a stretched string. The maximum transverse }
speed of a particle on the string is 24.0 m/s.
\text{speed of a particle on the string is $24.0 \mathrm{~m} / \mathrm{s}$.}
The frequency of oscillations of a particle
\text{The frequency of oscillations of a particle }
in the string is 120 Hz. What is the amplitude of the wave?
\text{in the string is $120 \mathrm{~Hz}$. What is the amplitude of the wave?}
um=ωym⇒ym=ωum=2πfum=2π×12024=0.0318m
u_m=\omega y_m \Rightarrow y_m=\frac{u_m}{\omega}=\frac{u_m}{2\pi f}=\frac{24}{2 \pi\times 120}=0.0318\hspace{1mm} m
The maximum transverse speed is
\text{The maximum transverse speed is}
Figure 1 shows a snapshot graph of a wave traveling to the right along a string at 25 m/s.
\text{Figure 1 shows a snapshot graph of a wave traveling to the right along a string at 25 $\mathrm{m} / \mathrm{s}$.}
At this instant, what are the velocities of points A, B , and C on the string, respectively?
\text{At this instant, what are the velocities of points A, B , and C on the string, respectively?}
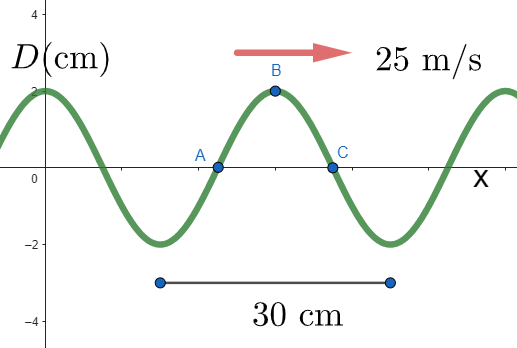
A) −11 m/s,0,+11 m/s
\text{A) $-11 \mathrm{~m} / \mathrm{s}, 0,+11 \mathrm{~m} / \mathrm{s}$}
B) −11 m/s,0,−11 m/s
\text{B) $-11 \mathrm{~m} / \mathrm{s}, 0,-11 \mathrm{~m} / \mathrm{s}$}
C) 0,−11 m/s,0
\text{C) $0,-11 \mathrm{~m} / \mathrm{s}, 0$}
D) 0,+11 m/s,−11 m/s
\text{D) $0,+11 \mathrm{~m} / \mathrm{s},-11 \mathrm{~m} / \mathrm{s}$}
E) −19 m/s,0,+19 m/s
\text{E) $-19 \mathrm{~m} / \mathrm{s}, 0,+19 \mathrm{~m} / \mathrm{s}$}
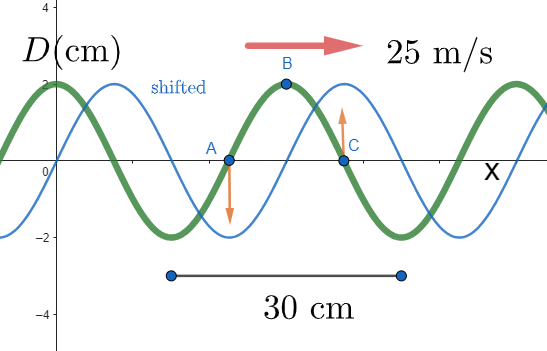
We shift the wave to the right
\text{We shift the wave to the \textcolor{red}{right}}
(because it is a right moving wave)
\text{(because it is a right moving wave)}
We check in which direction each point moves
\text{We check in which direction each point moves}
A: down, C: Up
\text{A: down, C: Up}
∣umax∣=ωym=2πfym
\left|u_{\max }\right|=\omega y_m=2 \pi f y_m
f=λv=0.325=83.33 m/s
f=\frac{v}{\lambda}=\frac{25}{0.3}=83.33 \mathrm{~m} / \mathrm{s}
∣umax∣=2π×83.33×2×10−2=10.47 m/s≈11 m/s
\left|u_{\max }\right|=2 \pi \times 83.33 \times 2 \times 10^{-2}=10.47 \mathrm{~m} / \mathrm{s}\approx11 \mathrm{~m} / \mathrm{s}
Figure 1 shows a snapshot graph of a wave traveling to the right along a string at 25 m/s.
\text{Figure 1 shows a snapshot graph of a wave traveling to the right along a string at 25 $\mathrm{m} / \mathrm{s}$.}
At this instant, what are the velocities of points A, B , and C on the string, respectively?
\text{At this instant, what are the velocities of points A, B , and C on the string, respectively?}
At B, the transverse speed=0
\text{At B, the transverse speed=0}
At A and C, the transverse speed is maximum
\text{At A and C, the transverse speed is maximum}
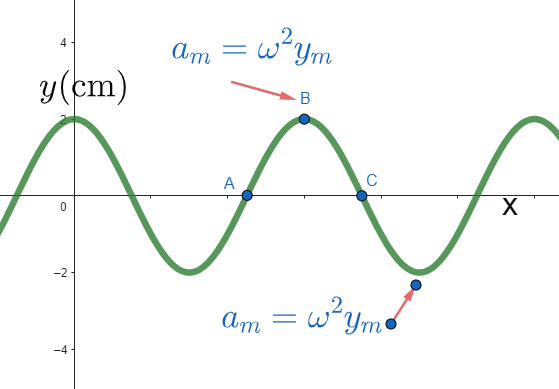
The displacement of a particle moving in simple harmonic motion is given by the equation:
\text{The displacement of a particle moving in simple harmonic motion is given by the equation:}
y(t)=2.0cos(kx−6.0t), where y is in meters and t is in seconds.
\text{ $y(t)=2.0 \cos (kx-6.0 t)$, where $y$ is in meters and $t$ is in seconds. }
What is the maximum acceleration of the particle and where does it occur?
\text{What is the maximum acceleration of the particle and where does it occur?}
y(t)=2cos(kx−6.0t)
y(t)=2 \cos(kx-6.0 t)
ω=6 rad/s
\omega=6 \text{ rad/s}
a(t)=−ω2y(t)
a(t)=-\omega^2 y(t)
a(t) will be maximum for y(t)=−ym
a(t) \text{ will be maximum for } y(t)=-y_m
am=ω2ym=62×2=72 m/s2
a_m=\omega^2 y_m=6^2\times 2=72 \text{ $m/s^2$}
for y(t) minimum
\text{ for $y(t)$ minimum}
The displacement of a particle moving in simple harmonic motion is given by the equation:
\text{The displacement of a particle moving in simple harmonic motion is given by the equation:}
y(t)=2.0cos(kx−6.0t), where y is in meters and t is in seconds.
\text{ $y(t)=2.0 \cos (kx-6.0 t)$, where $y$ is in meters and $t$ is in seconds. }
What is the maximum acceleration of the particle and where does it occur?
\text{What is the maximum acceleration of the particle and where does it occur?}
A stretched string of mass 2.0 g and length 10 cm, carries a wave having the following
\text{A stretched string of mass $2.0 \mathrm{~g}$ and length $10 \mathrm{~cm}$, carries a wave having the following }
displacement wave: y(x,t)=0.05sin(2πx−400πt), where x and y are in meters
\text{displacement wave: $y(x, t)=0.05 \sin (2 \pi x-400 \pi t)$, where $x$ and $y$ are in meters }
and t is in seconds. What is the tension in the string?
\text{and $t$ is in seconds. What is the tension in the string?}

v=μτ⇒ττ=v2μ=(kω)2μ=(kω)2lm=(2π400π)210×10−22×10−3=800 N
\begin{aligned}
v=\sqrt{\frac{\tau}{\mu}} \Rightarrow \tau & =v^2 \mu=\left(\frac{\omega}{k}\right)^2 \mu=\left(\frac{\omega}{k}\right)^2 \frac{m}{l} \\
\tau & =\left(\frac{400 \pi}{2 \pi}\right)^2 \frac{2 \times 10^{-3}}{10 \times 10^{-2}}=800 \mathrm{~N}
\end{aligned}
A stretched string of mass 2.0 g and length 10 cm, carries a wave having the following
\text{A stretched string of mass $2.0 \mathrm{~g}$ and length $10 \mathrm{~cm}$, carries a wave having the following }
displacement wave: y(x,t)=0.05sin(2πx−400πt), where x and y are in meters
\text{displacement wave: $y(x, t)=0.05 \sin (2 \pi x-400 \pi t)$, where $x$ and $y$ are in meters }
and t is in seconds. What is the tension in the string?
\text{and $t$ is in seconds. What is the tension in the string?}
FIGURE 1 shows a graph that represents a transverse wave on a string. The wave is moving
\text{FIGURE 1 shows a graph that represents a transverse wave on a string. The wave is moving}
in the +x direction with a speed of 0.15 m/s. Using the information contained in the graph,
\text{in the $+x$ direction with a speed of $0.15 \mathrm{~m} / \mathrm{s}$. Using the information contained in the graph,}
write the expression for the wave
\text{write the expression for the wave}
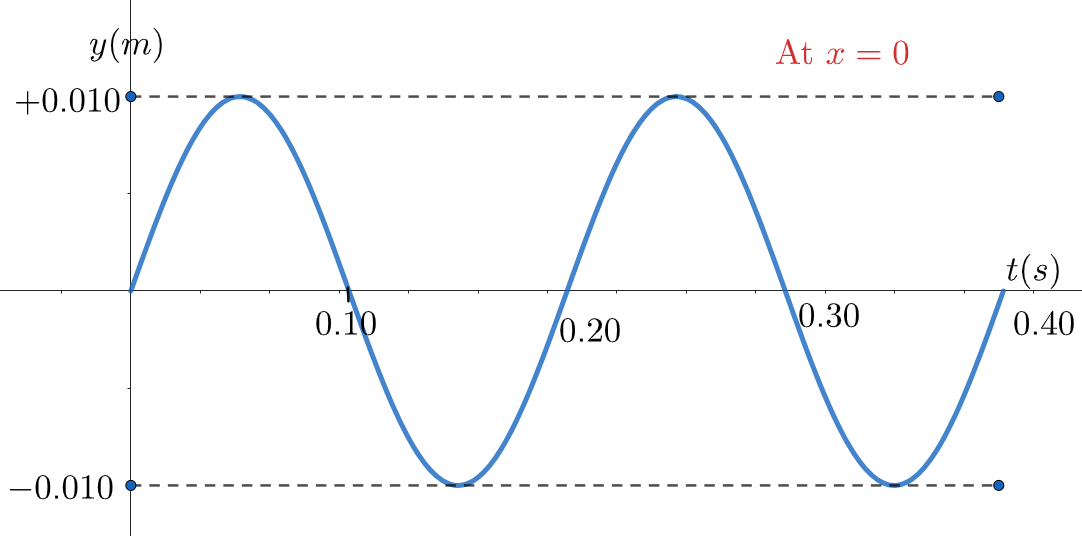
A) y=0.01sin(209x−31.4t)
\text{A) $y=0.01 \sin (209 x-31.4 t)$}
B) y=0.01sin(209x+31.4t)
\text{B) $y=0.01 \sin (209 x+31.4 t)$}
C) y=0.04sin(209x−6.28t)
\text{C) $y=0.04 \sin (209 x-6.28 t)$}
D) y=0.01sin(0.942x−31.4t)
\text{D) $y=0.01 \sin (0.942 x-31.4 t)$}
E) y=0.01sin(0.942x+31.4t)
\text{E) $y=0.01 \sin (0.942 x+31.4 t)$}
FIGURE 1 shows a graph that represents a transverse wave on a string. The wave is moving
\text{FIGURE 1 shows a graph that represents a transverse wave on a string. The wave is moving}
in the +x direction with a speed of 0.15 m/s. Using the information contained in the graph,
\text{in the $+x$ direction with a speed of $0.15 \mathrm{~m} / \mathrm{s}$. Using the information contained in the graph,}
write the expression for the wave
\text{write the expression for the wave}

ω=T2π=0.22π=10π=31.4 rad/s k=vω=0.1510π=3200π=209 rad/m
\begin{aligned}
& \omega=\frac{2 \pi}{T}=\frac{2 \pi}{0.2}=10 \pi=31.4 \text{ rad/s}\\
&\\
& \mathrm{~k}=\frac{\omega}{v}=\frac{10 \pi}{0.15}=\frac{200}{3} \pi=209 \text{ rad/m}
\end{aligned}
ym=0.01 m
y_m=0.01 \text{ m}
T=0.2 s
\mathrm{T}=0.2\text{ s}
right moving wave, so: y=0.01sin(209x−31.4t)
\text{right moving wave, so: $ y=0.01 \sin (209 x-31.4 t)$ }
The amplitude is:
\text{The amplitude is:}
Graph: displacement vs time
\text{Graph: displacement vs time}
Two pieces of string, each of length L=1.5 m, are joined together end to end, to make
\text{Two pieces of string, each of length $L=1.5 \mathrm{~m}$, are joined together end to end, to make }
a 3.0 m long combined string. The first piece of string has mass per unit length μ1=100 g/m,
\text{a $3.0 \mathrm{~m}$ long combined string. The first piece of string has mass per unit length $\mu_1=100$ $\mathrm{g} / \mathrm{m}$, }
the second piece has mass per unit length μ2=6.0μ1.
\text{the second piece has mass per unit length $\mu_2=6.0 \mu_1$.}
travelling wave to travel the entire 3.0 m length of the string?
\text{travelling wave to travel the entire $3.0 \mathrm{~m}$ length of the string?}
If the combined string is under tension τ=5.0 N, how much time does it take a transverse
\text{If the combined string is under tension $\tau=5.0 \mathrm{~N}$, how much time does it take a transverse }
A) 0.73 s
\text{A) $0.73 \mathrm{~s}$}
B) 1.1 s
\text{B) $1.1 \mathrm{~s}$}
C) 1.7 s
\text{C) $1.7 \mathrm{~s}$}
D) 1.9 s
\text{D) $1.9 \mathrm{~s}$}
E) 2.8 s
\text{E) $2.8 \mathrm{~s}$}
Two pieces of string, each of length L=1.5 m, are joined together end to end, to make
\text{Two pieces of string, each of length $L=1.5 \mathrm{~m}$, are joined together end to end, to make }
a 3.0 m long combined string. The first piece of string has mass per unit length μ1=100 g/m,
\text{a $3.0 \mathrm{~m}$ long combined string. The first piece of string has mass per unit length $\mu_1=100$ $\mathrm{g} / \mathrm{m}$, }
the second piece has mass per unit length μ2=6.0μ1.
\text{the second piece has mass per unit length $\mu_2=6.0 \mu_1$.}
travelling wave to travel the entire 3.0 m length of the string?
\text{travelling wave to travel the entire $3.0 \mathrm{~m}$ length of the string?}
If the combined string is under tension τ=5.0 N, how much time does it take a transverse
\text{If the combined string is under tension $\tau=5.0 \mathrm{~N}$, how much time does it take a transverse }
tTot =L(v11+v21)=L(τμ1+τμ2)=1.5[50.1+50.6]=0.731 s
\displaystyle t_{\text {Tot }}=L\left(\frac{1}{v_1}+\frac{1}{v_2}\right)=L\left(\sqrt{\frac{\mu_1}{\tau}}+\sqrt{\frac{\mu_2}{\tau}}\right)=1.5\left[\sqrt{\frac{0.1}{5}}+\sqrt{\frac{0.6}{5}}\right]=0.731 \mathrm{~s}
tTot=t1+t2
t_\text{Tot}=t_1+t_2
The cords in the three configurations are the same. All the objects have the same mass.
\text{The cords in the three configurations are the same. All the objects have the same mass.}
In which configuration, the wave speed in the cord is maximum?
\text{In which configuration, the wave speed in the cord is maximum?}
The cords in the three configurations are the same. All the objects have the same mass.
\text{The cords in the three configurations are the same. All the objects have the same mass.}
In which configuration, the wave speed in the cord is maximum?
\text{In which configuration, the wave speed in the cord is maximum?}
τ1
\tau_1
τ2
\tau_2
τ3
\tau_3
τ1=mg
\tau_1=mg
τ2=mg
\tau_2=mg
τ3=2mg
\tau_3=2mg
τ2
\tau_2
The highest speed is in configuration 3
\text{The highest speed is in configuration 3}
The speeds in configurations 1 and 2 are tie
\text{The speeds in configurations 1 and 2 are tie}
(The tension in the string is constant)
(\text{The tension in the string is constant})
(we neglect the mass of the hanging string)
(\text{we neglect the mass of the hanging string})
mg
mg
mg
mg
mg
mg
2mg
2mg
A 0.550 m long string fixed at both ends is vibrating in its fundamental mode.
\text{A $0.550 \mathrm{~m}$ long string fixed at both ends is vibrating in its fundamental mode. }
The maximum transverse acceleration of a point at the middle of the string is 8.40×103 m/s2
\text{The maximum transverse acceleration of a point at the middle of the string is $8.40 \times 10^3$ $\mathrm{m} / \mathrm{s}^2$ }
and the maximum transverse velocity is 3.80 m/s.
\text{and the maximum transverse velocity is $3.80 \mathrm{~m} / \mathrm{s}$.}
What is the wave speed of the transverse waves traveling on this string?
\text{What is the wave speed of the transverse waves traveling on this string? }
umaxamax=ym′ωym′ω2=ω=3.88.4×103=2210.5rad/sλ=2L=2×0.55=1.1 mv=kω=2πλω=2π1.1×2210.5=386.99 m/s
\begin{aligned}
& \frac{a_{\max }}{u_{\max }}=\frac{y_m^{\prime} \omega^2}{y_m^{\prime} \omega}=\omega=\frac{8.4 \times 10^3}{3.8}=2210.5 \mathrm{rad} / \mathrm{s} \\
& \lambda=2 L=2 \times 0.55=1.1 \mathrm{~m} \\
& v=\frac{\omega}{k}=\frac{\lambda \omega}{2 \pi}=\frac{1.1 \times 2210.5}{2 \pi}=386.99 \mathrm{~m} / \mathrm{s}
\end{aligned}
A 0.550 m long string fixed at both ends is vibrating in its fundamental mode.
\text{A $0.550 \mathrm{~m}$ long string fixed at both ends is vibrating in its fundamental mode. }
The maximum transverse acceleration of a point at the middle of the string is 8.40×103 m/s2
\text{The maximum transverse acceleration of a point at the middle of the string is $8.40 \times 10^3$ $\mathrm{m} / \mathrm{s}^2$ }
and the maximum transverse velocity is 3.80 m/s.
\text{and the maximum transverse velocity is $3.80 \mathrm{~m} / \mathrm{s}$.}
What is the wave speed of the transverse waves traveling on this string?
\text{What is the wave speed of the transverse waves traveling on this string? }
(because it is the fundamental)
\text{(because it is the fundamental)}
y′(x,t)=[2ymsinkx]cosωt.
y^{\prime}(x, t)=\left[2 y_m \sin k x\right] \cos \omega t .
(standing wave equation)
\text{(standing wave equation)}
u(x,t)=−[2ymωsinkx]sinωt.
u(x, t)=-\left[2 y_m \omega \sin k x\right] \sin\omega t .
a(x,t)=−[2ymω2sinkx]cosωt.
a(x, t)=-\left[2 y_m \omega^2 \sin k x\right] \cos\omega t .
Two sinusoidal waves, identical except for phase, travel in the same direction along
\text{Two sinusoidal waves, identical except for phase, travel in the same direction along }
a stretched string, producing a resultant wave y(x,t)=0.097sin(15x−2.4t+0.78),
\text{a stretched string, producing a resultant wave $y(x, t)=0.097 \sin (15 x-2.4 t+0.78)$,}
where x is in meters and t is in seconds. What is the amplitude of the interfering waves?
\text{where $x$ is in meters and $t$ is in seconds. What is the amplitude of the interfering waves? }
A) 0.068 m
\text{A) $0.068 \mathrm{~m}$}
B) 0.052 m
\text{B) $0.052 \mathrm{~m}$}
C) 0.097 m
\text{C) $0.097 \mathrm{~m}$}
D) 0.035 m
\text{D) $0.035 \mathrm{~m}$}
E) 0.044 m
\text{E) $0.044 \mathrm{~m}$}
Two sinusoidal waves, identical except for phase, travel in the same direction along
\text{Two sinusoidal waves, identical except for phase, travel in the same direction along }
a stretched string, producing a resultant wave y(x,t)=0.097sin(15x−2.4t+0.78),
\text{a stretched string, producing a resultant wave $y(x, t)=0.097 \sin (15 x-2.4 t+0.78)$,}
where x is in meters and t is in seconds. What is the amplitude of the interfering waves?
\text{where $x$ is in meters and $t$ is in seconds. What is the amplitude of the interfering waves? }
y′(x,t)=[2ymcos21ϕ]sin(kx−ωt+21ϕ).
y^{\prime}(x, t)=\left[2 y_m \cos \frac{1}{2} \phi\right] \sin \left(k x-\omega t+\frac{1}{2} \phi\right) \text {. }
We can see that: 2ϕ=0.78 rad
\text{We can see that: }\frac{\phi}{2}=0.78 \text{ rad}
2ym∣cos21ϕ∣=0.097⇒ym=2∣cos21ϕ∣0.097=2∣cos0.78∣0.097=0.068 m
2 y_m |\cos \frac{1}{2} \phi|=0.097 \Rightarrow y_m=\displaystyle \frac{0.097}{2 |\cos \frac{1}{2} \phi|}=\frac{0.097}{2 |\cos 0.78|}=0.068 \text{ m}
(resultant wave)
\text{(resultant wave)}
A)1.83 m B)1.22 m C)1.12 m D)2.86 m E)2.12 m
\begin{gathered}
\text{A)1.83 m }\\
\text{B)1.22 m } \\
\text{C)1.12 m }\\
\text{D)2.86 m }\\
\text{E)2.12 m }
\end{gathered}
A light string is fixed between two supports with two successive standing-wave frequenciesoccur at 525 Hz and 630 Hz. There are other standing-wave frequencies lower than 525 Hzand higher than 630 Hz. If the speed of transverse waves on the string is 384 m/ s, then find the length of the string?
\begin{aligned}
&\text{A light string is fixed between two supports with two successive standing-wave frequencies}\\
&\text{occur at 525 Hz and 630 Hz. There are other standing-wave frequencies lower than 525 Hz}\\
&\text{and higher than 630 Hz. If the speed of transverse waves on the string is 384 m/ s, then find }\\
&\text{the length of the string?}
\end{aligned}
f1=630−525=105 Hzf1=2Lv=2L384=105 Hz⇒L=1.83 m
\begin{aligned}
& f_1=630-525=105 \mathrm{~Hz} \\
& f_1=\frac{ v}{2 L}=\frac{384}{2 L}=105 \mathrm{~Hz} \\
& \Rightarrow L=1.83 \mathrm{~m}
\end{aligned}
The difference between successive frequencies gives the fundamental
\text{The difference between successive frequencies gives the fundamental}
A light string is fixed between two supports with two successive standing-wave frequenciesoccur at 525 Hz and 630 Hz. There are other standing-wave frequencies lower than 525 Hzand higher than 630 Hz. If the speed of transverse waves on the string is 384 m/ s, then find the length of the string?
\begin{aligned}
&\text{A light string is fixed between two supports with two successive standing-wave frequencies}\\
&\text{occur at 525 Hz and 630 Hz. There are other standing-wave frequencies lower than 525 Hz}\\
&\text{and higher than 630 Hz. If the speed of transverse waves on the string is 384 m/ s, then find }\\
&\text{the length of the string?}
\end{aligned}
A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point
\text{A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point }
30 cm from one end of the string is half the maximum amplitude. How long is the string?
\text{$30 \mathrm{~cm}$ from one end of the string is half the maximum amplitude. How long is the string?}

A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point
\text{A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point }
30 cm from one end of the string is half the maximum amplitude. How long is the string?
\text{$30 \mathrm{~cm}$ from one end of the string is half the maximum amplitude. How long is the string?}
L=23λ⇒k=λ2π=2π×2L3=L3π
L=\frac{3 \lambda}{2} \Rightarrow k=\frac{2 \pi}{\lambda}=2 \pi \times \frac{3}{2 L}=\frac{3 \pi}{L}
Amplitude at (x=0.3m);ym′=2ymsinkx=2ymsin(L3π×0.3)=ym
\operatorname{Amplitude}\text{ at }(x=0.3 m) ; y'_m=2 y_m \operatorname{sin}k x=2 y_m \sin \left(\frac{3 \pi}{L} \times 0.3\right)=y_m
1=2sin(L0.9π)
1=2 \sin \left(\frac{0.9 \pi}{L}\right)
L=sin−1(21)0.9π=30∘0.9π=π/60.9π=6×0.9=5.4 m
L=\frac{0.9 \pi}{\sin ^{-1}\left(\frac{1}{2}\right)}=\frac{0.9 \pi}{30^{\circ}}=\frac{0.9 \pi}{\pi / 6}=6 \times 0.9=5.4 \mathrm{~m}
(maximum amplitude is 2ym)
\text{(maximum amplitude is $2y_m$)}
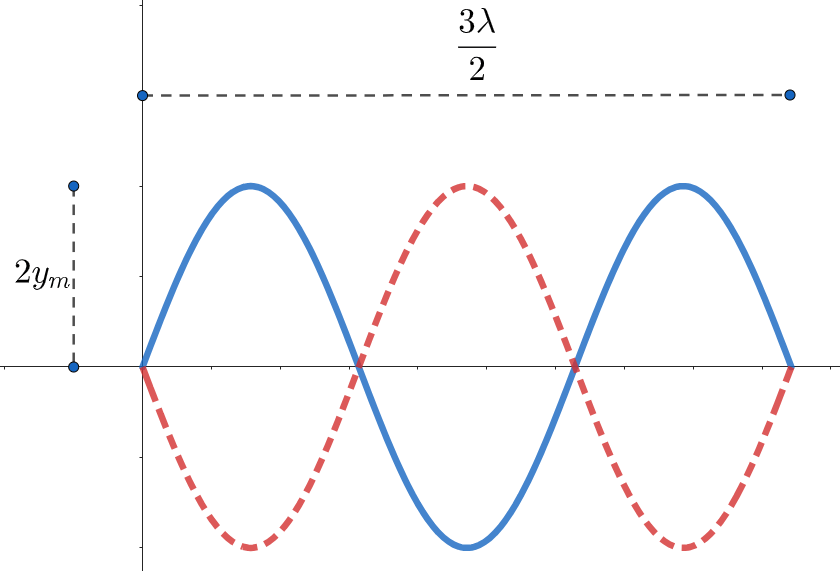
A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point
\text{A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point }
30 cm from one end of the string is half the maximum amplitude. How long is the string?
\text{$30 \mathrm{~cm}$ from one end of the string is half the maximum amplitude. How long is the string?}
ym′=2ym∣sinkx∣=2ymsin(L3π×0.3)=ym
y'_m=2 y_m |\operatorname{sin}k x|=2 y_m \Big|\sin \left(\frac{3 \pi}{L} \times 0.3\right)\Big|=y_m
21=sin(L0.9π)
\frac{1}{2}=\Big| \sin \left(\frac{0.9 \pi}{L}\right)\Big|
L=sin−1(±21)0.9π
L=\frac{0.9 \pi}{\sin ^{-1}\left(\displaystyle\textcolor{red}{\pm}\frac{1}{2}\right)}

The previous solution is actually incomplete.
\text{The previous solution is actually incomplete.}
The correct way is to do it as follows
\text{The correct way is to do it as follows}
sin−1(21)=6π+2πn
\sin^{-1}(\frac{1}{2})=\frac{\pi}{6}+2\pi n
sin−1(21)=65π+2πn
\sin^{-1}(\frac{1}{2})=\frac{5\pi}{6}+2\pi n
or
\text{ or }
with n integer
\text{with $n$ integer}
A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point
\text{A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point }
30 cm from one end of the string is half the maximum amplitude. How long is the string?
\text{$30 \mathrm{~cm}$ from one end of the string is half the maximum amplitude. How long is the string?}
L=sin−1(±21)0.9π
L=\frac{0.9 \pi}{\sin ^{-1}\left(\displaystyle\pm\frac{1}{2}\right)}

sin−1(21)=6π+2πn
\sin^{-1}(\frac{1}{2})=\frac{\pi}{6}+2\pi n
a)
a)
L=1+12n5.4
L=\frac{5.4}{1+12n}
the acceptable n for which L>(x=0.3) are
\text{the acceptable $n$ for which $L>(x=0.3)$ are}
n=0,1
n=0,1
So, L=5.4 m or L=0.42 m
\text{So, $L=5.4 $ m or $L=0.42 $ m}
sin−1(21)=65π+2πn
\sin^{-1}(\frac{1}{2})=\frac{5\pi}{6}+2\pi n
L=5+12n5.4
L=\frac{5.4}{5+12n}
the acceptable n for which L>(x=0.3) are
\text{the acceptable $n$ for which $L>(x=0.3)$ are}
n=0,1
n=0,1
So, L=1.08 m or L=0.32 m
\text{So, $L=1.08 $ m or $L=0.32 $ m}
b)
b)
A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point
\text{A string oscillates in a third -harmonic standing wave pattern. The amplitude at a point }
30 cm from one end of the string is half the maximum amplitude. How long is the string?
\text{$30 \mathrm{~cm}$ from one end of the string is half the maximum amplitude. How long is the string?}
L=sin−1(±21)0.9π
L=\frac{0.9 \pi}{\sin ^{-1}\left(\displaystyle\pm\frac{1}{2}\right)}

sin−1(−21)=−6π+2πn
\sin^{-1}(-\frac{1}{2})=-\frac{\pi}{6}+2\pi n
c)
c)
L=−1+12n5.4
L=\frac{5.4}{-1+12n}
the acceptable n for which L>(x=0.3) are
\text{the acceptable $n$ for which $L>(x=0.3)$ are}
n=1
n=1
So, L=0.42 m
\text{So, $L=0.42 $ m}
sin−1(−21)=−65π+2πn
\sin^{-1}(-\frac{1}{2})=-\frac{5\pi}{6}+2\pi n
L=−5+12n5.4
L=\frac{5.4}{-5+12n}
the acceptable n for which L>(x=0.3) are
\text{the acceptable $n$ for which $L>(x=0.3)$ are}
n=1,2
n=1,2
So, L=0.77 m
\text{So, $L=0.77 $ m}
d)
d)
⋅
\cdot
⋅
\cdot
⋅
\cdot
⋅
\cdot
⋅
\cdot
⋅
\cdot
(as we can see from the figure, there are 6 possible points)
\text{(as we can see from the figure, there are 6 possible points)}
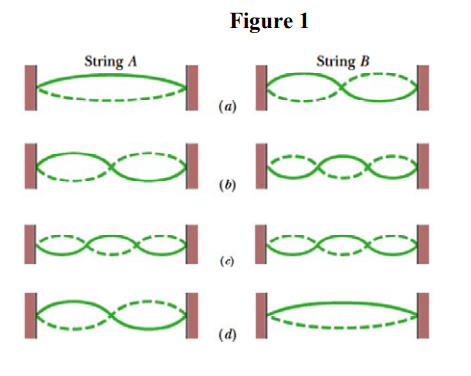
Strings A and B have identical lengths and linear densities, but string A is under greater tension
\text{Strings A and B have identical lengths and linear densities, but string A is under greater tension }
than string B. Figure 1 shows four situations, in which standing wave patterns exist on the two
\text{than string B. Figure 1 shows four situations, in which standing wave patterns exist on the two }
strings. In which situation(s) is there the possibility that strings A and B are oscillating at the
\text{strings. In which situation(s) is there the possibility that strings A and B are oscillating at the}
same resonant frequency?
\text{ same resonant frequency? }
Strings A and B have identical lengths and linear densities, but string A is under greater tension
\text{Strings A and B have identical lengths and linear densities, but string A is under greater tension }
than string B. Figure 1 shows four situations, in which standing wave patterns exist on the two
\text{than string B. Figure 1 shows four situations, in which standing wave patterns exist on the two }
strings. In which situation(s) is there the possibility that strings A and B are oscillating at the
\text{strings. In which situation(s) is there the possibility that strings A and B are oscillating at the}
same resonant frequency?
\text{ same resonant frequency? }
v=μτvA>vBfnA=2LnAvA,fnB=2LnBvB2LnAvA=2LnBvB
\begin{aligned}
& v=\sqrt{\frac{\tau}{\mu}} \\
& v_A>v_B \\
& f_{n A}=\frac{n_A v_A}{2 L}, f_{n B}=\frac{n_B v_B}{2 L} \\
& \frac{n_A v_A}{2 L}=\frac{n_B v_B}{2 L}
\end{aligned}

nB=vBvAnA
n_B=\frac{v_A}{v_B} n_A
⇒
\Rightarrow
nB>nA
n_B> n_A
because (vA>vB)
\text{because $(v_A>v_B$)}
⇒
\Rightarrow
The possible situations are a and b
\text{The possible situations are a and b}

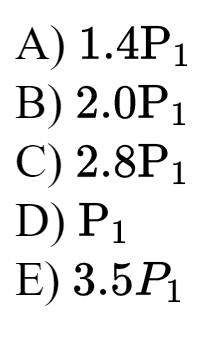

P1=21μ1v1ω2ym2μ2=2μ1;v2=μ2τ=2v1P2=212μ12v1ω2ym2P2=22[21μ1v1ω2ym2]=2P1≈1.4P1
\begin{aligned}
& P_1=\frac{1}{2} \mu_1 v_1\omega^2 y_m^2 \\
& \mu_2=2 \mu_1 ; v_2=\sqrt{\frac{\tau}{\mu_2}}=\frac{v_1}{\sqrt{2}} \\
& P_2=\frac{1}{2} 2 \mu_1 \frac{v_1}{\sqrt{2}} \omega^2 y_m^2 \\
& P_2=\frac{2}{\sqrt{2}}\left[\frac{1}{2} \mu_1 v_1\omega^2 y_m^2 \right]=\sqrt{2} P_1 \approx 1.4 P_1
\end{aligned}
Standing waves are created in a 160 cm long string. The string has two adjacent resonances
\text{Standing waves are created in a $160 \mathrm{~cm}$ long string. The string has two adjacent resonances }
at frequencies of 85.0 Hz and 102 Hz.
\text{at frequencies of $85.0 \mathrm{~Hz}$ and $102 \mathrm{~Hz}$.}
What is the length of each loop at the 85.0 Hz resonance?
\text{What is the length of each loop at the $85.0 \mathrm{~Hz}$ resonance?}
A) 32.0 cm
\text{A) $32.0 \mathrm{~cm}$}
B) 10.4 cm
\text{B) $10.4 \mathrm{~cm}$}
C) 68.0 cm
\text{C) $68.0 \mathrm{~cm}$}
D) 83.5 cm
\text{D) $83.5 \mathrm{~cm}$}
E) 25.7 cm
\text{E) $25.7 \mathrm{~cm}$}
Standing waves are created in a 160 cm long string. The string has two adjacent resonances
\text{Standing waves are created in a $160 \mathrm{~cm}$ long string. The string has two adjacent resonances }
at frequencies of 85.0 Hz and 102 Hz.
\text{at frequencies of $85.0 \mathrm{~Hz}$ and $102 \mathrm{~Hz}$.}
What is the length of each loop at the 85.0 Hz resonance?
\text{What is the length of each loop at the $85.0 \mathrm{~Hz}$ resonance?}
Adjacent resonances⇒fn+1−fn=102−85.0=17 Hz
\text{Adjacent resonances} \Rightarrow f_{n+1}-f_n=102-85.0=17 \text{ Hz}
So, the fundamental frequency is:f1=17 Hz
\text{So, the fundamental frequency is}: f_1=17 \text{ Hz}
fn=nf1⇒n=f1fn=1785.0=5
f_n=n f_1\Rightarrow n=\frac{f_n}{f_1}=\frac{85.0}{17}=5
85.0 Hz is the frequency of the 5th harmonic
85.0 \text{ Hz}\text{ is the frequency of the $5^{th}$ harmonic}
The 5th harmonics has 5 loops. The length of one loop is d=L/5=160/5=32 cm
\text{The $5^\text{th}$ harmonics has 5 loops. The length of one loop is }d=L/5=160/5=32 \text{ cm}
C h a p t e r 1 6 \text{Chapter 16} C h a p t e r 1 6 \text{Chapter 16} C h a p t e r 1 6 \text{Chapter 16} C h a p t e r 1 6 \text{Chapter 16} D r . A d e l A b b o u t \text{Dr. Adel Abbout}
Chapter 16-Phys102
By smstry
Chapter 16-Phys102
- 865