Downloading many-body continuous variable entanglement to qubits
Zhihua Han, Kero Lau
Simon Fraser University
CAP Congress
May 28, 2024
Imagine I have some qubits:
|+\rangle
Qubit cluster state
and now I entangle the edges with the (qubit) CZ gate.
\hat{C}_Z
|+\rangle
Qubit cluster state
\hat{C}_Z = \begin{bmatrix}
1 & 0 & 0 & 0 \\
0 & 1 & 0 & 0 \\
0 & 0 & 1 & 0 \\
0 & 0 & 0 &-1
\end{bmatrix}
\begin{aligned}
&= |G\rangle
\end{aligned}
The quantum state specified by \(G\) is called a qubit cluster state.
\begin{aligned}
&= |G\rangle\\
&= \prod_{i, j \in E}\hat{C}_{Z_{ij}} |+\rangle^{\otimes N}
\end{aligned}
\hat{C}_Z
|+\rangle
Qubit cluster state
Qubit cluster state
+
Single qubit measurements
Fault tolerant universal quantum computation
(Raussendorf 2001)
Why we need qubit cluster state
Superconducting qubits: 51
Photonic qubits: 14
Trapped ion: 32
Qubit cluster state
Why we need qubit cluster state
Goal: Make many body entanglement in physical qubits
How do we make scalable qubit cluster states?
"Downloading entanglement from a CV cluster state"
Continuous variable (CV) cluster state
Physical qubits
|0, \sigma_p \rangle_p
\hat{C}_Z^\mathrm{CV}
Entanglement Transfer Protocol
How do we make scalable qubit cluster states?
Entanglement Transfer Protocol
Continuous variable (CV) cluster state
Qubit cluster state
|0, \sigma_p \rangle_p
\hat{C}_Z^\mathrm{CV}
Entanglement Transfer Protocol
Now if I have some bosons:
|0, \sigma_p \rangle_p
CV cluster state
Squeezed state
and entangle them with CV CZ gate:
|0, \sigma_p \rangle_p
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1 \hat{q}_2}
CV cluster state
We say it is a CV cluster state.
|0, \sigma_p \rangle_p
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1 \hat{q}_2}
\begin{aligned}
&= |G\rangle^\mathrm{CV}
\end{aligned}
\begin{aligned}
&= |G\rangle^\mathrm{CV}\\
&= \prod_{i, j \in E}\hat{C}_{Z_{ij}}^\mathrm{CV} |0, \sigma_p \rangle_p^{\otimes N}
\end{aligned}
CV cluster state
\(\sigma_p\) represents the variance of the squeezed state.
|0, \sigma_p \rangle_p
When \(\sigma_p \to 0\), the CV cluster state is an ideal CV cluster state.
\(p\)
|0, \sigma_p \rangle_p
Finite vs Ideal CV cluster state
\(\sigma_p\) represents the variance of the squeezed state.
|0, \sigma_p \rangle_p
When \(\sigma_p \to 0\), the CV cluster state is an ideal CV cluster state.
\(p\)
|0\rangle_p
Finite vs Ideal CV cluster state
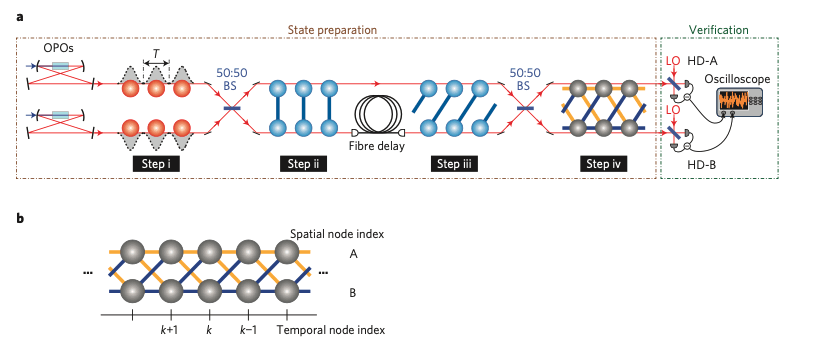
10000 modes! 1D, (Furusawa 2013)
How to make CV cluster state
1 million modes, 1D, (Furusawa 2016)
How to make CV cluster state
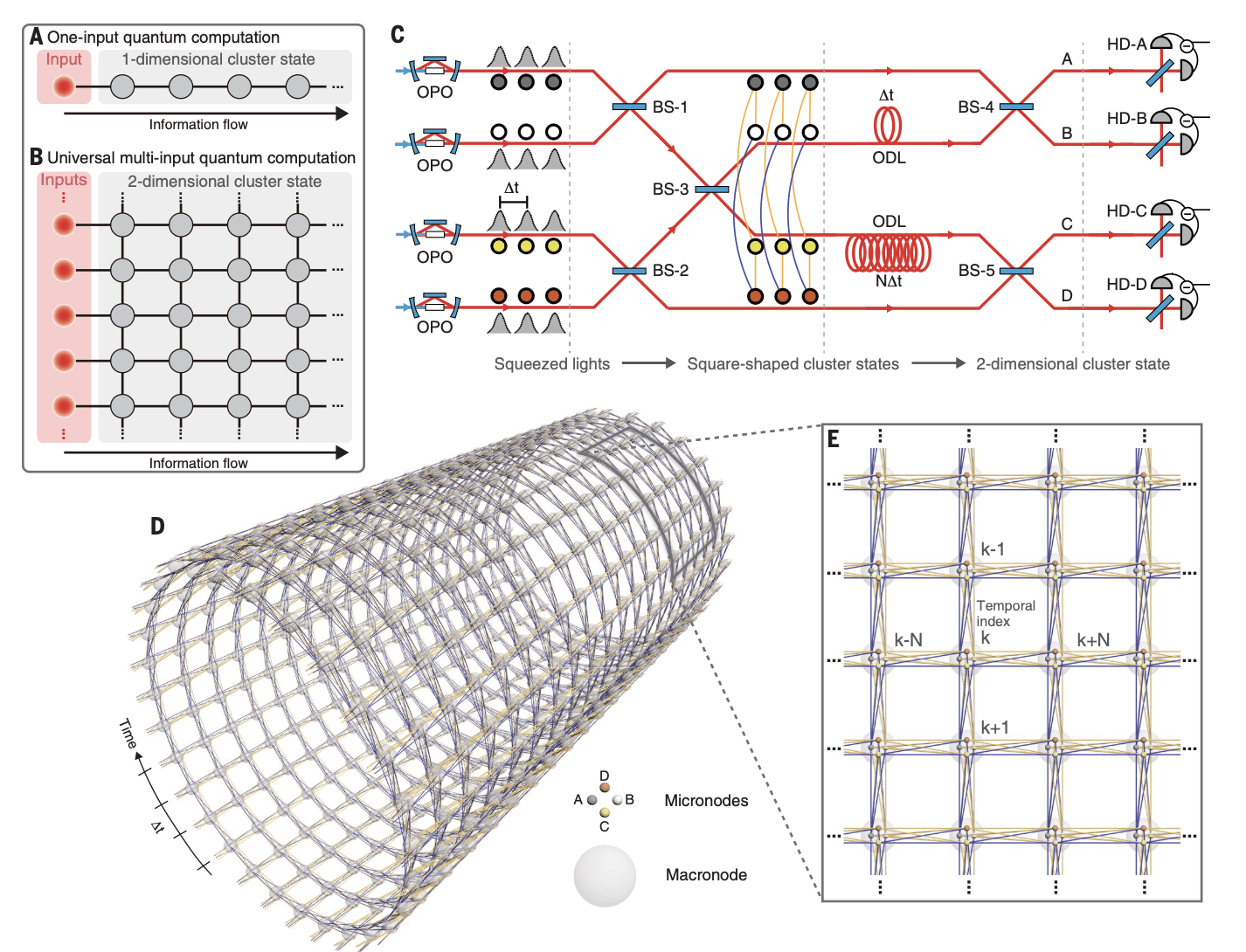
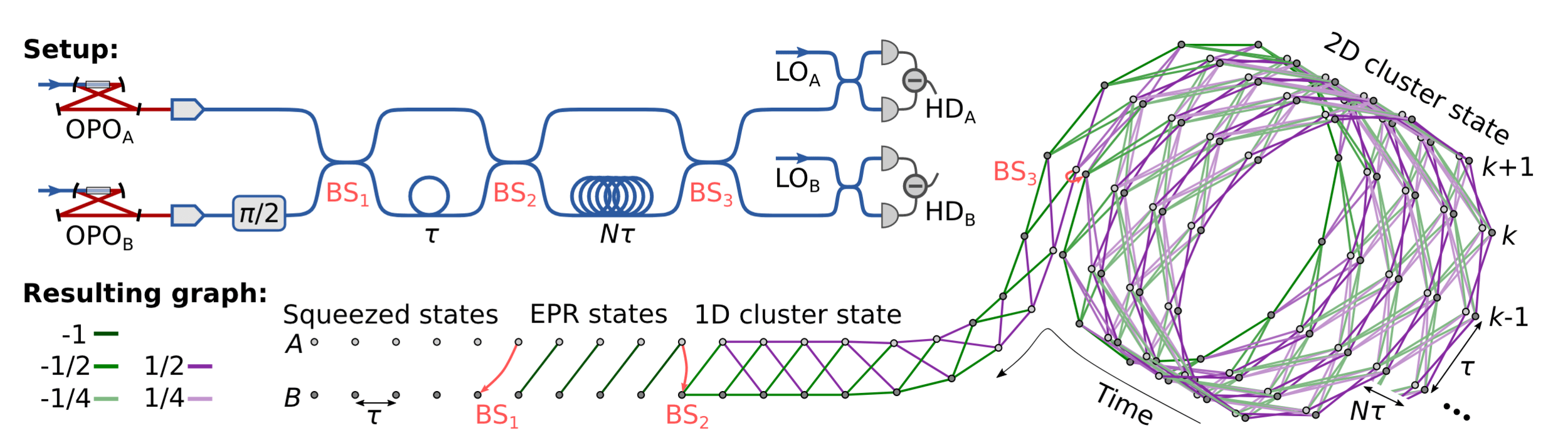
5x1240 modes, 2D, (Furusawa 2019)
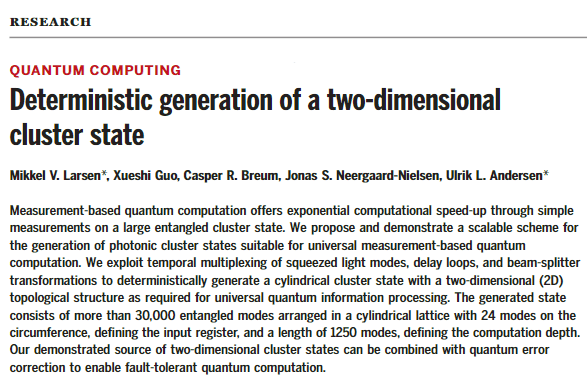
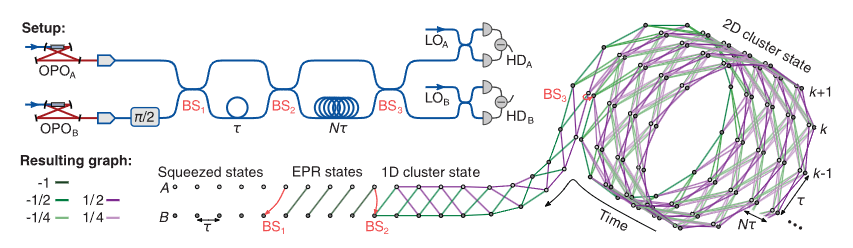
24x1250, 2D (Andersen 2019)
How to make CV cluster state
Ideal CV cluster state
|G\rangle^\mathrm{CV} = \prod_{i, j \in E}\hat{C}_{Z_{ij}}^\mathrm{CV} |0\rangle_p^{\otimes N}
|0\rangle_p
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1 \hat{q}_2}
Qubit cluster state
\begin{aligned}
|G\rangle= \prod_{i, j \in E}\hat{C}_{Z_{ij}} |+\rangle^{\otimes N}
\end{aligned}
|+\rangle
\hat{C}_Z = \text{diag}(1, 1, 1, -1)
CV vs qubit cluster state
Continuous variable (CV) cluster state
Physical qubits
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
How to perform entanglement transfer?
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1 \hat{q}_2}
\hat{C}_Z = \text{diag}(1, 1, 1, -1)
Entanglement transfer protocol
How to perform entanglement transfer?
Continuous variable (CV) cluster state
Qubit cluster state
|0\rangle_p
\hat{C}_Z
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1 \hat{q}_2}
\hat{C}_Z = \text{diag}(1, 1, 1, -1)
Entanglement transfer protocol
We need:
- A CV cluster state*
- \(\hat{q}\) quadrature homodyne detection
- Conditional displacement gate \( \hat{C}_D\)
\hat{C}_D = |0\rangle \langle 0| \hat I + |1\rangle \langle 1| \hat{D}_q({\sqrt{\pi}})
How to perform entanglement transfer?
Entanglement transfer protocol
x
\(\psi (x)\)
\(e^{-ia\hat p}\psi (x)\)
Displacement gate of strength \(a\) shifts the state.
\(a\)
ETP: Displacement Gate
\hat D_q(a) |x\rangle:= |x + a\rangle
\hat D_q(a) |x\rangle:= |x + a\rangle
\(q\)
\(p\)
ETP: Displacement Gate
Displacement gate of strength \(a\) shifts the state.
\(q\)
\(p\)
\(a\)
ETP: Displacement Gate
\hat D_q(a) |x\rangle:= |x + a\rangle
Displacement gate of strength \(a\) shifts the state.
1. Initialize all qubits to \(|+\rangle\).
(These are physical qubits)
|+\rangle
ETP: Overview
Step 2: Get a CV cluster state.
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
ETP: Overview
Step 3. Apply conditional displacement to each pair:
\(\hat{C}_D = |0\rangle \langle 0| \hat I + |1\rangle \langle 1| \hat{D}_q({\sqrt{\pi}})\)
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
ETP: Overview
Step 3. Apply conditional displacement to each pair:
\(\hat{C}_D = |0\rangle \langle 0| \hat I + |1\rangle \langle 1| \hat{D}_q({\sqrt{\pi}})\)
\hat{C}_D
ETP: Overview
Step 4. Measure \(q\) quadrature.
ETP: Overview
You now have a qubit cluster state!
But why does it work?
ETP: Overview
We show there is a hidden qubit cluster state inside a CV cluster state!
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
\(q\)
\(q\)
\(q\)
\(q\)
CV cluster state
Qubit cluster inside CV cluster
|0\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |2n\sqrt{\pi}\rangle_q
Gottesman-Kitaev-Preskill (GKP state)
\(q\)
\(|2n\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
GKP Background
|1\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |(2n+1)\sqrt{\pi}\rangle_q
\(q\)
\(|(2n+1)\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
\(\sqrt \pi\)
\(3\sqrt \pi\)
\(5\sqrt \pi\)
\hat{X}^{\mathrm{GKP}} = e^{-i \sqrt \pi \hat p} = \hat{D}_q(\sqrt{\pi})
GKP Background
|1\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |(2n+1)\sqrt{\pi}\rangle_q
\(q\)
\(|(2n+1)\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
\(\sqrt \pi\)
\(3\sqrt \pi\)
\(5\sqrt \pi\)
\(|2n\sqrt{\pi}\rangle\)
|0\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |2n\sqrt{\pi}\rangle_q
GKP Background
|+\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |n\sqrt{\pi}\rangle_q = \frac{1}{\sqrt{2}}(|0\rangle_\mathrm{GKP} + |1\rangle_\mathrm{GKP})
\(|2n\sqrt{\pi}\rangle\)
\(q\)
\(|(2n+1)\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
\(\sqrt \pi\)
\(3\sqrt \pi\)
\(5\sqrt \pi\)
GKP Background
\(p\)
|0\rangle_p
Node of ideal CV cluster
\(q\)
|0\rangle_p
Node of ideal CV cluster
\(q\)
|0\rangle_p
|+\rangle_\mathrm{GKP}
GKP state
Node of ideal CV cluster
\(q\)
|0\rangle_p
\(\mu_q\)
\mu_q \in [-\frac{\sqrt{\pi}}{2}, -\frac{\sqrt{\pi}}{2})
\hat{D}_q(\mu_q)|+\rangle_\mathrm{GKP}
Displaced GKP state
|+_{\mu_q, 0} \rangle_\mathrm{GKP} \equiv \hat{D}_q(\mu_q)|+\rangle_\mathrm{GKP}
(Glancy 2006)
Displaced GKP
\(q\)
|0\rangle_p
|+_{\mu_q, 0} \rangle_\mathrm{GKP} \equiv \hat{D}_q(\mu_q)|+\rangle_\mathrm{GKP}
So if we integrate over \(\mu_q\), we should form an ideal \(|0\rangle_p\) state.
Node of ideal CV cluster is superposition of displaced GKP
Displaced GKP Basis
(Glancy 2006)
\(q\)
|0\rangle_p
+
+
+
+
= \int d\mu_q
Node of ideal CV cluster is superposition of displaced GKP
=
Displaced GKP Basis
CV Basis
\(q\)
|0\rangle_p
Edges of ideal CV cluster
\(q\)
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
=
CV Basis
\(q\)
|0\rangle_p
Edges of ideal CV cluster
+
+
+
+
+
+
+
+
\(q\)
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
=
=
\(q\)
|0\rangle_p
+
+
+
+
+
+
+
+
\(q\)
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
= \iint d \mu_{q_1} d \mu_{q_2}
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV}
Edges of ideal CV cluster
\hat{C}_Z^\mathrm{CV}
Displaced GKP Basis
CV Basis
\(q\)
|0\rangle_p
+
+
+
+
+
+
+
+
\(q\)
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV}
Edges of ideal CV cluster
= \iint d \mu_{q_1} d \mu_{q_2}
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV}
\(q\)
|+_{\mu_q, 0} \rangle_\mathrm{GKP}
is a superposition of
Nodes of a ideal CV cluster state
Displaced GKP states
\hat{C}_Z^\mathrm{CV}
|0\rangle_p
\(q\)
\(q\)
\(q\)
Node of ideal CV cluster is displaced GKP
The edges of the CV cluster state?
GKP CZ gate
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
\(q\)
\(q\)
\(q\)
\(q\)
|+_{\mu_q, 0} \rangle_\mathrm{GKP}
Edge of ideal CV cluster is GKP CZ
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1\hat{q}_2}
|+\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |n\sqrt{\pi}\rangle_q
\hat{C}_Z^\mathrm{CV} \ket{++}_{\mathrm{GKP}} = ?
Substitute definition
|+\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |n\sqrt{\pi}\rangle_q
e^{i \hat{q}_1 \hat{q}_2} \sum_{n_1, n_2} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q = ?
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1\hat{q}_2}
Apply \(\hat q\)
|+\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |n\sqrt{\pi}\rangle_q
e^{i \pi n_1 n_2} \sum_{n_1, n_2} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q = ?
\hat{C}_Z^\mathrm{CV} = e^{i\hat{q}_1\hat{q}_2}
Expand into even and odd sums
e^{i \pi n_1 n_2} \sum_{n_1, n_2} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q = ?
\(n_1\) or \( n_2\) even \(\implies n_1n_2\) is even
\sum_{n_1 \text{ or } n_2 \text{ even }} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q
= |00\rangle_\mathrm{GKP} + |01\rangle_\mathrm{GKP} + |10\rangle_\mathrm{GKP}
e^{i \pi n_1 n_2} \sum_{n_1, n_2} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q = ?
\(n_1\) and \( n_2\) odd \(\implies n_1n_2\) is odd
-\sum_{n_1 \text{ and } n_2 \text{ odd }} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q
= -|11\rangle_\mathrm{GKP}
Edge of ideal CV cluster is logical CZ
= \frac{1}{2}(|00\rangle_\mathrm{GKP} + |01\rangle_\mathrm{GKP} + |10\rangle_\mathrm{GKP} -|11\rangle_\mathrm{GKP})
\hat{C}_Z^\mathrm{CV} \ket{++}_{\mathrm{GKP}}
Logical qubit CZ gate on GKP states!
= \sum_{n_1, n_2}e^{i \pi n_1 n_2} |n_1\sqrt{\pi}\rangle_q |n_2\sqrt{\pi}\rangle_q
\equiv \hat{C}_Z^\mathrm{GKP} \ket{++}_{\mathrm{GKP}}
\hat{C}_Z^\mathrm{CV}
Edge of ideal CV cluster is logical CZ
=
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{GKP}
What about CV CZ on a displaced GKP state?
\hat{C}_Z^\mathrm{CV} \ket{++_{\pmb{\mu}}}_{\mathrm{GKP}} = \,?
Edge of ideal CV cluster is logical CZ
\begin{bmatrix}
\mu_{q_1} \\
\mu_{q_2} \\
\mu_{p_1} + \mu_{q_2} \\
\mu_{p_2} + \mu_{q_1} \\
\end{bmatrix}
\begin{bmatrix}
\mu_{q_1} \\
\mu_{q_2} \\
\mu_{p_1}\\
\mu_{p_2}\\
\end{bmatrix}
\hat{C}_Z^\mathrm{GKP}
=
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^\mathrm{CV} \ket{++_{\pmb{\mu}}}_{\mathrm{GKP}} = \hat{C}_Z^\mathrm{GKP} \ket{++_{\pmb{\mu}'}}_{\mathrm{GKP}}
Edge of ideal CV cluster is logical CZ
\hat{C}_Z^\mathrm{CV} \ket{++_{\pmb{\mu}}}_{\mathrm{GKP}} = \hat{C}_Z^\mathrm{GKP} \ket{++_{\pmb{\mu}'}}_{\mathrm{GKP}}
\hat{C}_Z^\mathrm{GKP}
=
\equiv \hat{C}_Z^\mathrm{GKP} \ket{++}_{\mathrm{GKP}}
\hat{C}_Z^\mathrm{CV}
\begin{bmatrix}
\mu_{q_1} \\
\mu_{q_2} \\
0 + \mu_{q_2} \\
0 + \mu_{q_1} \\
\end{bmatrix}
\begin{bmatrix}
\mu_{q_1} \\
\mu_{q_2} \\
0\\
0\\
\end{bmatrix}
Ideal CV cluster:
\(\mu_p = 0\)
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
Displaced GKP cluster inside a CV cluster
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
= \int \ldots
+
+
Displaced GKP cluster inside a CV cluster
= \int \ldots
q = (2n+L)\sqrt\pi + \mu_q
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
+
+
Homodyne detection collapses the GKP cluster
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
\hat{C}_Z^{\mathrm{GKP}}
Displaced GKP cluster state inside a CV cluster... How to get the entanglement out?
Displaced GKP cluster inside a CV cluster
= \int \ldots
|+_{\mu_q, 0} \rangle_\mathrm{GKP}
\hat{C}_Z^{\mathrm{GKP}}
Interpret the GKP cluster as a qubit cluster.
We perform qubit-qubit quantum teleportation.
Displaced GKP cluster to qubit cluster
\hat{C}_Z^{\mathrm{GKP}} = \text{diag}(1, 1, 1, -1)
Interpret the GKP cluster as a qubit cluster.
We perform qubit-qubit quantum teleportation.
\hat{C}_Z = \text{diag}(1, 1, 1, -1)
\ket+
Displaced GKP cluster to qubit cluster
qubit
qubit
qubit
One bit teleportation
qubit
qubit
qubit
One bit teleportation
qubit
GKP (logical qubit)
qubit
teleportation by products
qubit
qubit
qubit
GKP-qubit one bit teleportation
|0\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |2n\sqrt{\pi}\rangle_q
Gottesman-Kitaev-Preskill (GKP state)
\(q\)
\(|2n\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
GKP-qubit one bit teleportation: X gate
|1\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |(2n+1)\sqrt{\pi}\rangle_q
\(q\)
\(|(2n+1)\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
\(\sqrt \pi\)
\(3\sqrt \pi\)
\(5\sqrt \pi\)
\hat{X}^{\mathrm{GKP}} = e^{-i \sqrt \pi \hat p} = \hat{D}_q(\sqrt{\pi})
GKP-qubit one bit teleportation: X gate
|0\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |2n\sqrt{\pi}\rangle_q
Gottesman-Kitaev-Preskill (GKP state)
\(q\)
\(|2n\sqrt{\pi}\rangle\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
GKP-qubit one bit teleportation: \(\mu_q, \mu_p\)
|1\rangle_\mathrm{GKP} = \sum_{n=-\infty}^{\infty} |(2n+1)\sqrt{\pi}\rangle_q
\(q\)
\(0\)
\(2\sqrt \pi\)
\(4\sqrt \pi\)
\(6\sqrt \pi\)
\(\sqrt \pi\)
\(3\sqrt \pi\)
\(5\sqrt \pi\)
\(|2n\sqrt{\pi}+\mu_q\rangle\)
\(\mu_q\): Rotational X gate
\(\mu_p\): Rotational Z gate
\(\mu_q, \mu_p\) as rotational X, Z
qubit
GKP (logical qubit)
teleportation by products
qubit
qubit
qubit
GKP-qubit one bit teleportation
qubit
GKP (logical qubit)
qubit
qubit
qubit
qubit
GKP-qubit one bit teleportation: X gate
GKP X gate
qubit
GKP (logical qubit)
qubit
qubit
qubit
qubit
GKP-qubit one bit teleportation: \(\mu_p\)
displaced GKP interpreted as rotated qubit
qubit
GKP (logical qubit)
qubit
qubit
qubit
qubit
q = (2n+L)\sqrt\pi + \mu_q
GKP-qubit one bit teleportation: \(\mu_q\)
teleport based on
logical value = {0, 1} of GKP
qubit
GKP (logical qubit)
qubit
q = (2n+L)\sqrt\pi + \mu_q
Homodyne detection roles:
1. Collapsing the superposition into some GKP cluster
2. Quantum teleportation
Entanglement transfer protocol: Recap
1. Initialize all qubits to \(|+\rangle\).
|+\rangle
Entanglement transfer protocol: Recap
|+\rangle
|0\rangle_p
\hat{C}_Z^\mathrm{CV}
2. Create a CV cluster state.
Entanglement transfer protocol: Recap
|+\rangle
|0\rangle_p
Step 3. Apply conditional displacement to each pair:
\(\hat{C}_D = |0\rangle \langle 0| \hat I + |1\rangle \langle 1| \hat{D}_q({\sqrt{\pi}})\)
Entanglement transfer protocol: Recap
|+\rangle
|0\rangle_p
\hat{C}_D
Step 3. Apply conditional displacement to each pair:
\(\hat{C}_D = |0\rangle \langle 0| \hat I + |1\rangle \langle 1| \hat{D}_q({\sqrt{\pi}})\)
Entanglement transfer protocol: Recap
Step 4. Measure the \(q\) quadrature.
|+\rangle
|0\rangle_p
Entanglement transfer protocol: Recap
|+\rangle
Step 5. Correct by products.
Entanglement transfer protocol: Recap
\hat{C}_Z\ket{++}
Entanglement transfer protocol: Loss
1. Ideal CV cluster \(\to\) perfect qubit cluster
2. No reference to GKP states in the protocol
3. Generalizes to arbitrary graph \(G\)
Detector Loss
Channel Loss
Finite squeezing
Entanglement transfer protocol: Loss
Detector Loss
Channel Loss
Finite squeezing
Entanglement transfer protocol: Loss
Detector Loss
Channel Loss
Finite squeezing
Entanglement transfer protocol: Loss
non trivial math ✨
Equivalent circuit model
Squeezed thermal state
loss reduces entanglement transferred
Entanglement transfer protocol: Loss
Finite squeezing:
\(p\)
|0, \sigma_p \rangle_p
Loss: Finite Squeezing
\(q\)
|0, \sigma_p \rangle_p
Finite squeezing:
Loss: Finite Squeezing
\(q\)
\hat{D}_q(\sqrt{\pi})|0, \sigma_p \rangle_p \ket{1}^{\mathrm{Qubit}}
After conditional displacement:
|0, \sigma_p \rangle_p \ket{0}^{\mathrm{Qubit}}
Loss: Finite Squeezing
\(q\)
\hat{D}_q(\sqrt{\pi})|0, \sigma_p \rangle_p \ket{1}^{\mathrm{Qubit}}
Now, the probability of measuring \(q\):
|0, \sigma_p \rangle_p \ket{0}^{\mathrm{Qubit}}
Loss: Finite Squeezing
\(q\)
The displaced GKP state after measuring \(q\):
Loss: Finite Squeezing
\(q\)
The displaced GKP state after measuring \(q\):
Loss: Finite Squeezing
\(q\)
The displaced GKP state after measuring \(q\):
\ket{0} + \, \, \ket 1
Amplitude imbalance error
The qubit is:
Loss: Finite Squeezing
\ket{0} + \, \, \ket 1
Amplitude imbalance error
The qubit is:
We can correct the qubit by performing weak measurement POVMs \(M_0, M_1\).
Failure:
\ket{1}
\ket{0} + \, \, \ket 1
Success:
Loss: Finite Squeezing
\ket{0} + \, \, \ket 1
Amplitude imbalance error
The qubit is:
We can correct the qubit by performing weak measurement POVMs \(M_0, M_1\).
Failure: \(p\)
\ket{1}
\ket{0} + \, \, \ket 1
Success: \(1-p\)
\hat M_0
\hat M_1
Loss: Finite Squeezing
Finitely squeezed CV cluster state
Loss: Finite Squeezing
Loss: Finite Squeezing
Amplitude imbalance error!
After weak measurement:
Loss: Finite Squeezing
Failure: \(p\)
Can convert initial squeezing error to deletion error!
Loss: Finite Squeezing
Loss: Finite Squeezing
Failure: \(p\)
(Stace 2009)
(Barrett and Stace 2010)
Site 1
Site 2
Site 3
Loss: Finite Squeezing
Loss: Finite Squeezing
After weak measurement:
Loss: Finite Squeezing
Dual rail encoding (n=2)
Loss: Finite Squeezing
In order to break entanglement between site 1 and 3 both qubits has to be deleted.
Dual rail encoding (n=2)
Loss: Finite Squeezing
Deletion probability of a site: \(p^n\)
What happens to the qubit if you send in a squeezed thermal state?
\(q\)
\(p\)
\rho^{\mathrm{thermal}}
= \rho
\(q\)
\(p\)
|0, \sigma_p \rangle_p
\hat{D}_p(p_0)
Squeezed thermal state
Loss: Channel and detector loss
What happens to the qubit if you send in a squeezed thermal state?
\(q\)
\(p\)
\rho^{\mathrm{thermal}}
= \rho
\(q\)
\(p\)
|0, \sigma_p \rangle_p
\hat{D}_p(p_0)
Mixture of squeezed states
Squeezed thermal state
=
Loss: Channel and detector loss
What happens to the qubit if you send in a squeezed thermal state?
\(q\)
\(p\)
\rho^{\mathrm{thermal}}
\(q\)
\(p\)
|0, \sigma_p \rangle_p
\hat{D}_p(p_0)
Loss: Channel and detector loss
\rho
What happens to the qubit if you send in a squeezed thermal state?
\(q\)
\(p\)
\rho^{\mathrm{thermal}}
\(q\)
\(p\)
|0, \sigma_p \rangle_p
\hat{D}_p(p_0)
Qubit dephases
Loss: Channel and detector loss
\rho
Suppose \(\hat{C}_D\) is 3 times weaker
Weak conditional displacement
Suppose \(\hat{C}_D\) is 3 times weaker
Weak conditional displacement
Suppose \(\hat{C}_D\) is 3 times weaker
Weak conditional displacement
Weak conditional displacement can be cancelled out by performing entanglement transfer more times.
Suppose \(\hat{C}_D\) is 3 times weaker
Weak conditional displacement
Only one round of weak measurement correction.
Suppose \(\hat{C}_D\) is 3 times weaker
Weak conditional displacement
Possible implementations: Superconducting qubits
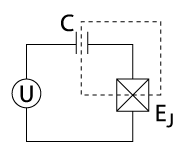
Transmon
CV cluster: Frequency comb in microwave resonator
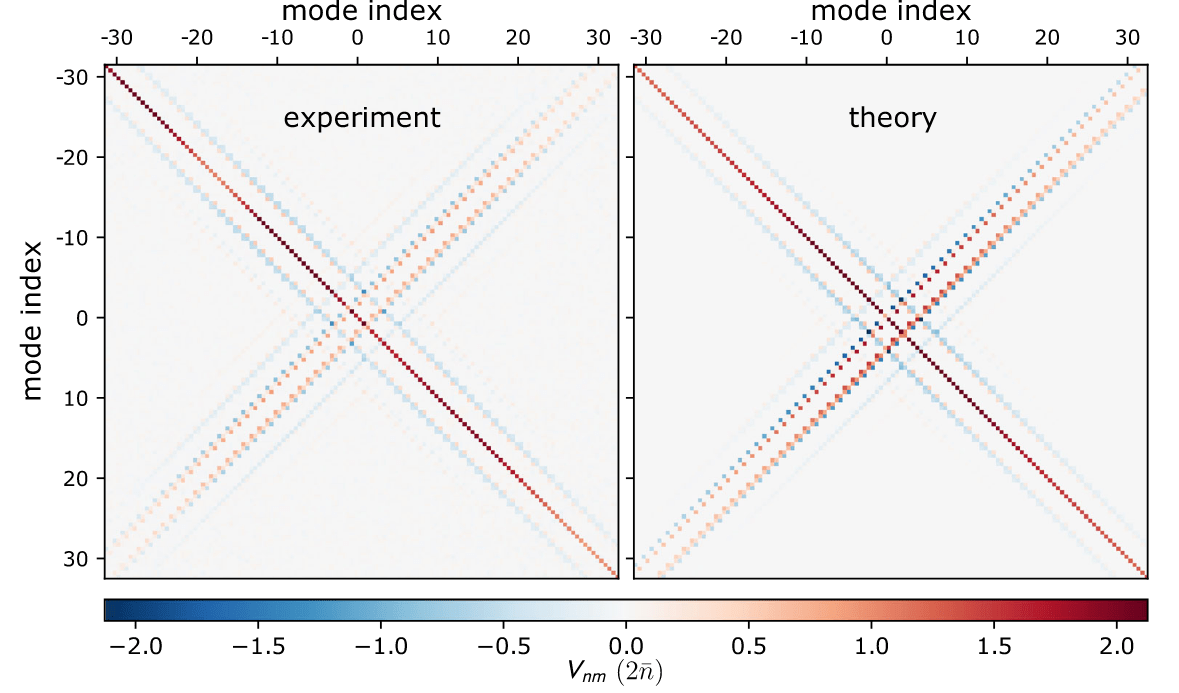
64 correlated modes
(Jolin 2023)
95 correlated modes
(Hernández 2024)
Possible implementations: Superconducting qubits
Transmon
CV cluster: Frequency comb in microwave resonator
Conditional displacement:
ECD gate (A. Eickbusch 2018)
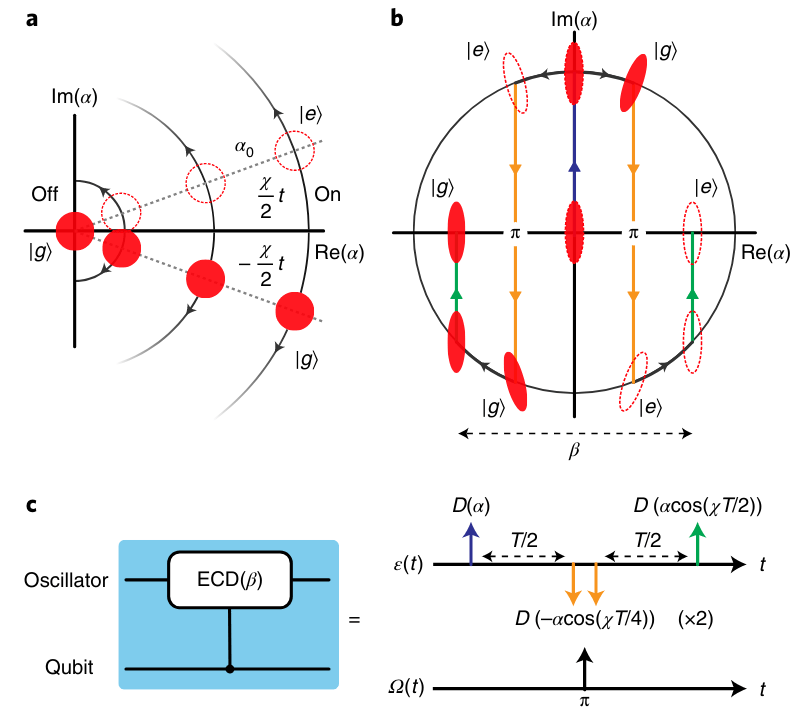
Possible implementations: Superconducting qubits
Transmon
CV cluster: Frequency comb in microwave resonator
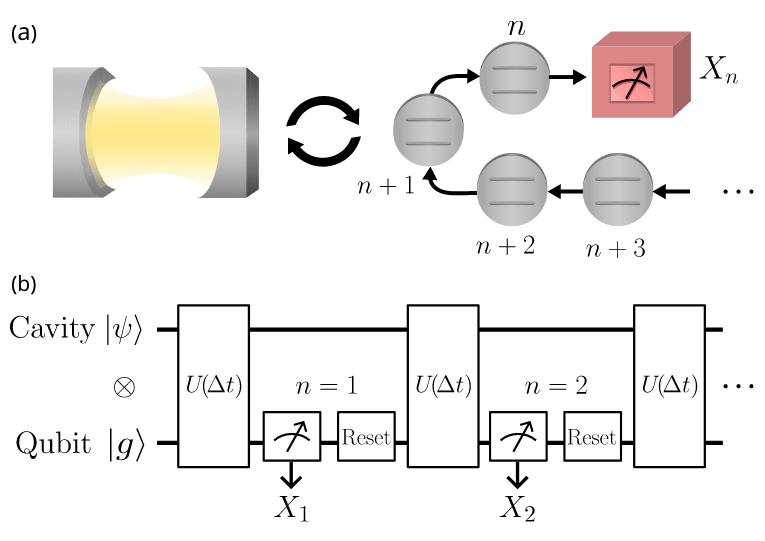
Qubitdyne detection (Strandberg 2023)
Quantum Phase Estimation (Terhal and Weigand 2016)
Possible implementations: Free electron qubits
Free electron qubits
(Reinhardt 2021, Baranes 2024)
CV cluster: Furusawa protocol
Homodyne detection
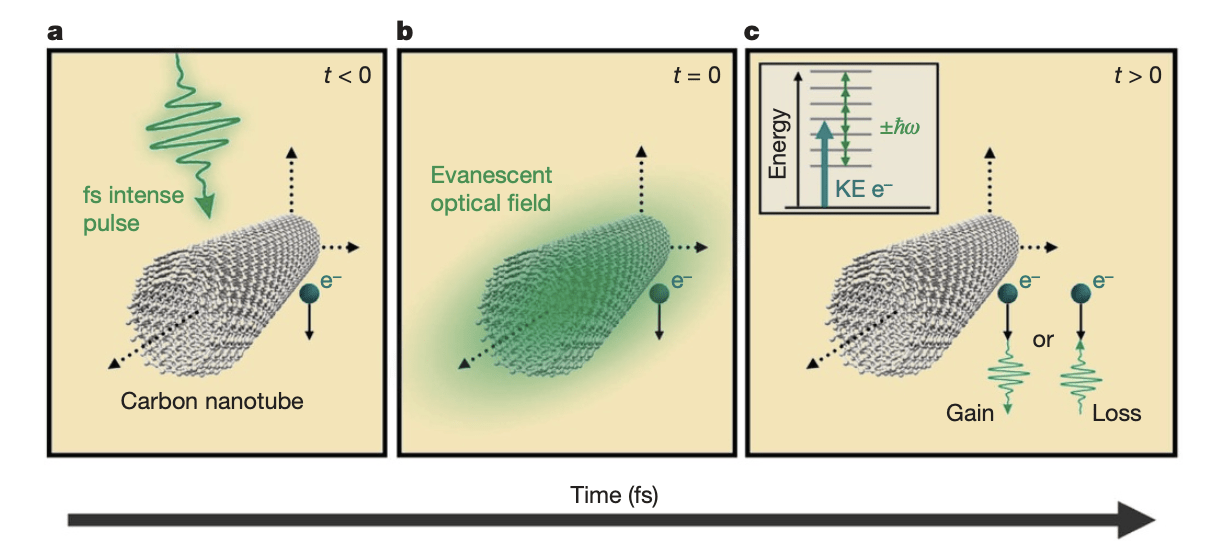
CD gate: Photon-induced near-field electron microscopy (PINEM)
(Barwick 2009)
| Superconducting qubit + microwave cavity | Free electron qubits | |
|---|---|---|
| CV cluster state | Frequency comb in cavity | Optics |
| Conditional displacement | Echoed conditional displacement gate (ECD gate) | PINEM (photon-induced near field electron microscopy) |
| Homodyne detection | Quantum phase estimation Qubitdyne detection |
Homodyne detection |
| Qubit | Transmon | Free electrons |
Possible implementations: Summary
Downloading many-body continuous variable entanglement to qubits
- We can make many body entanglement in qubits!
- Entanglement transfer from CV cluster state to qubit cluster state is possible
- Quality of the qubit cluster state depends on initial CV cluster
- Weak measurement / qubit deletion protocol can reduce requirements
- 6dB squeezing for robust quantum memory
- 12dB squeezing for fault tolerant quantum computing
- No generation of GKP states is needed
- arXiV in progress
- This research was sponsored by the QuantumBC CREATE Program.
Zhihua Han: zhi_han@sfu.ca
References
[1] W. Asavanant et al., Generation of Time-Domain-Multiplexed Two-Dimensional Cluster State, Science 366, 373 (2019).
[2] S. Takeda and A. Furusawa, Toward Large-Scale Fault-Tolerant Universal Photonic Quantum Computing, APL Photonics 4, 060902 (2019).
[3] J. Yoshikawa, S. Yokoyama, T. Kaji, C. Sornphiphatphong, Y. Shiozawa, K. Makino, and A. Furusawa, Invited Article: Generation of One-Million-Mode Continuous-Variable Cluster State by Unlimited Time-Domain Multiplexing, APL Photonics 1, 060801 (2016).
[4] Nicolas C. Menicucci, Peter van Loock, Mile Gu, Christian Weedbrook, Timothy C. Ralph, and Michael A. Nielsen, Universal Quantum Computation with Continuous-Variable Cluster States, Phys. Rev. Lett. 97, 110501 (2006).
[5] Shota Yokoyama et al., Ultra-large-scale continuous-variable cluster states multiplexed in the time domain, Nat. Photonics 7, 5 (2013).
[6] J. Yoshikawa, S. Yokoyama, T. Kaji, C. Sornphiphatphong, Y. Shiozawa, K. Makino, and A. Furusawa, Invited Article: Generation of One-Million-Mode Continuous-Variable Cluster State by Unlimited Time-Domain Multiplexing, APL Photonics 1, 060801 (2016).
[7] T. Monz, P. Schindler, J. T. Barreiro, M. Chwalla, D. Nigg, W. A. Coish, M. Harlander, W. Hänsel, M. Hennrich, and R. Blatt, 14-Qubit Entanglement: Creation and Coherence, Phys. Rev. Lett. 106, 130506 (2011).
[8] C. Song et al., Generation of Multicomponent Atomic Schrödinger Cat States of up to 20 Qubits, Science 365, 574 (2019).
[9] X.-L. Wang et al., Experimental Ten-Photon Entanglement, Phys. Rev. Lett. 117, 210502 (2016).
[10] R. Raussendorf, D. E. Browne, and H. J. Briegel, Measurement-Based Quantum Computation with Cluster States, Phys. Rev. A 68, 022312 (2003).
References
[11] D. Gottesman, A. Kitaev, and J. Preskill, Encoding a Qubit in an Oscillator, Phys. Rev. A 64, 012310 (2001).
[12] J. E. Bourassa et al., Blueprint for a Scalable Photonic Fault-Tolerant Quantum Computer, Quantum 5, 392 (2021).
[13] S. Glancy and E. Knill, Error Analysis for Encoding a Qubit in an Oscillator, Phys. Rev. A 73, 012325 (2006).
[14] A. Botero and B. Reznik, Modewise Entanglement of Gaussian States, Phys. Rev. A 67, 052311 (2003).
[15] C. Weedbrook, S. Pirandola, R. Garcia-Patron, N. J. Cerf, T. C. Ralph, J. H. Shapiro, and S. Lloyd, Gaussian Quantum Information, Rev. Mod. Phys. 84, 621 (2012).
[16] S. L. Braunstein and P. van Loock, Quantum Information with Continuous Variables, Quantum Information with Continuous Variables 77, 65 (2005).
[17] S. Takeda and A. Furusawa, Toward Large-Scale Fault-Tolerant Universal Photonic Quantum Computing, APL Photonics 4, 060902 (2019).
[18] R. Raussendorf, D. E. Browne, and H. J. Briegel, Measurement-Based Quantum Computation with Cluster States, Phys. Rev. A 68, 022312 (2003).
[19] M. V. Larsen, X. Guo, C. R. Breum, J. S. Neergaard-Nielsen, and U. L. Andersen, Deterministic Generation of a Two-Dimensional Cluster State, Science 366, 369 (2019).
[20] B. M. Terhal and D. Weigand, Encoding a Qubit into a Cavity Mode in Circuit QED Using Phase Estimation, Phys. Rev. A 93, 012315 (2016).
References
[21] O. Reinhardt, C. Mechel, M. Lynch, and I. Kaminer, Free-Electron Qubits, Annalen Der Physik 533, 2000254 (2021).
[22] G. Baranes, S. Even-Haim, R. Ruimy, A. Gorlach, R. Dahan, A. A. Diringer, S. Hacohen-Gourgy, and I. Kaminer, Free-Electron Interactions with Photonic GKP States: Universal Control and Quantum Error Correction, Phys. Rev. Res. 5, 043271 (2023).
[23] R. Dahan, G. Baranes, A. Gorlach, R. Ruimy, N. Rivera, and I. Kaminer, Creation of Optical Cat and GKP States Using Shaped Free Electrons, Phys. Rev. X 13, 031001 (2023).
[24] B. Hacker, S. Welte, S. Daiss, A. Shaukat, S. Ritter, L. Li, and G. Rempe, Deterministic Creation of Entangled Atom–Light Schrödinger-Cat States, Nature Photon 13, 110 (2019).
[25] I. Strandberg, A. Eriksson, B. Royer, M. Kervinen, and S. Gasparinetti, Digital Homodyne and Heterodyne Detection for Stationary Bosonic Modes, arXiv:2312.14720.
[26] A. Eickbusch, V. Sivak, A. Z. Ding, S. S. Elder, S. R. Jha, J. Venkatraman, B. Royer, S. M. Girvin, R. J. Schoelkopf, and M. H. Devoret, Fast Universal Control of an Oscillator with Weak Dispersive Coupling to a Qubit, Nat. Phys. 18, 1464 (2022).
[27] B. Wang and L.-M. Duan, Engineering Superpositions of Coherent States in Coherent Optical Pulses through Cavity-Assisted Interaction, Phys. Rev. A 72, 022320 (2005).
[28] S. Kono, K. Koshino, Y. Tabuchi, A. Noguchi, and Y. Nakamura, Quantum Non-Demolition Detection of an Itinerant Microwave Photon, Nature Phys 14, 546 (2018).
[29] J. Hastrup and U. L. Andersen, Protocol for Generating Optical Gottesman-Kitaev-Preskill States with Cavity QED, Phys. Rev. Lett. 128, 170503 (2022).
[30] A. Reiserer, S. Ritter, and G. Rempe, Nondestructive Detection of an Optical Photon, Science 342, 1349 (2013).
[31] J. C. R. Hernández, F. Lingua, S. W. Jolin, and D. B. Haviland, Control of Multi-Modal Scattering in a Microwave Frequency Comb, arXiv:2402.09068.
[32] S. W. Jolin, G. Andersson, J. C. R. Hernández, I. Strandberg, F. Quijandría, J. Aumentado, R. Borgani, M. O. Tholén, and D. B. Haviland, Multipartite Entanglement in a Microwave Frequency Comb, Phys. Rev. Lett. 130, 120601 (2023).
etp
By Zhi Han
