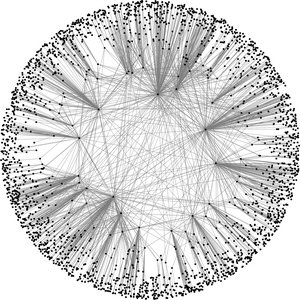
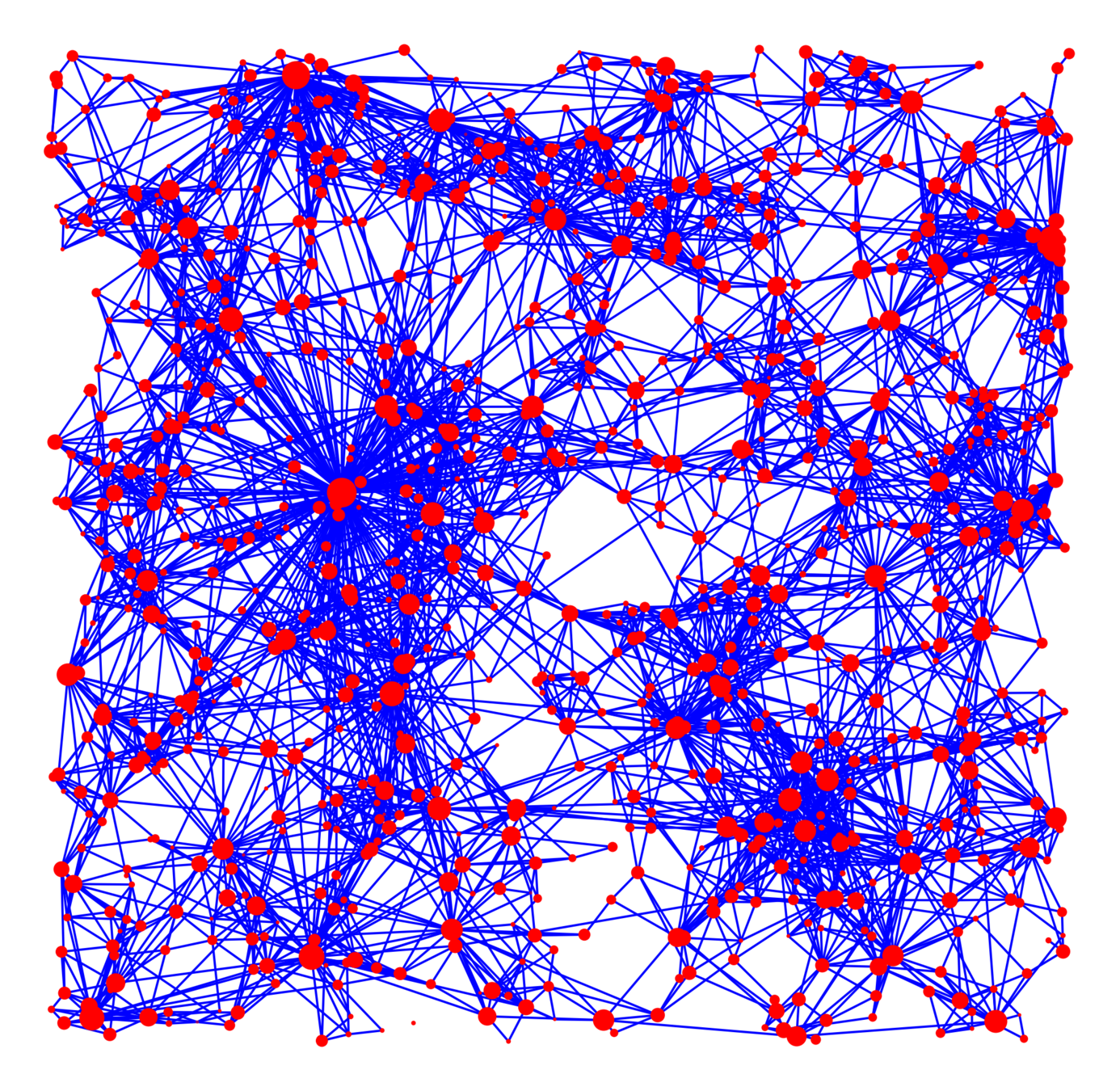
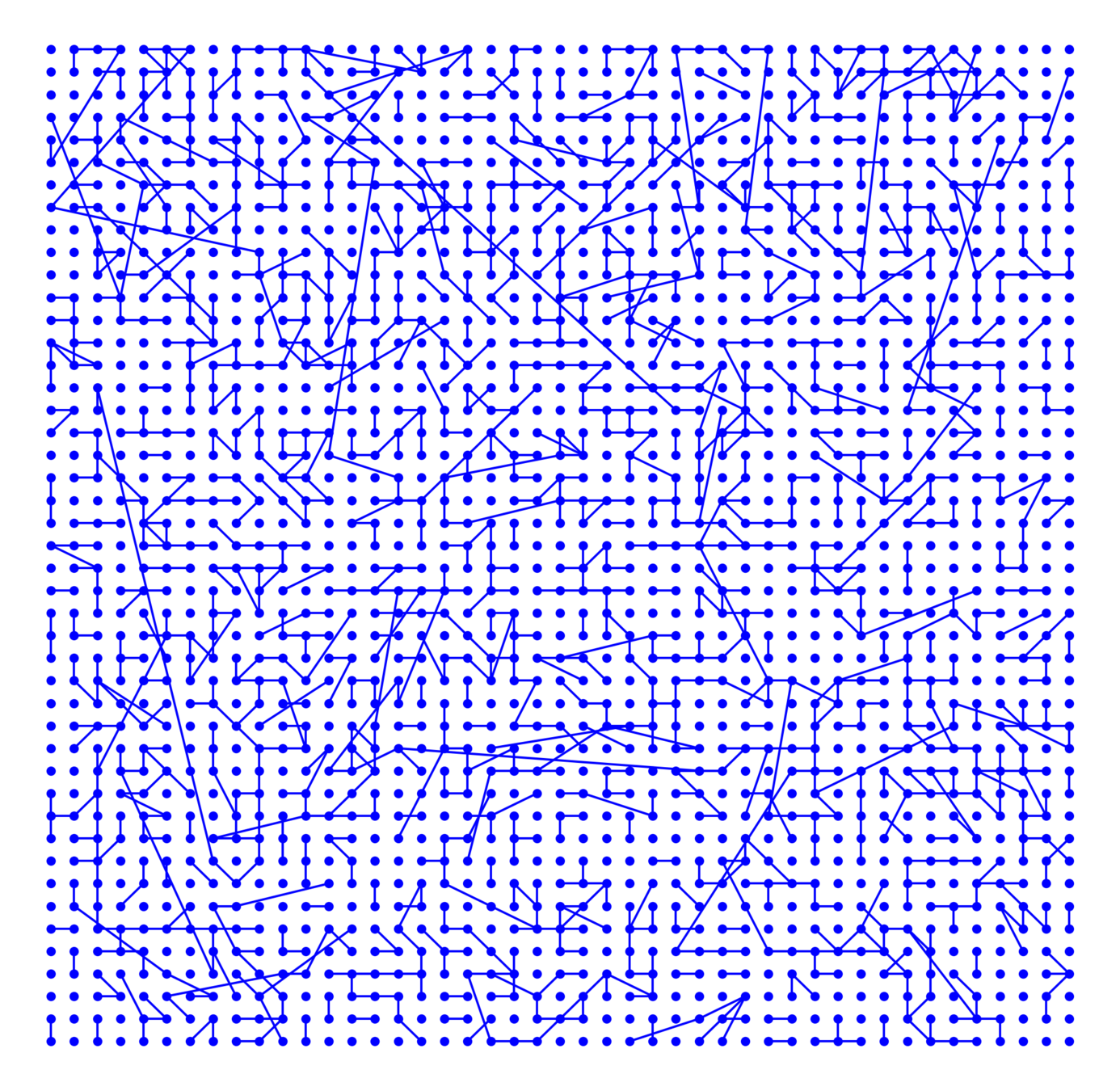
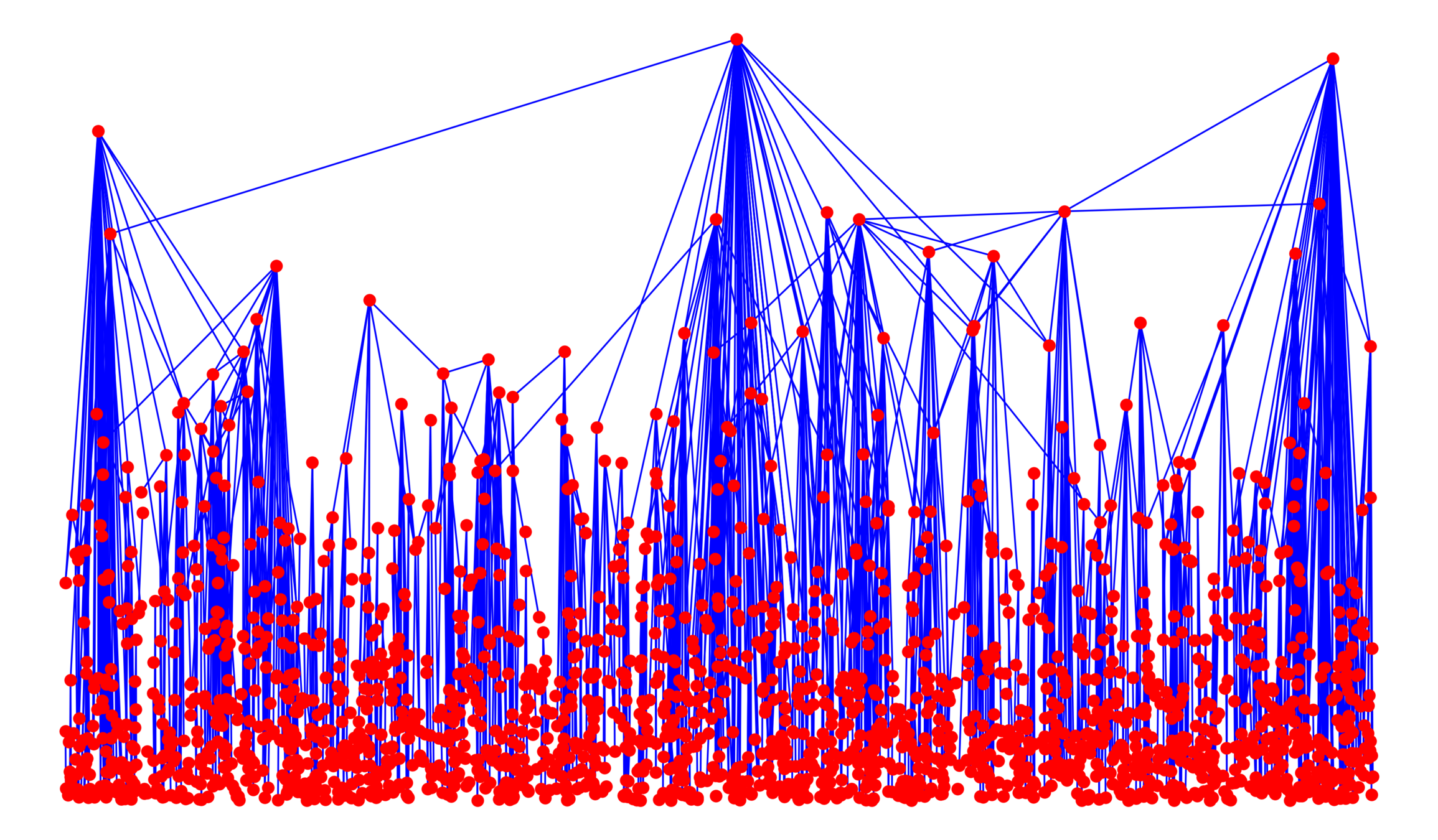
Hyperbolic random graph
Scale-free percolation
Long-range percolation
Age-dependent RCM
Random geom. graph
Nearest-neighbor percolation
Kernel-based spatial random graphs
Four parameters to interpolate/switch
Vertex set
- Spatial locations,
- i.i.d. (power-law) weights
Edge more likely if
- Spatially nearby,
- High weight

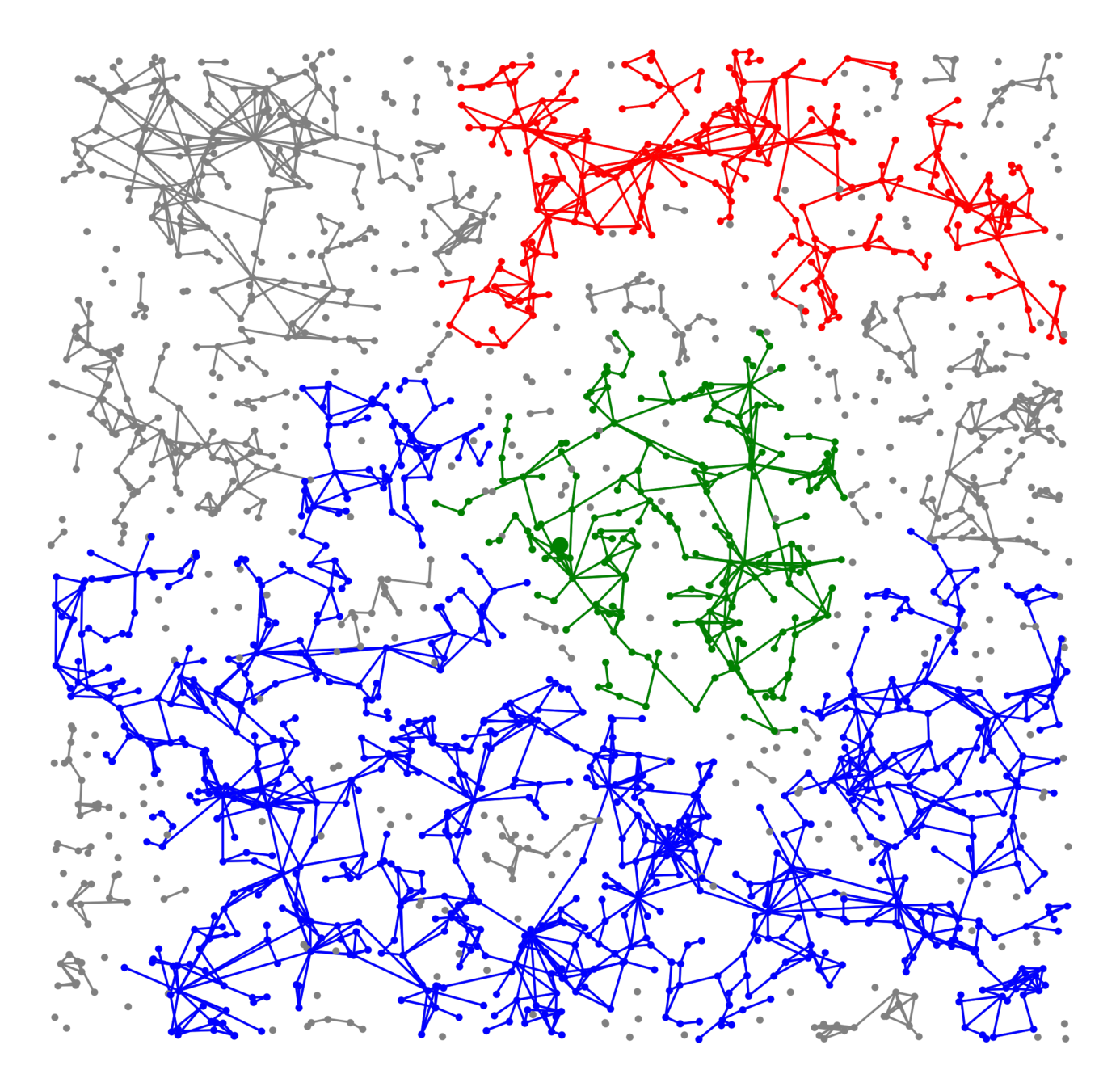
Component sizes: questions and results
- Largest component \(\mathcal{C}_n^{(1)}\):
- Cluster-size decay:
- Second-largest component:
Questions (finite, supercritical graph)





\mathbb{P}\Big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\Big)=
\frac{|\mathcal{C}_n^{(1)}|}{n}\overset{\mathbb{P}}\longrightarrow

\mathbb{P}\Big(|\mathcal{C}_n(0)|\ge k, 0\notin\mathcal{C}_n^{(1)}\Big)=
\mathbb{P}\big(\mathcal{C}(0)=\infty\big)
\exp\big(-\Theta(n^\zeta)\big)
\exp\big(-\Theta(k^\zeta)\big)
|\mathcal{C}_n^{(2)}|=\Theta\big(\phantom{(\log n)^{1/\zeta}}\big)
|\mathcal{C}_n^{(2)}|=\Theta\big((\log n)^{1/\zeta}\big)
Cool stuff, because
- Largest component \(\mathcal{C}_n^{(1)}\):
- Cluster-size decay:
- Second-largest component:
Questions (finite, supercritical graph)




{\color{lightgray}\mathbb{P}\Big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\Big)=}
{\color{lightgray}\frac{|\mathcal{C}_n^{(1)}|}{n}\overset{\mathbb{P}}\longrightarrow}
{\color{lightgray}\mathbb{P}\Big(|\mathcal{C}_n(0)|\ge k, 0\notin\mathcal{C}_n^{(1)}\Big)=}
{\color{lightgray}\mathbb{P}\big(\mathcal{C}(0)=\infty\big)}
\exp\big(-\Theta(n^\zeta)\big)
\exp\big(-\Theta(k^\zeta)\big)
{\color{lightgray}|\mathcal{C}_n^{(2)}|=}\,\, \Theta\big((\log n)^{1/\zeta}\big)
(\(\ge\))3 related quantities,
any value \(\zeta\in(0,1)\).
structural information,



Scale-free percolation
Long-range percolation
Age-dependent RCM
Current status*
Paper I
Paper II
Paper III
(Remaining) Upper bounds,
*all papers jointly with Júlia Komjáthy and Dieter Mitsche
Copy of Cluster-size decay
By joostjor
Copy of Cluster-size decay
- 300



